Fractions to Percentages: Ultimate Conversion Worksheet

Understanding the conversion from fractions to percentages is an essential skill in many academic and professional fields. Whether you're calculating discounts while shopping, analyzing financial data, or solving mathematical problems, converting fractions to percentages helps in quick and effective comparison and interpretation of data. This post will walk you through the systematic process of converting fractions to percentages, offer practical examples, and provide tips to make this conversion as easy as pie.
Why Convert Fractions to Percentages?

The conversion of fractions to percentages offers a standardized way to understand ratios, proportions, and rates. Here are several scenarios where this conversion is beneficial:
- Comparing discounts: Retail offers are often advertised as percentages. Knowing how to convert a fraction discount into a percentage can help you better understand the deal.
- Grades in school: Teachers might grade assignments out of total points, and converting these scores to percentages gives a clearer picture of performance.
- Financial analysis: Percentages are standard in financial statements, allowing for easy comparison and analysis of financial health over time.
Basic Conversion Steps

Here are the steps to convert any fraction to a percentage:
- Convert the fraction to a decimal: Divide the numerator by the denominator.
- Multiply by 100: This step converts the decimal into a percentage.
- Add the percentage symbol: Don’t forget the ‘%’ symbol at the end.
Examples

Let’s take a few examples to illustrate the process:
- Example 1: Convert 3⁄4 to a percentage.
- 3 ÷ 4 = 0.75
- 0.75 * 100 = 75
- So, 3⁄4 = 75%
- Example 2: Convert 1⁄2 to a percentage.
- 1 ÷ 2 = 0.5
- 0.5 * 100 = 50
- So, 1⁄2 = 50%
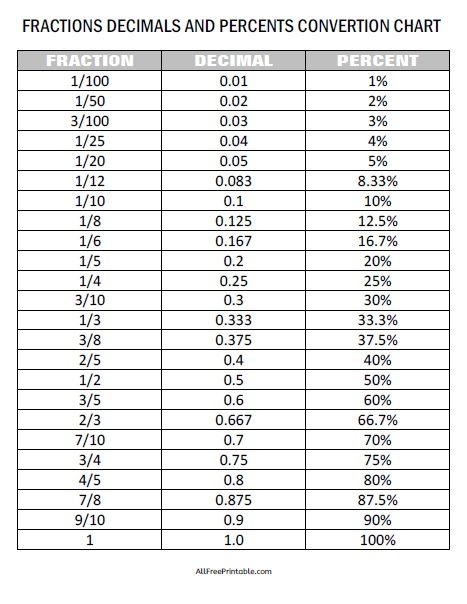
| Fraction | Decimal | Percentage |
|---|---|---|
| 3/4 | 0.75 | 75% |
| 1/2 | 0.5 | 50% |
| 5/8 | 0.625 | 62.5% |

💡 Note: When dealing with recurring decimals, you might want to round to the nearest hundredth to simplify the conversion to percentages.
Practical Applications

Converting fractions to percentages isn’t just an academic exercise; here are some practical applications:
- Budgeting: When planning your monthly expenses, converting savings to a percentage of your income helps in better financial planning.
- School: Students often need to convert their scores or grades into percentages to understand their performance better.
- Data Analysis: In fields like market research or psychology, converting ratios to percentages for charts and graphs simplifies presentation and analysis of data.
Tips for Quick Conversions

Here are some shortcuts to make conversions quicker:
- Common Fractions: Memorize the percentages for common fractions like 1⁄2 (50%), 1⁄4 (25%), and 3⁄4 (75%).
- Multiplication: Instead of division, sometimes multiplying by the reciprocal is easier. For example, 4⁄5 can be quickly converted by multiplying by 20 (since 5⁄4 = 1.25 and 1.25 * 100 = 125%).
- Mental Math: Use mental shortcuts, like understanding that any fraction less than 1⁄2 will be less than 50%.
🌟 Note: For complex fractions or when precision matters, stick to the step-by-step method to avoid mistakes.
Converting fractions to percentages is a fundamental skill that's useful in various daily activities. By understanding and practicing these conversions, you'll improve your numerical literacy and enhance your ability to make quick, informed decisions in both personal and professional settings. Remember, the more you practice, the more intuitive these conversions will become, enabling you to navigate through numerical data with ease.
How can I check my fraction to percentage conversion?
+
You can verify your work by converting the percentage back into a fraction and seeing if it matches the original.
Are there any online tools for converting fractions to percentages?

+
Yes, numerous websites and mobile applications offer calculators that can convert fractions to percentages instantly.
Why is converting fractions to percentages important in real life?
+
Conversions like these are used in finance, shopping, cooking (e.g., scaling recipes), and many other daily activities where understanding proportions is crucial.



