5 Essential Tips for Mastering Fraction Operations Worksheet

Mastering fraction operations is an essential skill that underpins a wide array of mathematical applications, from basic arithmetic to advanced calculus. Whether you're a student struggling with the complexities of fractions or an adult looking to brush up on your math skills, understanding and applying these operations effectively can significantly enhance your math proficiency. This detailed guide presents five essential tips to help you master fraction operations using worksheets, ensuring you can confidently navigate the world of fractions.
Understand the Basics

Before delving into the specifics of operations, it’s crucial to have a solid grasp on what fractions are and their fundamental properties:
- Definition: A fraction represents a part of a whole, where the top number (numerator) signifies the part, and the bottom number (denominator) signifies the total parts making up the whole.
- Types of Fractions: Learn to distinguish between proper fractions, improper fractions, and mixed numbers.
- Simplifying Fractions: Reducing fractions to their simplest form is key to solving complex problems efficiently.

Adding and Subtracting Fractions
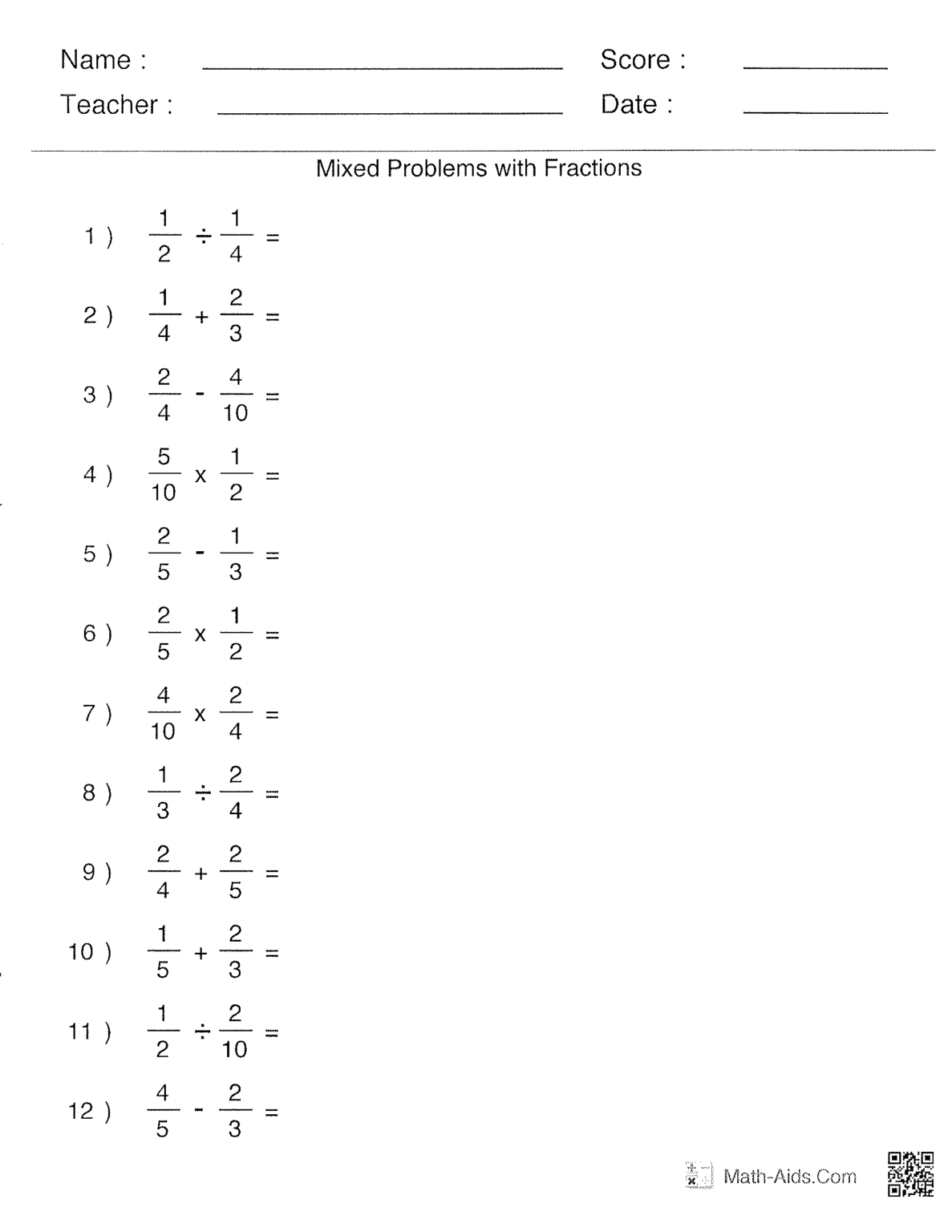
Adding and subtracting fractions involves:
- Finding a Common Denominator: Identify or create a common denominator to facilitate operations.
- Converting Mixed Numbers: If mixed numbers are involved, convert them into improper fractions for easier manipulation.
- Handling Different Denominators: Use cross-multiplication or other methods to convert to a common denominator.
| Fraction 1 | Fraction 2 | Operation | Result |
|---|---|---|---|
| 1⁄2 | 1⁄4 | Addition | 3⁄4 |
| 3⁄4 | 1⁄3 | Subtraction | 5⁄12 |

📝 Note: Cross-multiplication can be particularly useful when subtracting or adding fractions with different denominators to quickly find a common ground.
Multiplying and Dividing Fractions

Multiplication and division of fractions are straightforward once you know the rules:
- Multiplication: Simply multiply the numerators together and the denominators together, then simplify.
- Division: Invert the second fraction (divisor) and multiply. Simplify where possible.

Use Visual Aids

Visual aids like pie charts, number lines, and fraction strips can make fractions more tangible:
- Visualize each operation for better understanding.
- Use them to check your work or understand why a process works the way it does.
- They’re particularly helpful when dealing with mixed numbers or complex fractions.

Practice Consistently

Worksheet practice is invaluable:
- Diverse Problems: Work through problems that cover all operations and fraction types.
- Timed Challenges: Increase your speed and accuracy under timed conditions.
- Review Mistakes: Analyze errors to identify common pitfalls and improve.
- Mixed Operations: Practice sets that require you to perform multiple operations within the same problem.
✏️ Note: Keep practicing regularly, even if it’s just a few problems each day, to maintain and grow your skills in fraction operations.
Throughout this journey, you've explored various facets of fraction operations, from understanding the basics to mastering complex calculations. These five tips will help you solidify your foundation in fractions, making operations with fractions second nature. The consistent application of these strategies, coupled with the discipline of practice, can lead to remarkable improvement in your mathematical abilities, opening doors to deeper mathematical exploration and success in various academic and real-world scenarios.
Why is simplifying fractions important before performing operations?

+
Simplifying fractions before performing operations can make the calculations easier to manage and understand. It also helps in getting the final answer in its simplest form, reducing the chance of errors.
What if the fractions have very large or different denominators?

+
Use techniques like the Least Common Multiple (LCM) to find a common denominator that is manageable or apply cross-multiplication to make operations with different denominators more accessible.
Can visual aids really help with fraction operations?

+
Absolutely. Visual aids provide a concrete representation of abstract concepts, which can lead to a better understanding of how fractions behave during operations.
How often should one practice to improve fraction operations?
+
Consistent practice, even if it’s just for a short duration daily, can significantly enhance your skills. Aim for daily practice to keep your understanding sharp.



