Surface Area Nets Worksheet Answer Key: 5 Quick Tips

Whether you're a student struggling to master the concept of surface areas or a teacher in search of the most effective way to teach it, understanding the intricacies of surface area nets worksheets can significantly enhance your grasp or instructional delivery of this mathematical concept. The following are 5 quick tips to help you conquer or teach surface area nets with confidence:
1. Understanding the Basics
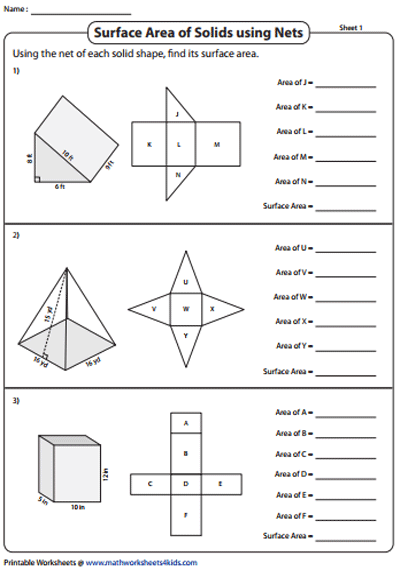
The journey to mastering surface areas begins with understanding what a net is. A net is a flat representation of a 3D shape when it is “unfolded”. Here’s how to approach it:
- Recognize the 3D Shape: Before folding, identify the shape you’re dealing with. Is it a cube, pyramid, or a more complex figure like a hexagonal prism?
- Visualize the Shape: Try to visualize how the net, when folded, forms the 3D shape. This can be done by mentally folding or using virtual 3D models to aid comprehension.

2. Properly Identifying Surface Areas

After understanding the concept of a net, the next step is to accurately calculate the surface area:
- Area Calculation: Calculate the area of each face of the net and sum them up. Ensure you’re using the correct dimensions for each face.
- Include All Faces: Make sure you’re not missing any hidden faces when unfolding the shape. Every surface counts.
3. Practice with Varied Shapes

To ensure a thorough understanding, practice with different shapes. Here are some ideas:
- Cube: Start with simple cubes, then move to cuboids for variations in dimensions.
- Pyramids and Cones: Understand the net of these shapes and how different slants affect the area.
- Complex Polyhedrons: For advanced learners, try prisms and polyhedrons with more surfaces.
4. Utilizing Online Resources and Tools
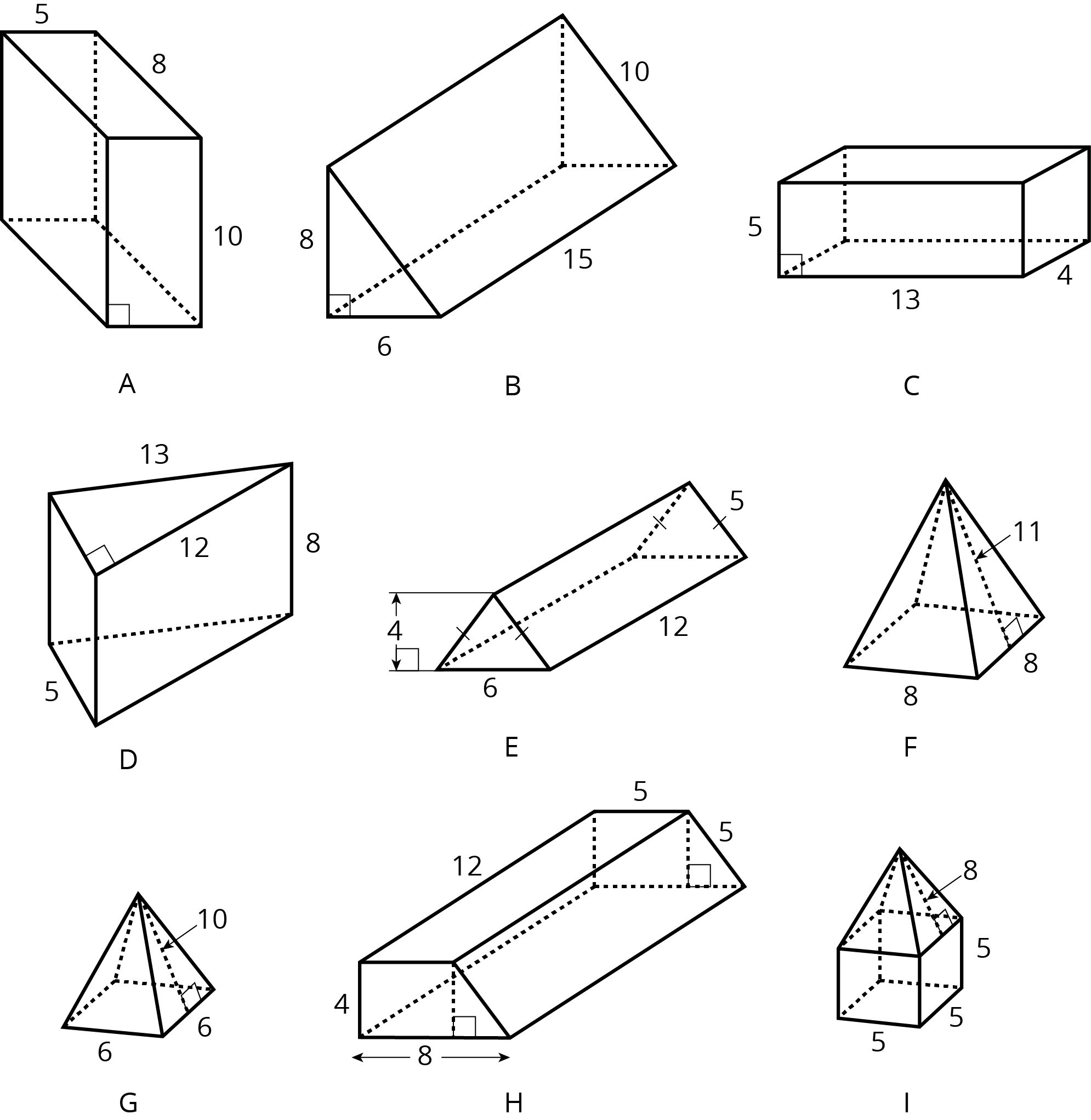
Technology can be your ally in mastering surface areas:
- Virtual Manipulatives: Use online tools where students can interact with and manipulate shapes, observing their transformation into nets.
- Worksheet Generators: Websites offer customizable worksheets, allowing for targeted practice based on the complexity of the shapes.
🔍 Note: Ensure the online resources you use are reliable and align with your curriculum standards to avoid teaching incorrect methods or concepts.
5. Revise and Review with Techniques

Here are some effective revision techniques:
- Flashcards: Create flashcards with a 3D shape on one side and its net on the other. This aids in quick recognition.
- Group Activities: Engage in activities where students discuss how to unfold shapes or calculate their surface areas collaboratively.
- Practice Quizzes: Regularly test yourself or your students with quick quizzes on surface area calculations.
In the journey to master or teach the concept of surface areas, remember that patience, practice, and a structured approach are key. By breaking down the 3D shapes into their 2D representations, visualizing, calculating, and reinforcing these skills through varied practice, you or your students will develop a solid understanding of surface area nets. This comprehensive approach not only enhances mathematical skills but also encourages spatial reasoning and problem-solving abilities, which are essential for future STEM endeavors.
What is the purpose of a net in mathematics?
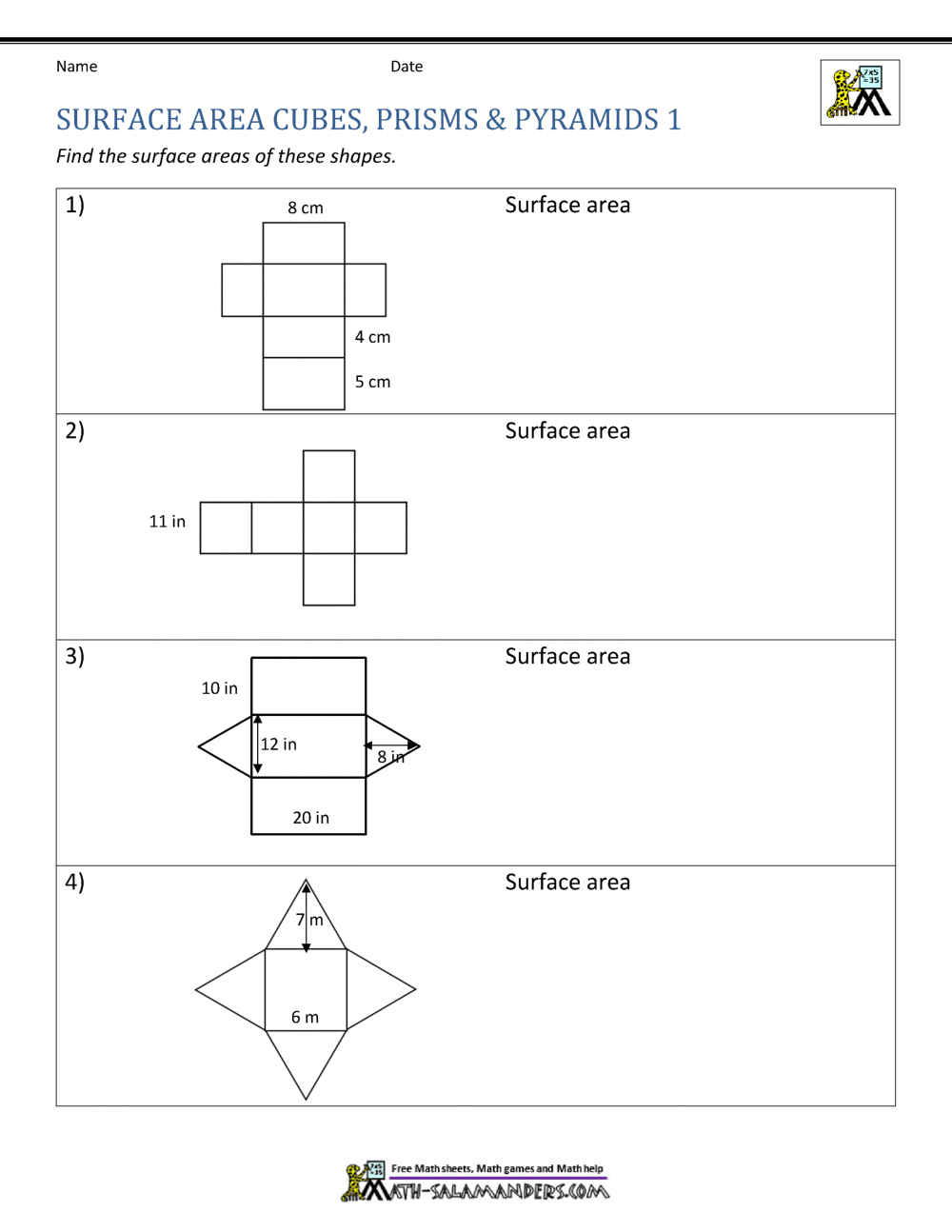
+
A net provides a 2D visualization of a 3D object, allowing for easier calculation of surface areas, understanding of shape structures, and preparation for folding into the original 3D shape.
How do you calculate the surface area using a net?

+
Calculate the area of each face of the net separately, using appropriate formulas based on their shapes (e.g., A=length * width for rectangles). Sum all these areas to get the total surface area of the 3D shape.
Why is it important to practice with different shapes?
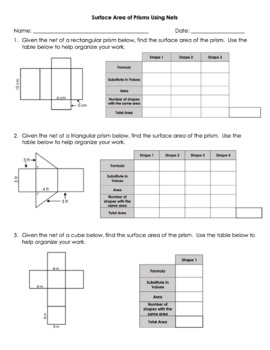
+
Practicing with various shapes helps in understanding the different ways a shape can be ‘unfolded’, recognizing the versatility of surface area calculations, and strengthening spatial visualization skills.
Can you use technology to learn surface areas?

+
Absolutely! There are many online resources, from interactive manipulatives to educational videos, that can aid in visualizing and calculating surface areas effectively.