5 Tips for Finding Slope: Rise Over Run Worksheet

When learning algebra, one of the foundational concepts students grapple with is understanding slope. Slope is essentially how steep a line is, and it's pivotal for graph interpretation, functions, and real-world applications like understanding gradients or determining the rate of change. In this comprehensive guide, we'll walk through five tips to simplify finding slope using the Rise Over Run method, complete with worksheet examples to solidify your understanding.
What is Slope?

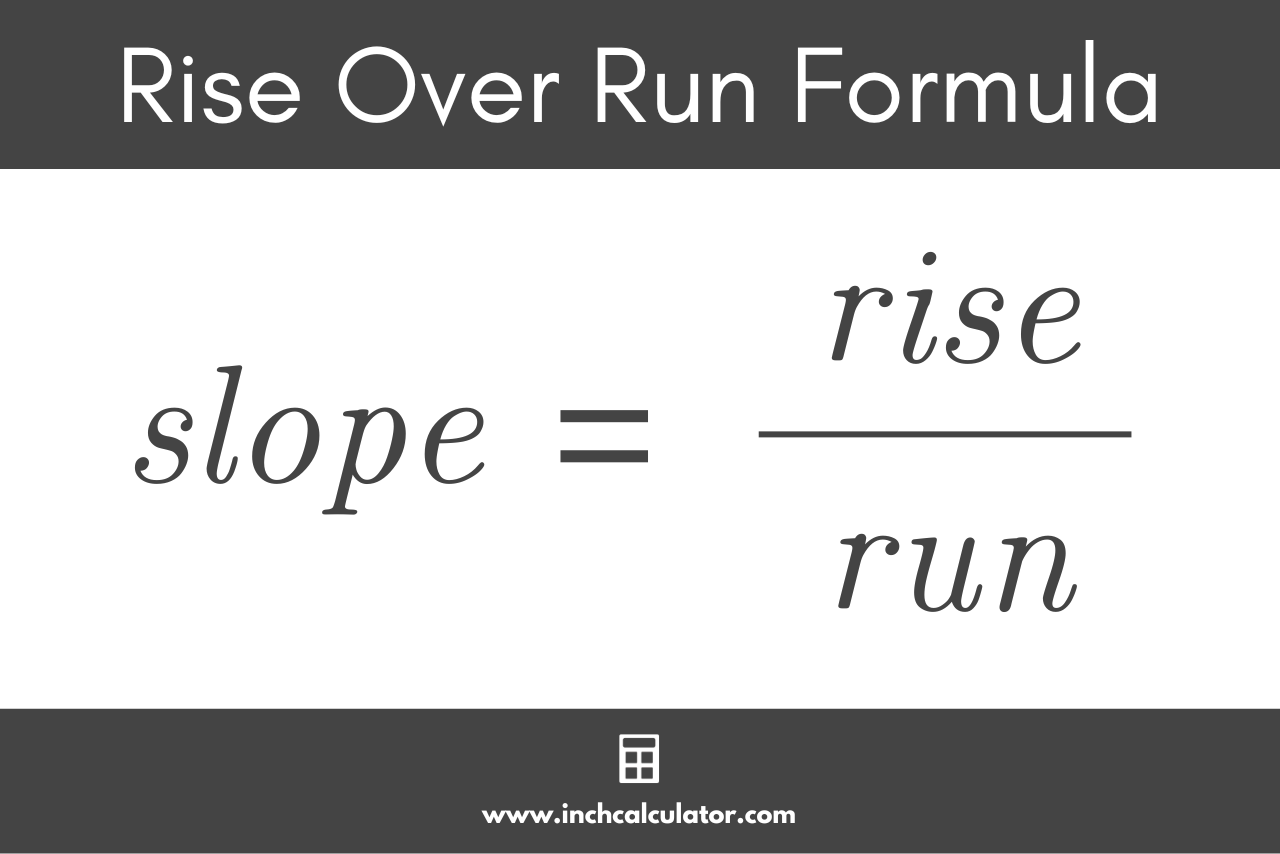
Slope, denoted as m, is the measure of the steepness of a line in a coordinate system. It’s calculated using the formula:
m = (Change in y) / (Change in x)
This formula breaks down as:
- Change in y: How much the y-coordinate changes from one point to another, also known as the rise.
- Change in x: How much the x-coordinate changes from one point to another, also known as the run.
Here’s a practical example for better understanding:
| Point 1 | Point 2 | Rise | Run | Slope (m) |
|---|---|---|---|---|
| (2, 3) | (6, 7) | 4 | 4 | 1 |

As you can see, if the rise equals the run, the slope will be 1, indicating a line that rises one unit for every unit run to the right.
Tip 1: Identify Two Points Clearly

To calculate slope, you must have at least two points on the line. Selecting these points should be done carefully:
- Choose Points with Whole Numbers: Initially, avoid points with fractions or decimals to reduce calculation errors.
- Identify Points Visually: When looking at a graph, pick points that are easily distinguishable.
Here’s a worksheet example:

Tip 2: Understand the Direction
The sign of the slope indicates the line’s direction:
- Positive Slope: The line moves upward from left to right (rise is positive).
- Negative Slope: The line moves downward from left to right (rise is negative).
- Zero Slope: The line is horizontal (no rise).
- Undefined Slope: The line is vertical (no run).
📘 Note: Remember, the direction of the line does not change the calculation of slope; it only determines the sign of the result.
Tip 3: Use a Slope Triangle

Drawing a right-angled triangle on the graph with the line as its hypotenuse can help visualize the slope:
- Draw a horizontal line from one point to another along the x-axis.
- Draw a vertical line from that point up or down to the y-axis, forming the triangle.
- The vertical leg of the triangle represents the rise, and the horizontal leg represents the run.

Tip 4: Fraction Simplification

If your calculated slope results in a fraction, simplify it. Simplifying makes it easier to compare slopes:
- Find the greatest common divisor (GCD) of the numerator (rise) and the denominator (run).
- Divide both by this GCD to simplify the fraction.
For example, if your slope is 6⁄4, simplify it to 3⁄2.
Tip 5: Practice with Real-life Scenarios

To solidify your understanding of slope, try applying it to real-life scenarios:
- Speed: The slope of a distance vs. time graph represents speed.
- Ramp Gradient: Understanding how steep a ramp is for wheelchair access or skateboarding.
- Stock Market: Plotting stock prices over time to see if there’s a trend in growth or decline.
This practical application helps connect algebraic theory to everyday life, making the concept more relatable and understandable.
To wrap up, calculating slope using the rise over run method might seem daunting at first, but with these five tips and regular practice, you'll find it becomes second nature. Remember to identify points clearly, understand the directional impact on slope, use visual aids like slope triangles, simplify your calculations, and relate them to real-world applications. The essence of understanding slope is not just in memorizing formulas but in truly comprehending its implications in various contexts, which ultimately enhances your overall grasp of algebra and beyond.
What does a slope of 0 mean?

+
A slope of 0 means that the line is horizontal and there is no rise in y for any change in x. Essentially, it’s a straight, flat line parallel to the x-axis.
How do you calculate the slope if the line is vertical?

+
A vertical line has an undefined slope because the run (change in x) is zero, and you can’t divide by zero. This means there’s an infinite rise for any given horizontal change.
Why is understanding slope important?

+
Understanding slope is crucial because it represents the rate of change, which is fundamental in fields like economics, engineering, physics, and even daily life scenarios like understanding the steepness of a hill or road.