5 Easy Tips for Finding Slope From Two Points

Understanding how to calculate the slope between two points is fundamental in mathematics, particularly in algebra and geometry. The slope is a measure of steepness or the rate at which the line changes, which can be applied in various real-world scenarios, from physics to financial modeling. Here are five straightforward tips to help you master this concept.
1. Recall the Slope Formula

The slope between two points, let’s say (x1, y1) and (x2, y2), is calculated using the formula:
m = (y2 - y1) / (x2 - x1)
This equation represents the change in y divided by the change in x. Before diving into calculations:
- Identify the coordinates of the two points.
- Be sure to keep the y-coordinates consistent in the numerator and x-coordinates in the denominator.
💡 Note: Ensure you are consistent with the order of subtraction in both the numerator and the denominator to avoid errors.
2. Use Order to Avoid Mistakes

Consistency in the order of subtraction is key to prevent common mistakes:
- If you subtract y2 from y1 in the numerator, you must subtract x2 from x1 in the denominator.
- Make sure you do not swap x-coordinates with y-coordinates.
Here’s a simple table to help remember the order:
| What to Subtract | Numerator (y-values) | Denominator (x-values) |
|---|---|---|
| First Value | y1 | x1 |
| Second Value | y2 | x2 |

3. Apply the Formula

Let’s put the slope formula into practice with an example:
- Given points (2,3) and (5,9):
- Using our formula: m = (9 - 3) / (5 - 2) = 6 / 3 = 2
This example demonstrates a positive slope since the line rises as it moves to the right.
📌 Note: If the result is negative, the slope is downward from left to right; if it's zero, the line is horizontal; if undefined, the line is vertical.
4. Check for Special Cases
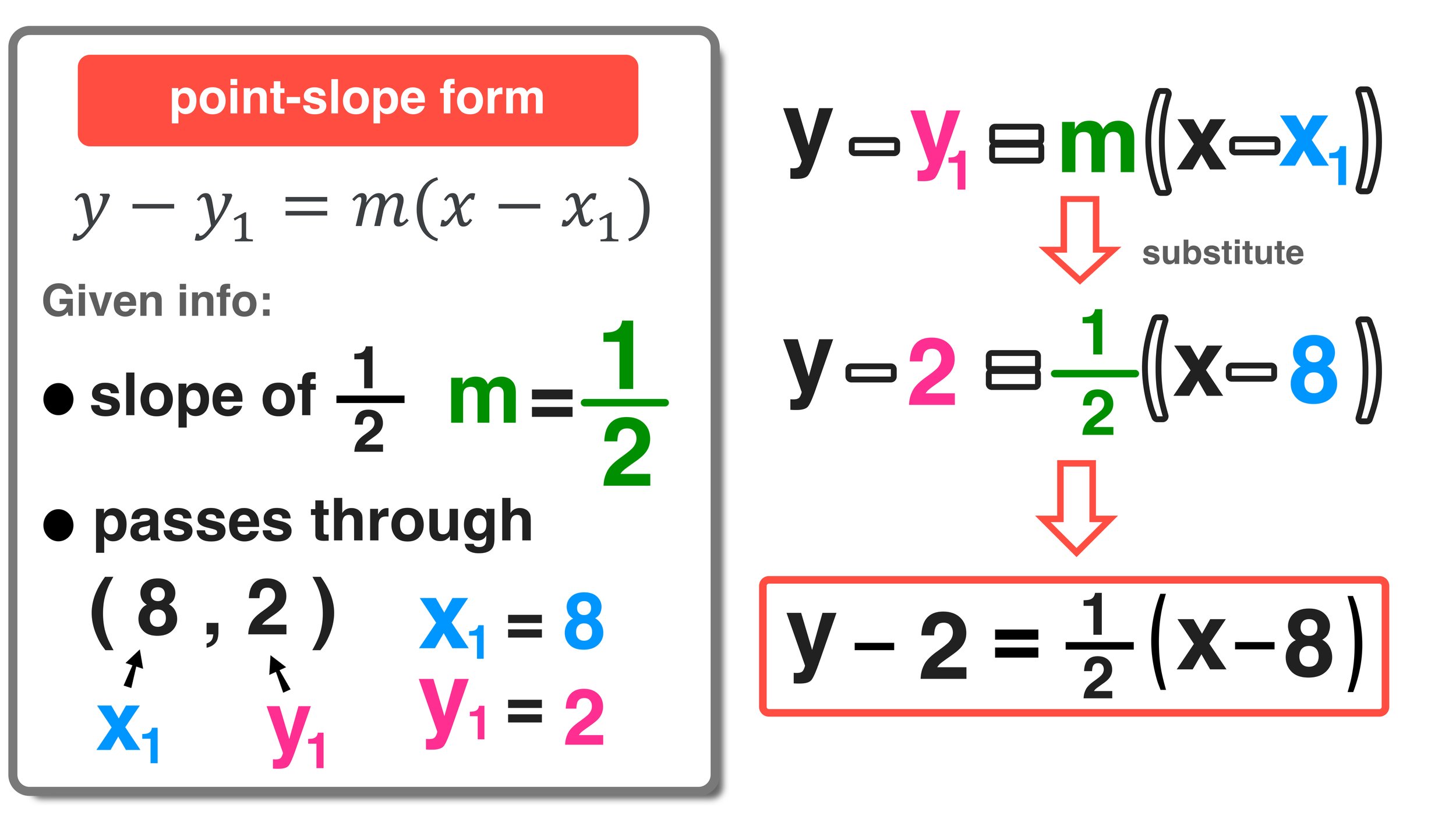
Be aware of special scenarios where the slope calculation might not yield a typical result:
- Zero Slope: When the change in x is zero (x2 = x1), the line is horizontal, and the slope is zero.
- Undefined Slope: When the change in y is zero (y2 = y1), the line is vertical, and the slope is undefined.
Understanding these special cases will help you interpret and avoid common mistakes in slope calculations.
5. Practice with Real-World Applications

To solidify your understanding, apply the slope concept to practical situations:
- Financial Models: Use slope to analyze the growth rate of investments or the decline in value over time.
- Physics: The slope of a velocity-time graph represents acceleration.
- Geography: Calculate the steepness of mountain slopes for hiking or engineering projects.
By practicing with real-life scenarios, you’ll not only understand the calculations better but also appreciate the importance of slope in various fields.
In wrapping up this discussion on finding slope from two points, remember that mastering these tips can significantly enhance your mathematical proficiency. From understanding the basics of the slope formula to recognizing special cases and applying it in real-world contexts, the journey towards better slope calculation is both practical and enlightening. Keep in mind the importance of consistency in subtraction, and remember that slope is not just a number; it's a story of how things change over space or time.
Why do we need the slope?

+
Slope is essential in various mathematical and scientific fields to describe the rate of change, angle of incline, or the direction of a line. It’s used in graphing, calculating rates of change in economics or physics, and understanding angles in trigonometry or geography.
What does a positive slope mean?

+
A positive slope means that as you move from left to right on the graph, the y-values increase. The line rises, indicating an increasing function or an upward trend.
What if the slope is negative?

+
A negative slope means that as you move from left to right on the graph, the y-values decrease. The line descends, indicating a decreasing function or a downward trend.
How do you handle an undefined slope?

+
An undefined slope occurs when the denominator (x2 - x1) of the slope formula is zero, meaning the line is vertical. This situation means that for all points on the line, the x-value remains constant while y can change.
Can the slope be a fraction?

+
Yes, the slope can be a fraction, which is common when the change in y and change in x are not whole numbers. This fraction represents the steepness or rate of change in a more precise manner.



