Master Missing Angles with Our Free Worksheet!

Identifying and calculating missing angles is a cornerstone skill in geometry, critical for both academic success and real-world problem-solving. This comprehensive blog post guides you through understanding various types of angles, how to find missing angles, and how to use this knowledge effectively, all enhanced by a tailored worksheet that covers a range of problems from basic to advanced levels.
Understanding Angles

Angles are fundamental in understanding the physical world around us, from architecture to the design of circuits. They represent the amount of rotation or turn between two lines intersecting at a common point. Here’s an overview:
- Acute Angles: Angles that measure less than 90 degrees.
- Right Angles: Angles that measure exactly 90 degrees, often marked with a square in diagrams.
- Obtuse Angles: Angles that measure more than 90 degrees but less than 180 degrees.
- Straight Angles: Angles that measure 180 degrees.
- Reflex Angles: Angles that measure more than 180 degrees but less than 360 degrees.
- Full Angles: Angles that measure exactly 360 degrees.
Knowing these types helps in navigating through complex geometric problems.

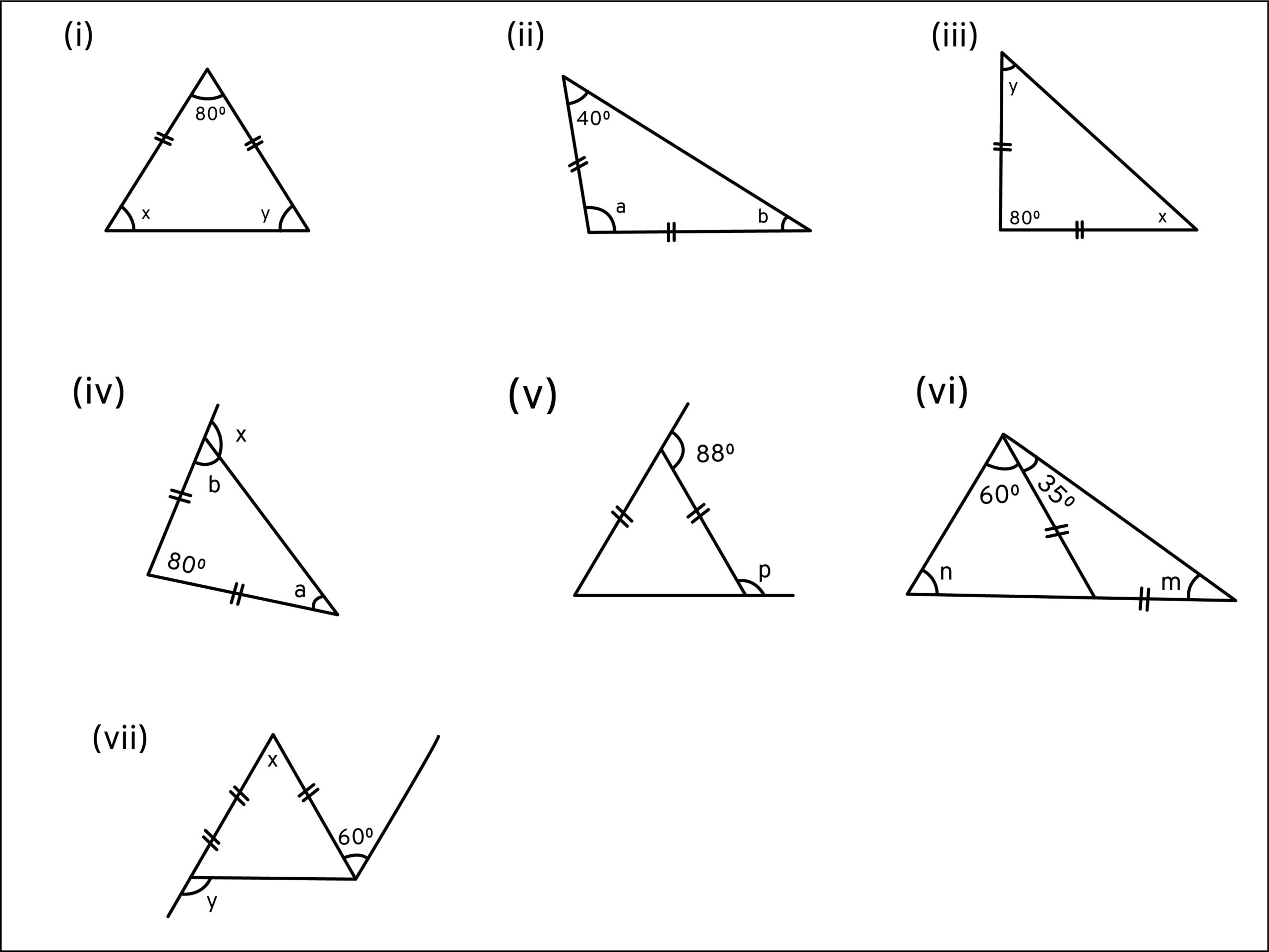
Steps to Find Missing Angles

Finding missing angles can often be straightforward if you follow these steps:
- Identify Angle Relationships: Determine if the angles are complementary, supplementary, vertical, or form part of a triangle or polygon.
- Use Known Properties:
- Complementary angles add up to 90°.
- Supplementary angles add up to 180°.
- Vertical angles are equal.
- Sum of angles in a triangle is 180°.
- The exterior angle of a triangle is equal to the sum of the two non-adjacent interior angles.
- Set Up Equations: Based on the relationships, formulate equations to solve for the unknown angle.
- Solve the Equations: Use algebraic methods to find the values of the missing angles.
- Check Results: Verify your calculations to ensure the sum of angles in the given context adds up correctly.
🌐 Note: Ensure all angles in a triangle or on a straight line add up correctly for accurate geometry problems.
Advanced Techniques for Solving Angle Problems

When dealing with more complex scenarios, consider the following advanced techniques:
- Using Trigonometry: For angles within a triangle or circle, sine, cosine, and tangent ratios can help find unknown angles.
- Circle Properties: Understand properties such as angles subtended by the same arc or inscribed angles.
- Multiple Triangles: When angles are part of multiple interconnected triangles, you might need to solve for angles in stages.
- Rotation and Reflection: Sometimes, you can use rotational symmetry or reflective properties to simplify calculations.
Free Worksheet on Missing Angles

To solidify your understanding and practice these techniques, here’s a free worksheet:
| Problem | Type | Solution |
|---|---|---|
| 1. Find the missing angle in the following triangle where two angles are 45° and 85°. | Triangle | Missing angle = 180° - (45° + 85°) = 50° |
| 2. Identify the missing angle when two angles on a straight line are 50° and 100°. | Straight Line | Missing angle = 180° - 100° = 80° |
| 3. Determine the missing angle in a quadrilateral where three angles are 95°, 80°, and 105°. | Quadrilateral | Missing angle = 360° - (95° + 80° + 105°) = 80° |

Download and print this worksheet for hands-on practice or use it digitally for a quick review.
📋 Note: This worksheet is designed to progress from basic to advanced problems, covering multiple angle relationships.
Real-World Applications of Angle Calculations
The ability to calculate angles isn’t just for academic settings; it has numerous practical applications:
- Engineering and Architecture: Accurate angle measurements are crucial for the design and construction of buildings, bridges, and machinery.
- Aviation and Navigation: Pilots and sailors use angles to determine bearings and paths.
- Surveying and Cartography: Measuring angles accurately helps in plotting land boundaries and creating maps.
- Gaming and Design: Creating realistic angles in video games and design software requires understanding geometry.
- Medicine: In medical imaging, angles help in diagnosing and treating conditions like scoliosis.
Final Thoughts

In conclusion, mastering the calculation of missing angles opens up a world of understanding and application in geometry. From the basic types of angles to their complex interactions within polygons and real-world scenarios, this skill is not only foundational but also universally applicable. The provided worksheet serves as a practical tool to reinforce your knowledge, ensuring you can tackle any geometry problem with confidence. Remember, as you practice these calculations, you’re not just mastering angles but also enhancing your problem-solving capabilities, which are valuable in various fields.
What are the different types of angles?

+
Angles can be classified into several types based on their measurement: acute (less than 90°), right (exactly 90°), obtuse (between 90° and 180°), straight (180°), reflex (more than 180° but less than 360°), and full (360°).
How can I practice finding missing angles?

+
You can practice finding missing angles by using the free worksheet provided above or by creating your own problems based on geometric shapes like triangles, quadrilaterals, or circles.
Why is it important to calculate missing angles?

+
Calculating missing angles is crucial in many practical fields such as engineering, architecture, navigation, and even in everyday tasks like planning a room layout or analyzing structural integrity.