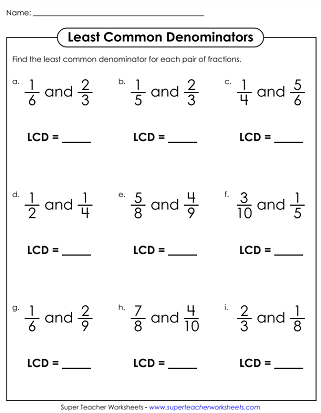
Finding Least Common Denominator Worksheet: Simplify Fractions Easily

When working with fractions, one often overlooked but crucial skill is finding the Least Common Denominator (LCD) to simplify, add, or subtract fractions efficiently. Not only does understanding this concept help in simplifying complex fractions, but it also empowers students and math enthusiasts with a tool to tackle problems more intuitively. Here's an in-depth guide on how to find the LCD and use it to simplify fractions with worksheets to reinforce the learning process.
Understanding Fractions and the Role of LCD

Fractions represent parts of a whole, with a numerator indicating how many parts we have, and a denominator showing how many equal parts the whole is divided into. When we need to add or subtract fractions, we encounter the challenge of different denominators. This is where the LCD comes into play.
- Definition: The Least Common Denominator (LCD) is the smallest (least) common multiple of the denominators of two or more fractions.
- Purpose: It allows for the addition or subtraction of fractions by providing a common ground for different denominators.
Step-by-Step Process to Find the LCD

Finding the LCD involves several methods, but we'll focus on the most straightforward approaches:
Prime Factorization Method

Here's how to find the LCD using prime factorization:
- Write down the denominators of the fractions.
- Find the prime factors of each denominator.
- Identify the highest power of all prime factors that appear in the factorizations.
- Multiply these highest powers together to get the LCD.
Example: Find the LCD for 1/4 and 2/6:
| Prime Factors: | 4 = 2 2 | 6 = 2 * 3 |
| Highest Powers: | 2 2 | 3 |
| LCD: | 2 2 * 3 = 4 * 3 = 12 | |

🔍 Note: You don't always need to include every prime factor if a lower power of a prime already suffices.
Least Common Multiple (LCM) Method

Another approach uses the Least Common Multiple (LCM) of the denominators:
- List the multiples of each denominator.
- Find the smallest multiple that both numbers have in common.
- This number is your LCD.
Example: Find the LCD for 3/8 and 5/12:
- Multiples of 8: 8, 16, 24, 32...
- Multiples of 12: 12, 24, 36...
- LCD = 24
Applying the LCD to Simplify Fractions

Once you have the LCD, you can add or subtract fractions, and simplify them:
Addition and Subtraction
- Convert each fraction to an equivalent fraction with the LCD as its denominator.
- Add or subtract the numerators, keeping the LCD as the denominator.
- Simplify if necessary.
Example: Simplify (1/4) + (2/6):
- LCD = 12
- Convert: 1/4 becomes 3/12 and 2/6 becomes 4/12
- Add: (3/12) + (4/12) = 7/12
- 7/12 is already in its simplest form.
Worksheet for Practice

Here's a worksheet to help you practice finding LCD and simplifying fractions:
- Find the LCD for the following fractions:
- 1/5 and 2/10
- 3/8 and 5/6
- 2/3 and 4/9
- Perform the following operations using the LCD method:
- Add: 1/4 + 1/6
- Subtract: 3/7 - 2/5
- Add: 5/12 + 1/8
📘 Note: Remember, the aim of practice is to get comfortable with the process. Don't hesitate to use different approaches to find the LCD until one method clicks.
Why Use LCD?

Mastering the use of LCD:
- Reduces errors in fraction arithmetic.
- Helps in understanding and simplifying complex mathematical problems.
- Prepares for higher math concepts like algebra and calculus.
In summary, finding the LCD is an essential skill for working with fractions. It enables one to add, subtract, or compare fractions effortlessly. By understanding prime factorization and the LCM method, you can find the LCD quickly. Use the practice worksheet to hone your skills and make fraction arithmetic as easy as pie.
Why is finding the LCD important when adding or subtracting fractions?

+
Finding the LCD allows you to transform fractions with different denominators into fractions with a common denominator, which is essential for adding or subtracting them correctly.
What’s the difference between finding the GCF (Greatest Common Factor) and the LCD?

+
The GCF is used to simplify fractions by dividing the numerator and the denominator by their largest common factor, while the LCD is used to convert fractions to a common base for arithmetic operations.
Can you find the LCD of more than two fractions?

+
Absolutely! The method involves finding the LCM (or prime factorization) of all the denominators involved.
What if a fraction cannot be simplified after finding the LCD?

+
That’s perfectly fine. Not all fractions need to be simplified after operations. However, if simplification is desired, check if the numerator and the denominator have a common factor other than 1.
Are there online tools to help with finding the LCD?

+
Yes, there are numerous online calculators and tools that can instantly compute the LCD for fractions. However, understanding the manual process is important for grasping the underlying math concepts.