5 Ways to Master Angles in Geometry Worksheets

The Importance of Angles in Geometry

Angles are fundamental to understanding geometry. They are the building blocks that help us define shapes, determine relationships between lines, and solve complex geometric problems. Without angles, geometry would be a flat and uninspiring field. In this blog post, we'll delve into 5 Ways to Master Angles in Geometry Worksheets to help you understand, solve, and become proficient in this essential aspect of geometry.
Understanding Types of Angles


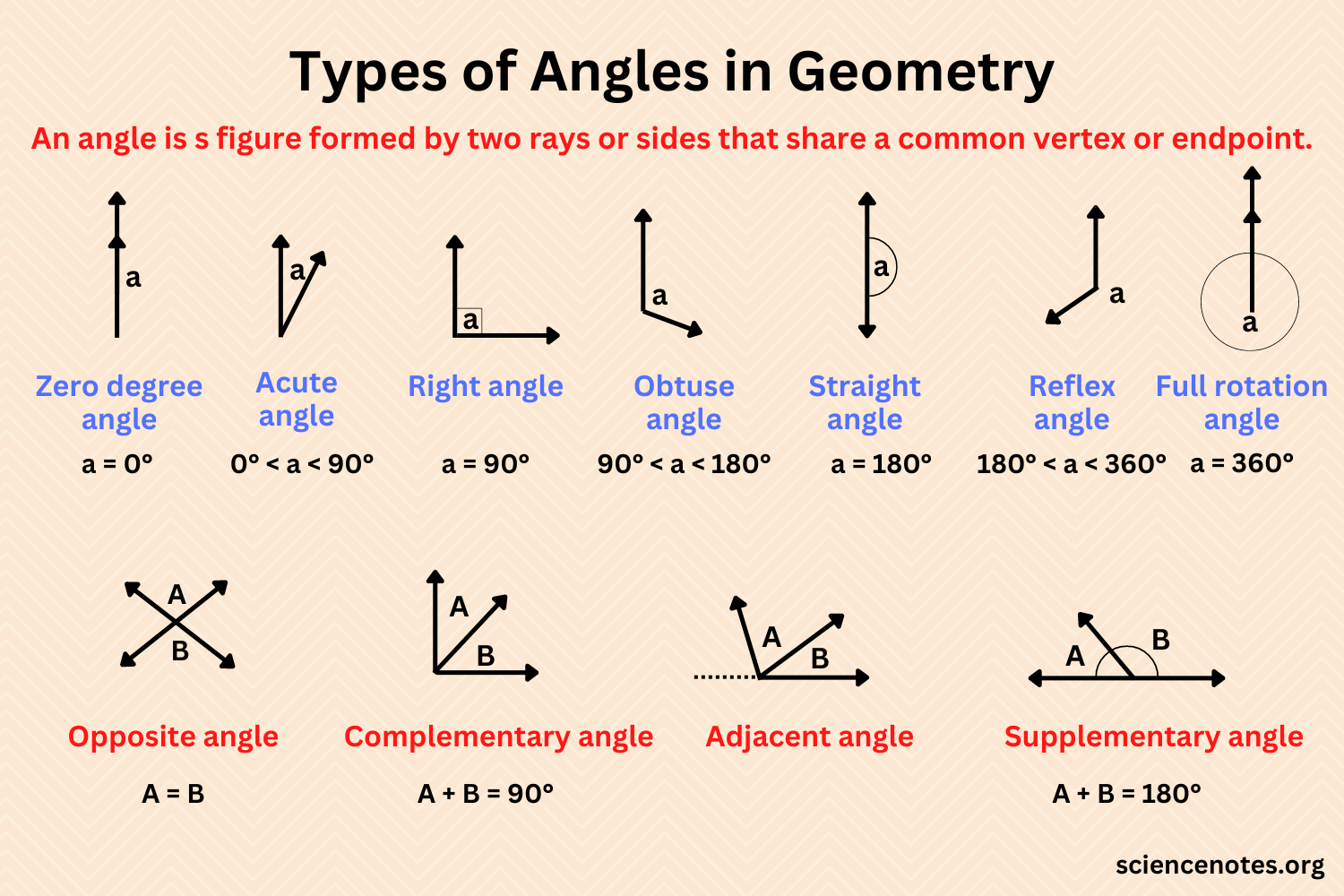
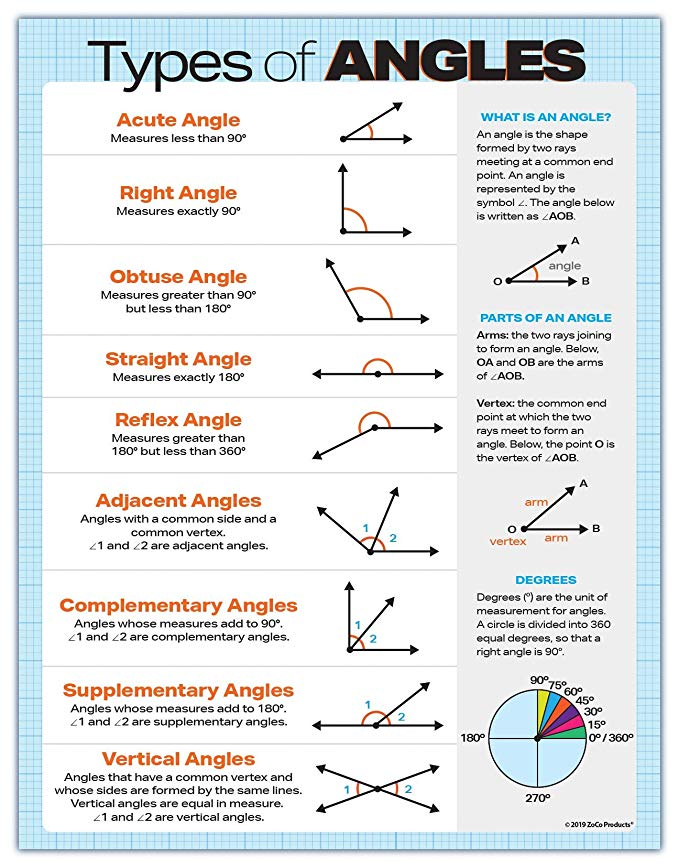
Before diving into problem-solving, let's get a firm grasp of the different types of angles:
- Acute Angle: Less than 90°
- Right Angle: Exactly 90°
- Obtuse Angle: Between 90° and 180°
- Straight Angle: Exactly 180°
- Reflex Angle: Between 180° and 360°
- Full Angle: Exactly 360°
Understanding these types will set a solid foundation for tackling geometry worksheets effectively.
1. Visualize the Geometry


Geometry worksheets often involve abstract shapes and angles. Here's how to visualize better:
- Draw it Out: Use a protractor and pencil to draw the angles, even if they are not to scale, to get a physical representation.
- Label Angles: Naming angles with letters or numbers can help keep track of relationships between different angles within a figure.
- Color Code: Use different colors to distinguish between different types of angles or sections of a figure to make relationships clearer.
📌 Note: Visualizing helps in identifying patterns and understanding spatial relationships, making solving problems much easier.
2. Apply Angle Properties

Utilize the properties of angles to your advantage:
- Angles on a Straight Line: Sum to 180°.
- Angles around a Point: Sum to 360°.
- Complementary Angles: Two angles whose measures add up to 90°.
- Supplementary Angles: Two angles whose measures add up to 180°.
- Vertical Angles: Opposite angles formed by two intersecting lines are equal.
3. Practice Problem-Solving Techniques


Geometry worksheets often include problems requiring algebraic manipulation:
- Set Up Equations: Use known angle properties to form equations that can be solved.
- Solve for Variables: Utilize algebra to find unknown angles.
- Check Your Work: After solving, ensure your answer fits the problem context by plugging it back into your equations.
📌 Note: Do not underestimate the power of algebra when dealing with angles in geometry.
4. Use Geometry Tools
Physical and digital tools can be invaluable in understanding angles:
- Protractors: A must-have for drawing and measuring angles accurately.
- Straightedges: Help in creating lines and extending rays to measure angles.
- Geometry Software: Tools like GeoGebra or Desmos Geometry provide dynamic, interactive environments for exploration.
Engaging with these tools can transform your ability to perceive and work with angles in a three-dimensional space.
5. Embrace Trigonometry

While this might sound advanced, trigonometry becomes a friend when dealing with angles:
- SOH-CAH-TOA: These formulas provide ways to find angles in right triangles.
- Law of Sines and Cosines: Applicable in all triangles for finding angles and side lengths.
These concepts might not always be necessary for basic geometry, but knowing them allows you to tackle problems in a more sophisticated manner.
Final Thoughts

Mastering angles in geometry worksheets involves a combination of conceptual understanding, practical application, and the use of appropriate tools. By approaching these worksheets systematically, visualizing relationships, and leveraging algebra and trigonometry, you'll not only excel in geometry but also build a strong foundation for more advanced mathematics. Remember, practice is the key to proficiency, so dive into geometry worksheets with enthusiasm, knowing that each problem solved enhances your understanding and skill set.
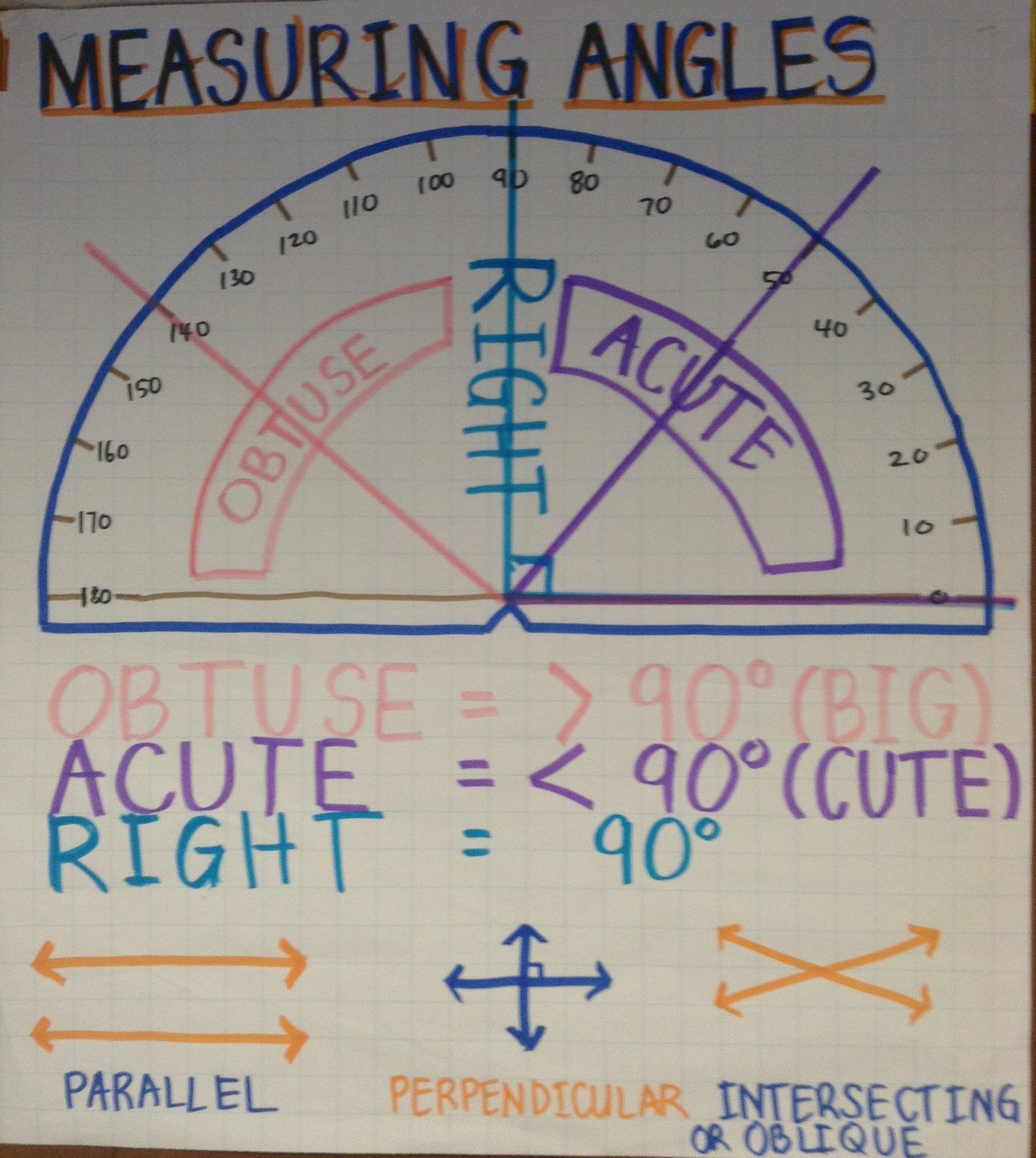
How do you measure angles accurately?
+
Use a protractor to measure angles. Align one ray of the angle with the baseline of the protractor, then read the degree where the other ray intersects the protractor.
What are the most common mistakes students make when solving angle problems?
+
Common mistakes include mixing up angle types, forgetting to account for straight or full angles, miscalculating complementary or supplementary angles, and not considering the full context of a problem.
Can geometry software really help with understanding angles?

+
Yes, software like GeoGebra or Desmos Geometry allows for dynamic manipulation of shapes and angles, providing immediate visual feedback on how angles relate to each other, which can deepen understanding.