Master Factoring Polynomials with GCF Worksheet Guide
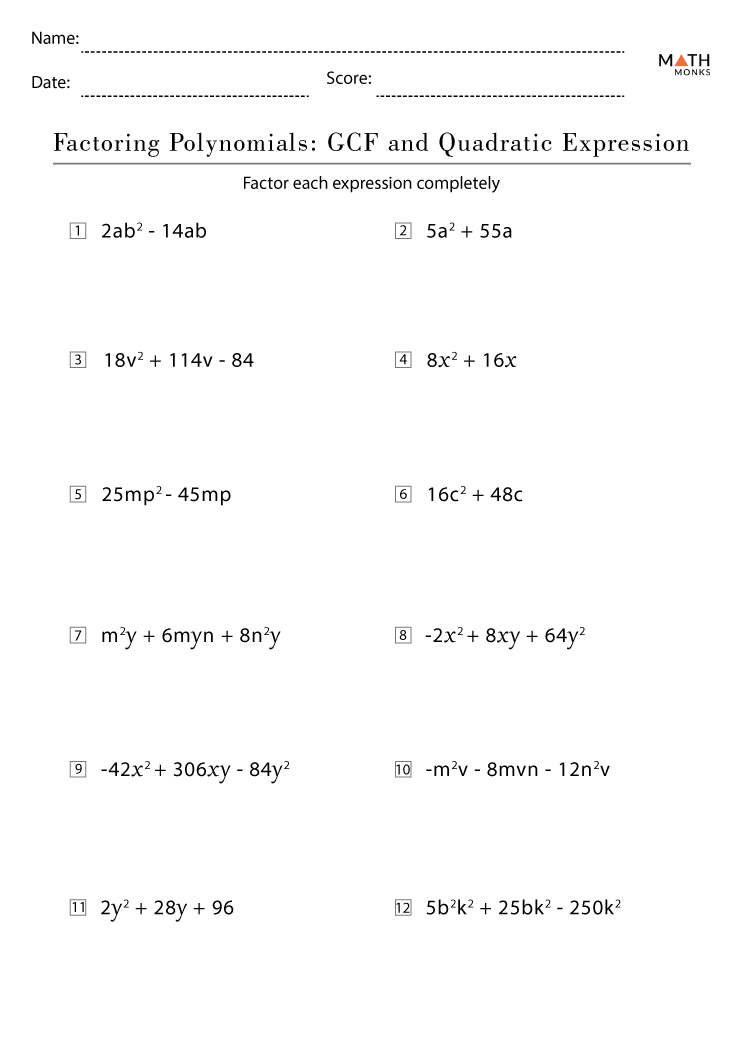
Factoring polynomials is a fundamental skill in algebra that can simplify expressions and make solving equations easier. In this comprehensive guide, we'll explore the process of factoring polynomials using the greatest common factor (GCF) method. Whether you're a student struggling with algebra or a teacher looking for effective teaching resources, this guide will provide you with step-by-step instructions, examples, and a handy worksheet to master the technique.
Why Factoring Polynomials Matters
Factoring polynomials is crucial for several reasons:
- Equation Solving: It allows for straightforward solutions to quadratic and higher-degree equations by breaking them into simpler factors.
- Graphing: Understanding factorization helps in sketching polynomial functions’ graphs by identifying roots and turning points.
- Simplification: It simplifies complex algebraic expressions, making them easier to manage in more advanced mathematical contexts.
The Greatest Common Factor Method
The GCF method involves pulling out the largest factor common to all terms in a polynomial. Here’s how you do it:
- Identify the GCF: Look for the largest number or variable that divides each term evenly.
- Divide Each Term by GCF: Rewrite the polynomial with each term divided by the GCF.
- Put GCF Outside Parentheses: Place the GCF in front of parentheses, and inside the parentheses put what remains after division.
Let's look at an example:
Example:
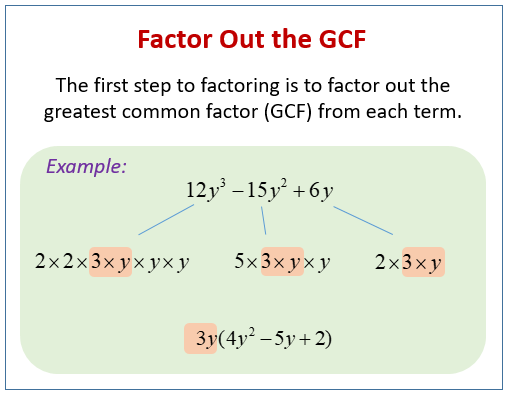
Factor the polynomial: 12x^3 + 18x^2 + 6x
- The GCF of 12x^3, 18x^2, and 6x is 6x.
- Divide each term by 6x:
- 12x^3 ÷ 6x = 2x^2
- 18x^2 ÷ 6x = 3x
- 6x ÷ 6x = 1
- Now, place the GCF outside the parentheses: 6x(2x^2 + 3x + 1)
📝 Note: Ensure all terms in the polynomial share at least one common factor for this method to be applicable.
Worksheet to Practice
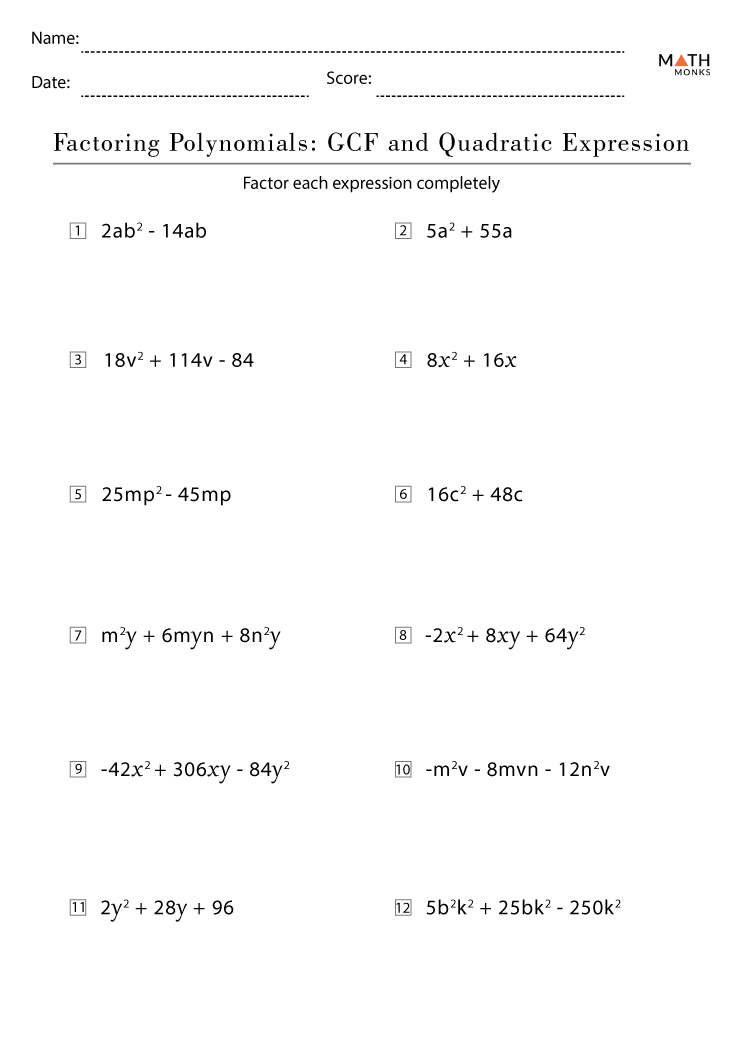
To help you master factoring with GCF, here’s a worksheet:
| Original Polynomial | Factored Form |
|---|---|
| 18x^2 + 6x | ___(___x + ___) |
| 10x^3 + 15x^2 | ___(___x^2 + ___x) |
| 7a^3 + 21a^2 | ___(___a^2 + ___) |
| 3y^4 + 9y^3 + 6y^2 | ___(___y^3 + ___y^2 + ___y) |

Practical Tips for Factoring

- Check for Common Factors: Always look for the largest common factor before attempting other factoring methods.
- Focus on One Term at a Time: If not all terms share a common factor, look for the GCF between pairs or groups of terms.
- Be Mindful of Negative Signs: If a common factor is negative, factor it out as a positive number but place a negative sign in front of the parentheses.
When GCF Method Isn’t Enough
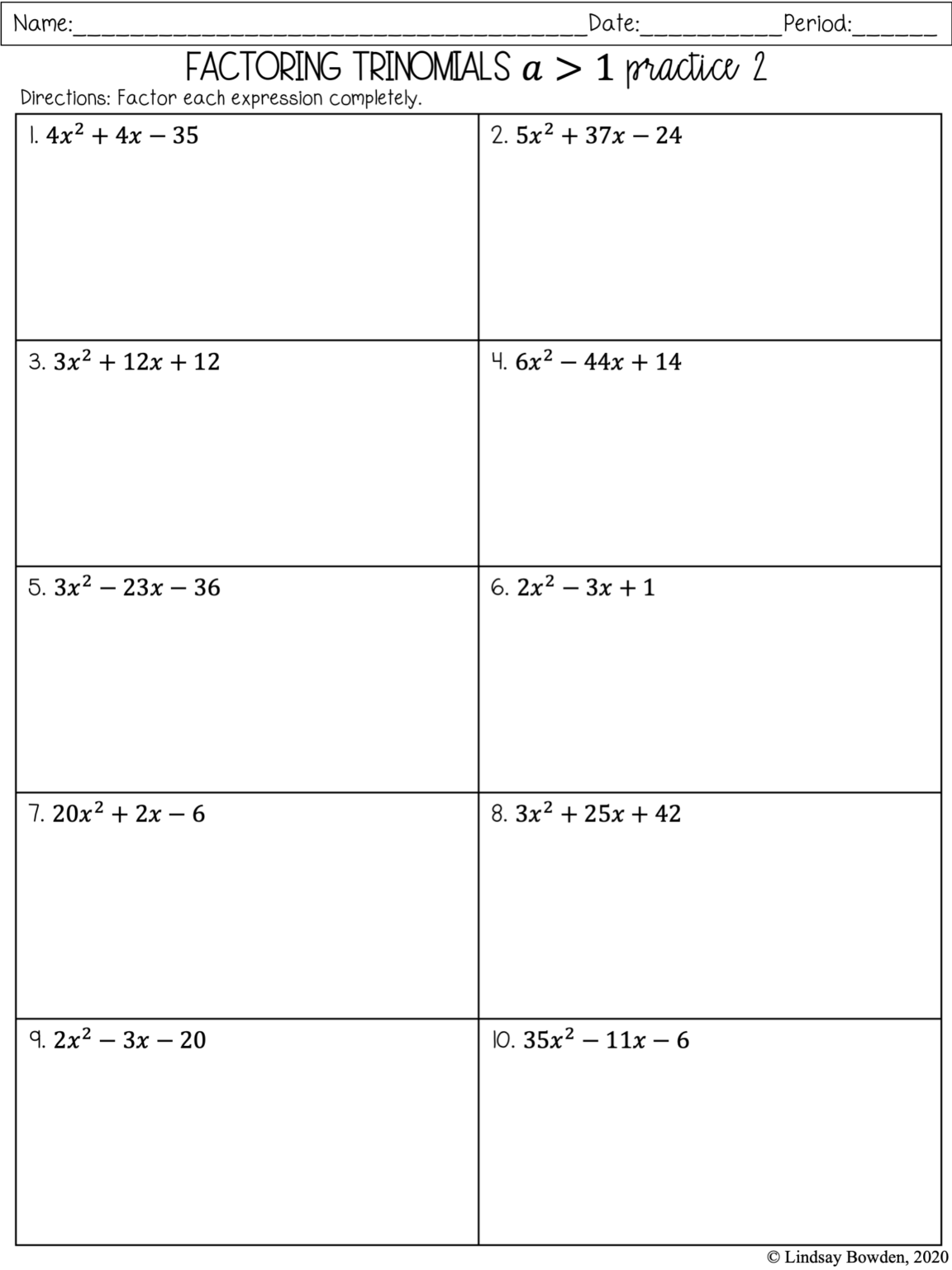
Sometimes, polynomials might not be completely factorable with just the GCF method. Here are some scenarios where you might need other techniques:
- Polynomials with no common factors among all terms.
- Polynomials where the GCF is 1, indicating no common factors to pull out.
💡 Note: In cases where the GCF is 1, you might need to use other factorization methods like grouping, binomial expansion, or the quadratic formula.
With these techniques and practice through the provided worksheet, you're on your way to becoming adept at factoring polynomials. Remember, the key is understanding the basic principles and then applying them consistently. Over time, you'll recognize patterns and make connections that simplify even the most complex expressions.
What is the GCF method of factoring?

+
The GCF method involves identifying the largest number or variable that divides all terms in a polynomial, pulling it out, and placing the remaining expression in parentheses.
Why do we factor polynomials?
+
Factoring polynomials helps in solving equations, simplifying expressions, and understanding the behavior of functions through their roots and graph analysis.
What if there is no common factor?

+
If no common factor exists among all terms, consider alternative factoring methods like grouping or binomial expansion. If no factorization is possible, the polynomial might be irreducible or require further methods for decomposition.