5 Factoring Polynomials Tricks for Instant Results

Polynomials are mathematical expressions consisting of variables and coefficients, combined with arithmetic operations and exponents. For many students and professionals alike, factoring polynomials is an essential algebraic skill. It simplifies the expression, making it easier to solve, graph, or manipulate in various mathematical contexts. Here are five key strategies or "tricks" to quickly and effectively factor polynomials for instant results.
Finding the Greatest Common Factor (GCF)

The first step in factoring any polynomial is to look for the Greatest Common Factor (GCF) among all its terms. This is not only a starting point but can often simplify your work significantly:
- Identify the GCF: Look at all the terms in the polynomial and find the largest number or variable that evenly divides each term.
- Factor out the GCF: Use the GCF to factor out common factors, reducing the complexity of the polynomial.
🔍 Note: If the GCF is only a 1, consider other factoring methods as the polynomial may already be in its simplest form.

Using the Difference of Squares

The difference of squares is a formula that comes in handy when factoring expressions where you have two squares being subtracted from each other:
- The formula for the difference of squares is ( a^2 - b^2 = (a + b)(a - b) ).
- Look for terms in your polynomial that match the pattern ( a^2 - b^2 ).
| Example Polynomial | Factored Form |
|---|---|
| (x^2 - 9) | ((x - 3)(x + 3)) |
| (4x^2 - 49) | ((2x - 7)(2x + 7)) |

📚 Note: The difference of squares works because ( (a + b)(a - b) ) is a perfect product of conjugate binomials.

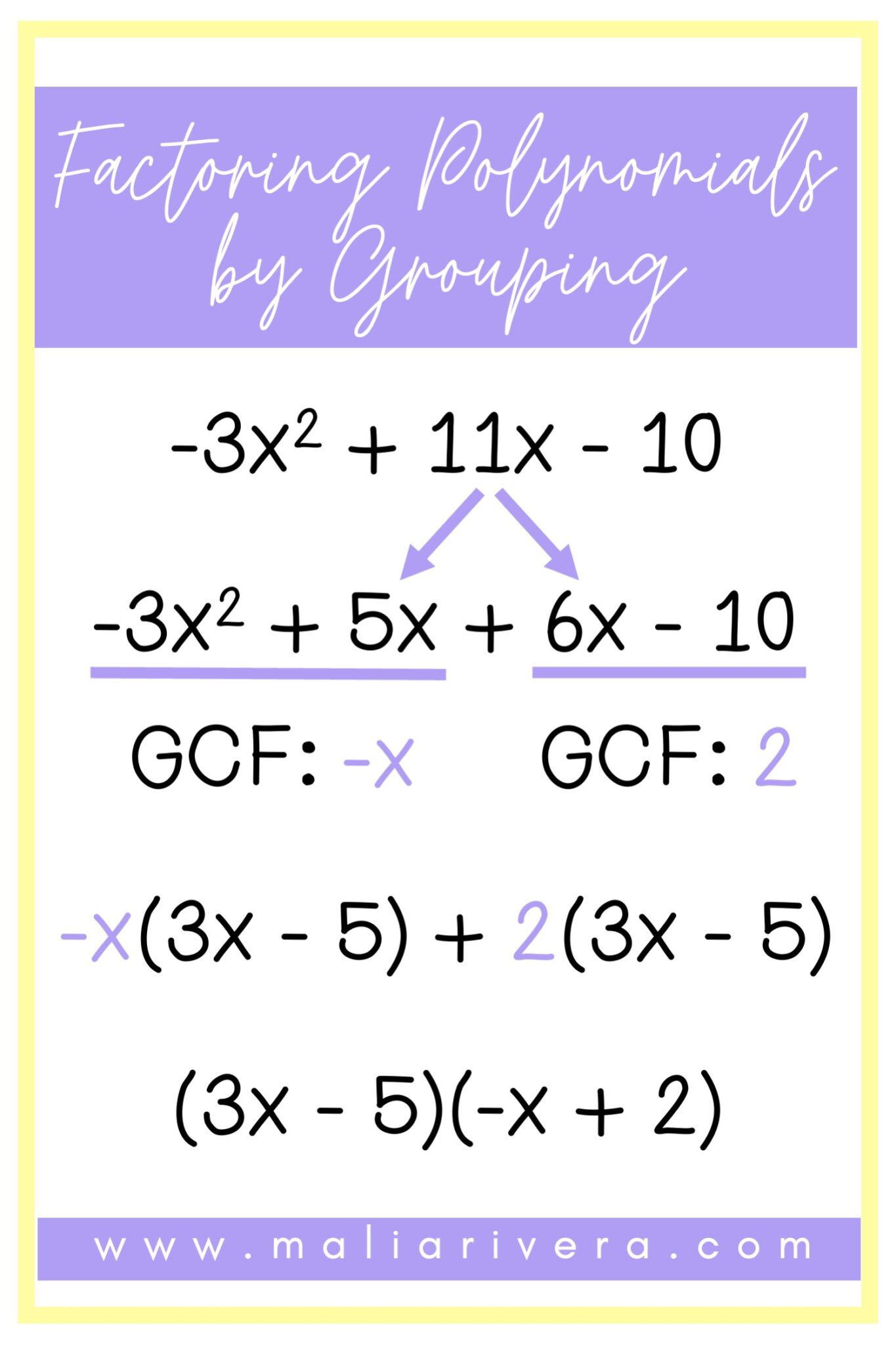
Factoring Trinomials: AC Method

The AC method is particularly useful for trinomials where the leading coefficient is not one. Here’s how to use it:
- Identify the trinomial: ( ax^2 + bx + c ).
- Multiply the leading coefficient (a) and the constant term ©: This product helps you find factors.
- Find factors of ( ac ) that add up to b: Use these factors to split ( bx ).
- Factor by grouping: Split the polynomial into groups and factor out common terms.
Let’s illustrate with an example:
- For ( 6x^2 + 11x + 4 ):
- Multiply 6 and 4 to get 24.
- Find factors of 24 that add to 11: 8 and 3.
- Split ( 11x ) into ( 8x + 3x ).
- Group and factor out: ( 6x^2 + 8x + 3x + 4 \rightarrow 2x(3x + 4) + 1(3x + 4) \rightarrow (3x + 4)(2x + 1) ).

Looking for Perfect Square Trinomials

Perfect square trinomials are trinomials that can be written as the square of a binomial. Identifying these can save time:
- The form is ( a^2 + 2ab + b^2 = (a + b)^2 ).
- Or, ( a^2 - 2ab + b^2 = (a - b)^2 ).
- When you see this pattern, factor immediately to the binomial squared form.
For example:
- (x^2 + 6x + 9 = (x + 3)^2)
- (4x^2 - 12x + 9 = (2x - 3)^2)
💡 Note: Perfect square trinomials come up frequently in calculus and solving quadratic equations.

Synthetic Division

Synthetic division is a method used when you suspect that a polynomial has a specific factor:
- Identify a possible root: Use the Rational Root Theorem or test values by synthetic division.
- Perform synthetic division: This can be a quick way to confirm or find factors for higher-degree polynomials.
An example would be:
- For the polynomial ( x^3 - 6x^2 + 11x - 6 ):
- Possible roots are 1, 2, 3, and 6.
- Using synthetic division, you find that x = 2 is a root, leaving you with ( (x - 2)(x^2 - 4x + 3) ).

Summing up, mastering these techniques will not only help in solving polynomial equations but also significantly improve your algebraic problem-solving skills. Each method offers a unique approach to factoring, making complex polynomials manageable. Remember, the key to instant results in factoring lies in recognizing patterns and applying the appropriate method swiftly.
What if none of these methods work?

+
If you’re unable to factor using these tricks, the polynomial might be prime, or you might need to use more advanced techniques like polynomial long division or look for complex roots.
How do I know which factoring method to use?

+
Start with the simplest method: look for the GCF. Then check for known patterns like the difference of squares or perfect square trinomials. If these don’t work, try the AC method for trinomials, or use synthetic division for polynomials of higher degree.
Can these methods be used in calculus?
+
Yes, many calculus problems, particularly those involving derivatives or integrals of polynomials, require factoring. Knowing these methods will aid in simplifying expressions and solving equations.