5 Simple Steps to Factor Monomials Easily
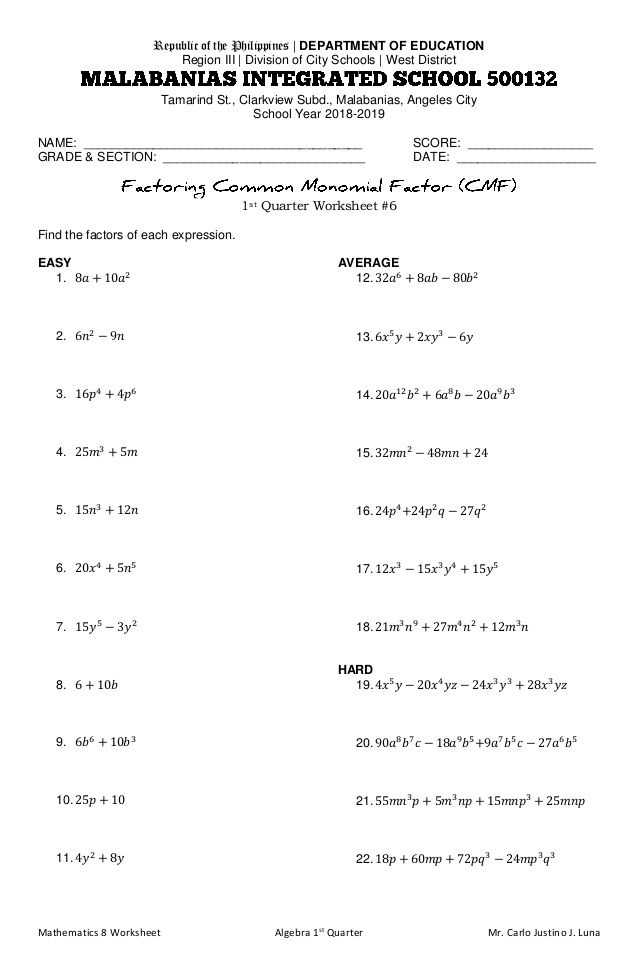
Algebra is often viewed as a daunting branch of mathematics, but with the right approach, even its most challenging aspects can become straightforward. Factoring monomials, or finding the common factors among them, is a fundamental skill in algebra that lays the groundwork for further algebraic manipulations and problem-solving. In this detailed guide, we'll walk through 5 simple steps that make factoring monomials an easy task, ensuring you grasp this concept thoroughly.
Step 1: Identify the Coefficients

A monomial is a polynomial with only one term, which includes a coefficient, variables, and exponents. Here's how you start:
- Examine the coefficients: Look at the numerical part of each monomial. The coefficient is the number that comes before any variables.
- Identify the greatest common factor (GCF) of these coefficients. This will be the numeric factor for your result.
- If the monomials have no common factors greater than 1, then the numeric factor in the factorization will be 1.
💡 Note: Coefficients are critical in determining how you will factor your monomials, as they dictate the common factor's numeric component.
Step 2: Look for Common Variables
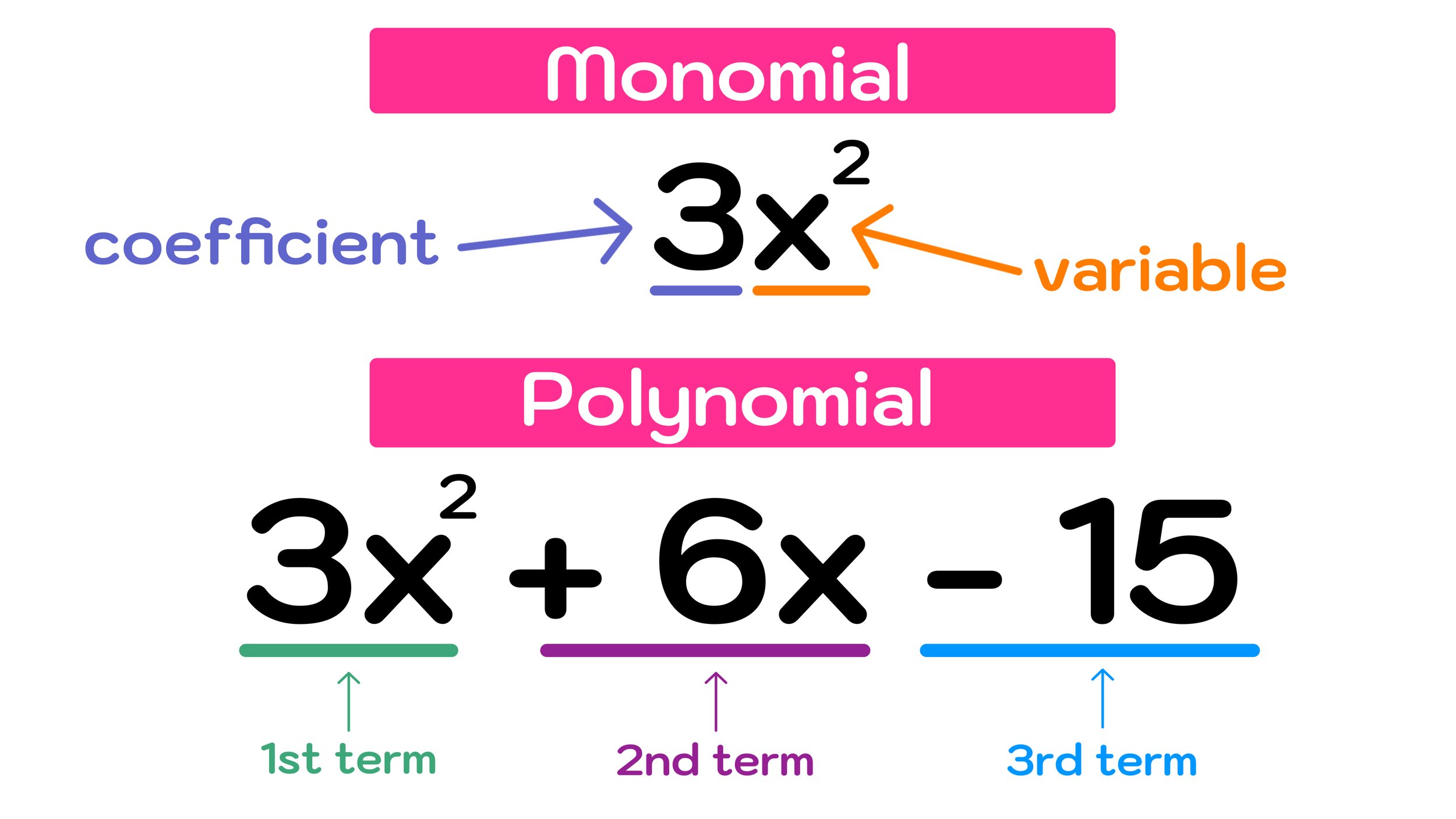
After addressing the coefficients:
- Identify all the variables in each monomial. Remember, variables can appear multiple times with different exponents.
- Find the common variables across all monomials.
- Determine the lowest exponent for each common variable to find the GCF of variables.
For example, in 12x^3, 16x^2y, and 8xy^3, the common variable is 'x'. The lowest exponent for 'x' is 1, making the common factor 'x'.
Step 3: Combine Coefficients and Variables

Once you've found the GCF for both coefficients and variables:
- Combine the GCF of the coefficients with the GCF of the variables to create a single term. This term is the common factor you'll extract from each monomial.
Using our example:
- Monomials: 12x^3, 16x^2y, 8xy^3
- GCF of coefficients: 4
- GCF of variables: x
- Combined GCF: 4x
Step 4: Factor Out the GCF

Now that you have the common factor:
- Divide each monomial by this common factor. This will give you the remaining factors after factoring out the GCF.
- Write down the factored form, where the GCF is extracted and placed outside, followed by the remaining terms in parentheses.
For instance:
- 12x^3 ÷ 4x = 3x^2
- 16x^2y ÷ 4x = 4xy
- 8xy^3 ÷ 4x = 2y^3
Resulting in 4x(3x^2 + 4xy + 2y^3)
Step 5: Check Your Work

Always verify your factoring:
- Multiply the factored expression back together to ensure it equals the original polynomial.
- If it doesn't match, revisit your steps to identify and correct any errors.
🔎 Note: Checking your work is an essential step in mathematics, as it helps you catch and correct mistakes.
In wrapping up, this method for factoring monomials streamlines a process that might seem complex at first. By breaking down the task into these 5 simple steps, you can quickly grasp the essence of factoring monomials:
- Identifying the coefficients
- Looking for common variables
- Combining these into a GCF
- Factoring out the GCF
- Verifying the factoring
This approach ensures that you have a robust understanding of the process and can apply it confidently to various algebraic expressions. Factoring monomials is not just about following rules; it's about understanding the logic behind each step, which makes algebra not only manageable but also quite enjoyable. Through these steps, you're not just solving problems; you're building a foundation for more advanced algebraic concepts.
What if there’s no common factor?

+
If there’s no common factor other than 1, the monomials are said to be relatively prime, and you factor out just a 1, essentially leaving the monomials unchanged.
Can I factor monomials with negative coefficients?

+
Yes, you can factor out negative coefficients just as you would with positive ones. Just ensure you follow the same steps for identifying the GCF, which might include negative numbers.
How can factoring monomials help with solving equations?

+
Factoring monomials can simplify algebraic expressions, making it easier to solve equations by eliminating common factors, reducing complexity, and sometimes directly leading to the solution.



