GCF Factoring Worksheets: Easy Answers Included

Factoring Greatest Common Factors (GCF) is a fundamental algebraic skill that not only helps students understand the structure of polynomials but also simplifies complex problems in algebra, calculus, and beyond. This blog post will guide you through the process of factoring polynomials by using GCF worksheets, complete with answers to help reinforce your understanding and practice. Let's explore why GCF factoring is essential, how to do it, and where you can find resources to practice.

Why Learn GCF Factoring?

• Simplicity: It simplifies polynomial expressions by breaking them down into manageable parts.
• Preparation: It prepares you for more advanced algebraic techniques like factoring by grouping or quadratic factoring.
• Real-world applications: Many mathematical models and equations in science and engineering use factored forms of polynomials.
• Problem-solving: Factoring is often the key step in solving polynomial equations and inequalities.

How to Factor Using the Greatest Common Factor

Here are the steps to factor polynomials using the GCF:

1. Identify the terms: List all the terms of the polynomial.
2. Find the GCF: Determine the Greatest Common Factor of all these terms. This involves finding the highest number or variable that divides all terms.
3. Factor out the GCF: Write the GCF outside parentheses and inside, write the quotient of each term divided by the GCF.
4. Verify: Check your factorization by multiplying the factors together to ensure they equal the original polynomial.

Practical Examples with Worksheets

Let’s delve into some practical examples using worksheets to illustrate the process:

Example 1:

Problem: Factor the polynomial: 15x + 30

Solution:

1. Identify the terms: 15x and 30.
2. Find the GCF: The GCF of 15 and 30 is 15, and since there are no variables in common, the GCF is 15.
3. Factor out the GCF:

   GCF (15x + 30)

   = 15(1x + 2)

   
   
4. Verify: Multiplying back gives us 15x + 30, confirming our factorization.

💡 Note: Remember to always check your factorization by distributing the GCF back through the parentheses.

Example 2:

Problem: Factor the polynomial: 28a³ - 14a² + 7a

Solution:

1. Identify the terms: 28a³, -14a², and 7a.
2. Find the GCF: The GCF of 28, 14, and 7 is 7. The GCF of a³, a², and a is ‘a’.
3. Factor out the GCF:

   GCF (28a3 - 14a2 + 7a)

   = 7a(4a2 - 2a + 1)

4. Verify: Multiplying 7a with the terms inside the parentheses gives us the original polynomial.

Example 3:

Problem: Factor the polynomial: 3x²y + 9xy² - 6xy

Solution:

1. Identify the terms: 3x²y, 9xy², and -6xy.
2. Find the GCF: The GCF of 3, 9, and 6 is 3. The GCF of x²y, xy², and xy is ‘xy’.
3. Factor out the GCF:

   GCF (3x2y + 9xy2 - 6xy)

   = 3xy(x + 3y - 2)

4. Verify: Multiplying 3xy back confirms our factorization.

Where to Find GCF Factoring Worksheets?

Here are some resources where you can find GCF factoring worksheets to practice:

• MathsDrills: Offers downloadable worksheets tailored to different levels of students.
• Kuta Software: Known for its comprehensive worksheet generators, including GCF factoring.
• Education.com: Provides a variety of printable math worksheets for all levels of education.
• CK-12: An open educational resource with practice problems and solutions.

Benefits of Using Worksheets for Practice

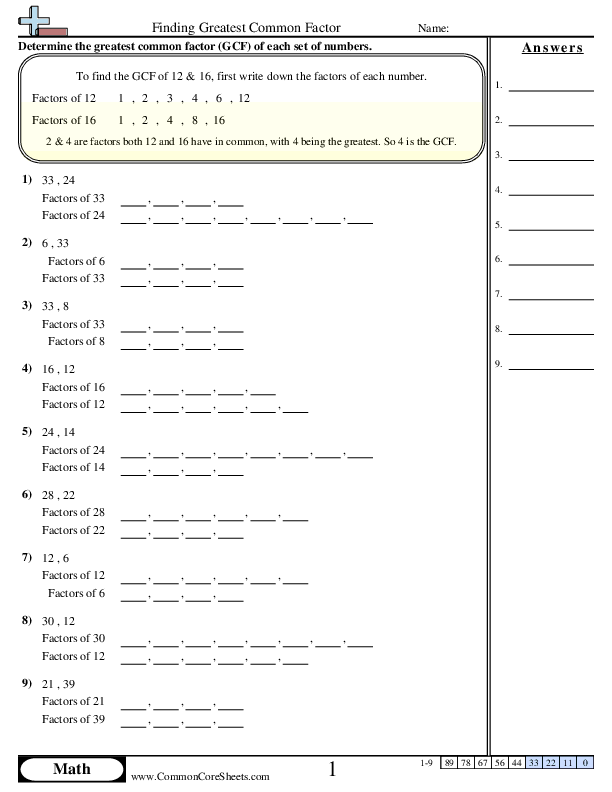
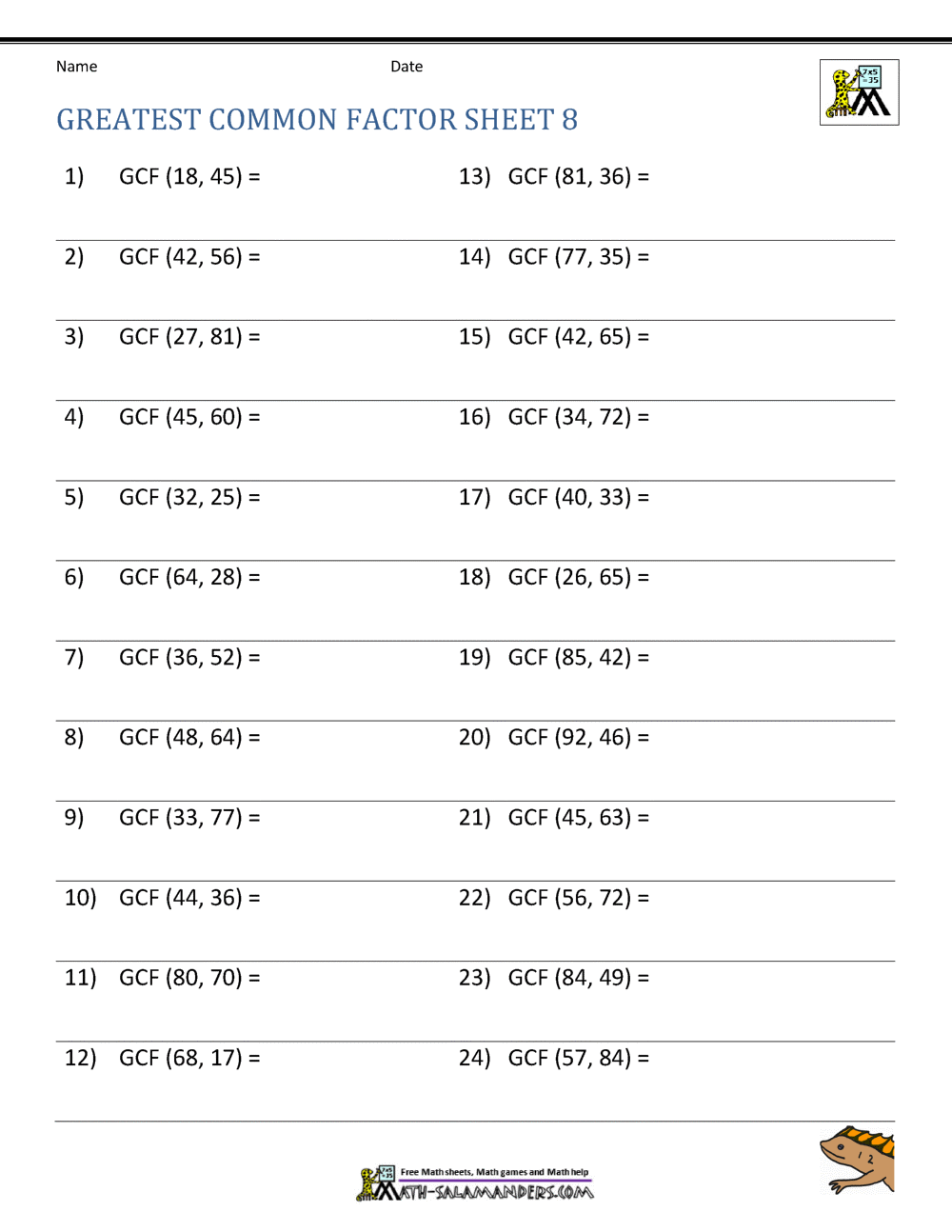
• Repetition: Repetition through worksheets helps solidify the concept in your memory.
• Instant Feedback: Worksheets often include answers, allowing for immediate self-correction.
• Skill Level: You can find worksheets tailored to your skill level, from basic to advanced problems.
• Confidence Building: Successfully completing worksheets builds confidence in applying mathematical concepts.

Final Thoughts

Factoring polynomials using the GCF is an essential algebraic technique that serves as a cornerstone for more complex mathematical processes. By understanding the basics of finding the GCF, practicing with worksheets, and verifying your work, you’re setting a solid foundation for your mathematical journey. Worksheets provide an excellent avenue for practice, ensuring you get ample repetition to master the skill, understand your mistakes, and improve. Remember, consistent practice, coupled with a clear understanding of the process, will make you adept at GCF factoring, benefiting you in mathematics and beyond.

What is the difference between the GCF and the LCM?

+

The GCF (Greatest Common Factor) is the largest factor shared by two or more numbers or variables, while the LCM (Least Common Multiple) is the smallest multiple that both numbers have in common. Essentially, GCF is used for simplifying polynomials and expressions, whereas LCM is more commonly used in operations like adding or subtracting fractions.

Can every polynomial be factored using GCF?

+

Not every polynomial can be factored using GCF if there are no common factors among the terms. However, GCF factoring is often the first step in factoring polynomials, leading to other methods if necessary.

Why is factoring important in algebra?

+

Factoring is crucial because it simplifies expressions, making them easier to work with in algebraic operations like solving equations, simplifying rational expressions, and understanding the behavior of polynomial functions.