5 Essential Tips for Factoring a 1 Worksheet

The journey through algebra can be both exciting and intimidating, especially when it comes to factoring polynomials. If you've landed on a 1 worksheet, you're likely delving into the basics of factoring, which is fundamental to mastering higher-level math. Here, we'll explore five essential tips that will not only help you factor polynomials with greater ease but also improve your overall understanding of algebra.
Understand the Basics of Factoring

Before we dive into specific tips, let's ground ourselves with what factoring means. Factoring involves breaking down a polynomial into a product of its factors, where each factor is either a polynomial of lower degree or a monomial. The goal is to express the polynomial in a form that's easier to work with:
- Greatest Common Factor (GCF): Always look for the GCF first. This is the largest factor that divides all terms of the polynomial evenly.
- Difference of Squares: Remember the formula a^2 - b^2 = (a + b)(a - b).
- Perfect Square Trinomials: These can be factored using the formulas (a + b)^2 = a^2 + 2ab + b^2 or (a - b)^2 = a^2 - 2ab + b^2.
Tip 1: Start with GCF

Identifying the GCF is often the simplest and most effective first step in factoring:
- Examine all the terms of the polynomial to find the GCF. This could be a number, a variable, or a combination of both.
- Divide each term by the GCF and write this as a separate factor. The remaining polynomial becomes the second factor.
Here's an example:
| Original Polynomial | Factored Form |
|---|---|
| 6x3 - 9x2 + 3x | 3x(2x2 - 3x + 1) |

🔍 Note: Always ensure the GCF is factored out completely to avoid missing any common factors.
Tip 2: Utilize Difference of Squares
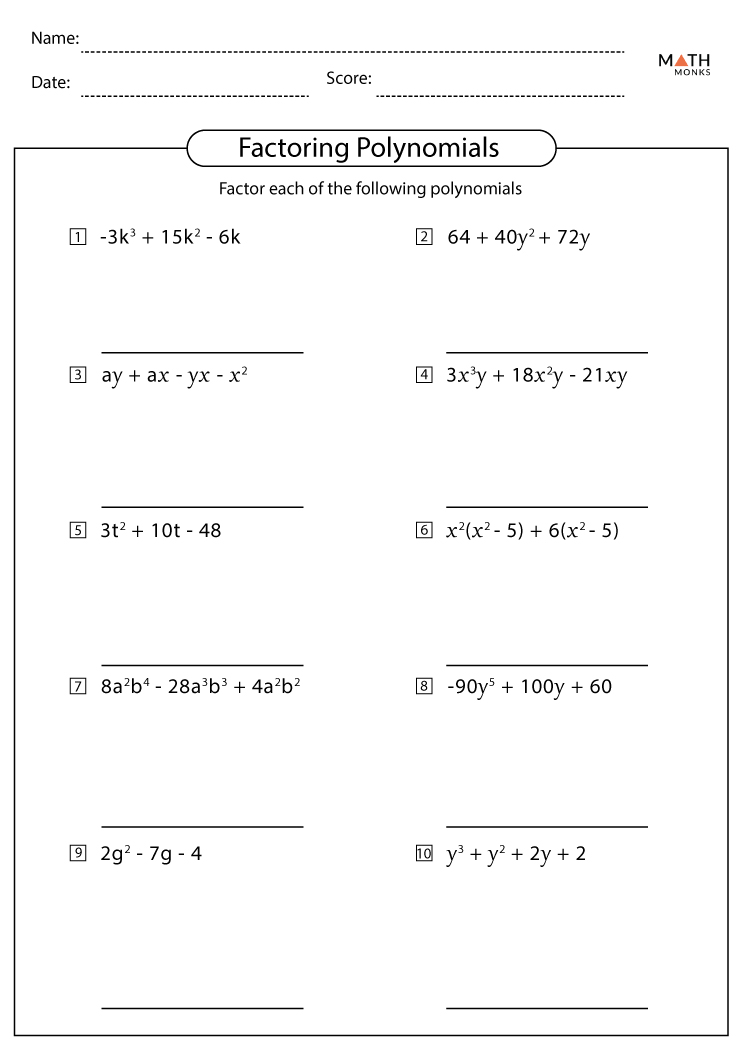
When you encounter a binomial that can be expressed as the difference of squares, the formula a^2 - b^2 = (a + b)(a - b) comes in handy:
- Identify the two terms that are being squared.
- Use the formula directly to factor the expression.
Here’s an example:
| Original Polynomial | Factored Form |
|---|---|
| x2 - 16 | (x + 4)(x - 4) |
💡 Note: Be aware that the difference of squares does not apply to a sum of squares, as it does not factor further in the real numbers.
Tip 3: Factor out Perfect Square Trinomials

Perfect square trinomials follow a recognizable pattern. If you recognize one, factoring becomes straightforward:
- Check if the first and last terms are perfect squares.
- Then, check if the middle term is twice the product of the square roots of the first and last terms.
Here’s an example:
| Original Polynomial | Factored Form |
|---|---|
| 4x2 + 12x + 9 | (2x + 3)2 |
📝 Note: Remember, not all trinomials are perfect squares, so it's important to check for this pattern first.
Tip 4: Grouping and Factoring by Grouping

This technique is particularly useful when factoring four-term polynomials:
- Group the terms into two pairs.
- Factor out the GCF from each pair.
- If the terms in parentheses are the same, factor that out as well.
Here’s an example:
| Original Polynomial | Factored Form |
|---|---|
| ab + ac + 2b + 2c | (a + 2)(b + c) |
👀 Note: Factoring by grouping can be time-consuming, but it's a crucial skill when dealing with polynomials that don’t fit into simpler patterns.
Tip 5: Practice Identifying Factorable Forms

Familiarity with common factorable forms will significantly improve your factoring speed:
- Get accustomed to recognizing patterns like trinomial factoring, difference of squares, or sum/difference of cubes.
- Practice recognizing these forms through worksheets and exercises.
🧐 Note: The more you practice, the easier it becomes to spot and remember these patterns, reducing your time spent on factoring.
In conclusion, factoring polynomials is an intricate yet rewarding part of algebra. By mastering these five tips—starting with the GCF, utilizing the difference of squares, recognizing perfect square trinomials, employing grouping strategies, and honing pattern recognition—you'll navigate the landscape of algebra with confidence. Remember, proficiency in factoring not only aids in solving equations but also deepens your understanding of how variables and numbers interact, setting a solid foundation for your mathematical journey ahead.
What is the difference between factoring and expanding polynomials?

+
Factoring breaks down a polynomial into its factors, reducing it to simpler terms, while expanding involves multiplying out these factors to get the original polynomial.
How do I know when a polynomial is completely factored?

+
A polynomial is completely factored when all its factors cannot be factored any further, are prime over the integers, and the product of the factors equals the original polynomial.
Can a polynomial with a degree greater than 2 always be factored?

+
Not all polynomials with a degree greater than 2 can be factored. Some require methods beyond simple factoring, like the use of the Rational Root Theorem, or may not have rational solutions at all.