Exponents Review Worksheet: Your Ultimate Study Guide

In the world of mathematics, understanding exponents is pivotal. These tiny superscript numbers wield immense power, simplifying complex calculations and providing a shorthand for large or small numbers. Whether you're revisiting algebra, preparing for standardized tests, or enhancing your math skills, this comprehensive guide will serve as your ultimate study tool for mastering exponents. Here, we'll explore the fundamentals, the rules, and practical applications to ensure you're thoroughly equipped.
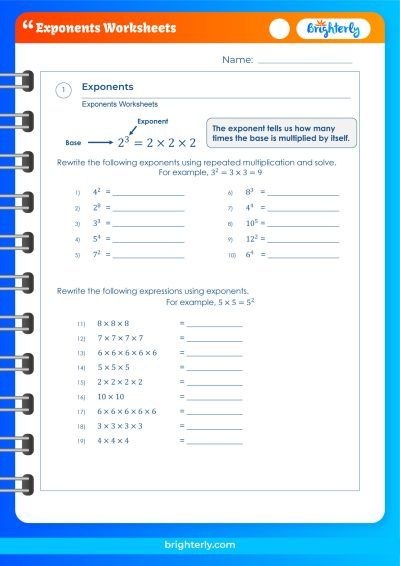
What Are Exponents?

An exponent, often referred to as a power, indicates how many times a base number is multiplied by itself. The base is the number being raised to a power, while the exponent tells you how many times to use the base in multiplication. This relationship is expressed as:
- Base: The number that you are multiplying
- Exponent: How many times you multiply the base by itself
For example, in 34, 3 is the base, and 4 is the exponent, meaning you multiply 3 by itself 4 times: 3 × 3 × 3 × 3 = 81.
Basic Rules of Exponents

Understanding the rules of exponents simplifies exponential expressions and helps in solving equations:
- Product Rule: am * an = am+n
- Quotient Rule: am / an = am-n, where a ≠ 0
- Power Rule: (am)n = amn
- Zero Exponent Rule: a0 = 1, where a ≠ 0
- Negative Exponent Rule: a-n = 1 / an, where a ≠ 0
- Exponents of One: a1 = a
🔥 Note: Exponents and their rules are not just theoretical; they have real-world applications in physics, finance, and computer science!
Examples and Exercises

To solidify your understanding, let's delve into some examples:
Simplifying Exponential Expressions
- Example: Simplify 23 * 25
- Using the Product Rule: 23 + 5 = 28 = 256
- Example: Simplify (32)4
- Using the Power Rule: 32*4 = 38 = 6,561
Exponents in Real Life

Exponents are not just an academic exercise; they are fundamental in various real-world applications:
- Compound Interest: The formula for compound interest involves exponents to calculate future value.
- Population Growth: Exponential growth models help predict population sizes.
- Physics: The distance traveled by a falling object is described by an exponential function.
Understanding how exponents work in these contexts can provide deeper insights into the mathematical modeling of natural phenomena.
Negative and Fractional Exponents

The scope of exponents extends beyond positive integers:
- Negative Exponents: Indicate division rather than multiplication. For instance, 2-3 means 1 / 23 = 1 / 8.
- Fractional Exponents: Represent roots and square roots. For example, 21/2 = √2.
Exponent Properties and Laws

Exponents follow specific properties and laws that make complex operations manageable:
| Property | Description |
|---|---|
| Addition of Exponents | When bases are the same, am + an = am+n |
| Subtraction of Exponents | When bases are the same, am - an = am-n |
| Multiplication of Exponents | (am)n = amn |
| Division of Exponents | am / an = am-n |

These properties form the backbone of operations involving exponents, ensuring consistency and accuracy in calculations.
In our journey to understanding exponents, we've explored their rules, practical applications, and how they extend to real-life scenarios. This guide not only provides you with the tools to excel in mathematics but also helps in developing a profound appreciation for how mathematical concepts are interwoven with our daily lives. Whether simplifying complex expressions, calculating exponential growth, or understanding physical laws, exponents are indeed a powerful tool in the mathematical arsenal.
What is an exponent?

+
An exponent is a number that indicates how many times a base number is multiplied by itself. It’s a shorthand for repeated multiplication.
How do I simplify exponents?

+
Exponents can be simplified using rules such as the Product Rule, Quotient Rule, and Power Rule. For example, to simplify 23 * 25, you add the exponents to get 28.
What are the real-life applications of exponents?

+
Exponents are used in finance (calculating interest), physics (modeling growth and decay), engineering (signal processing), and many more fields where quantities need to be scaled exponentially.