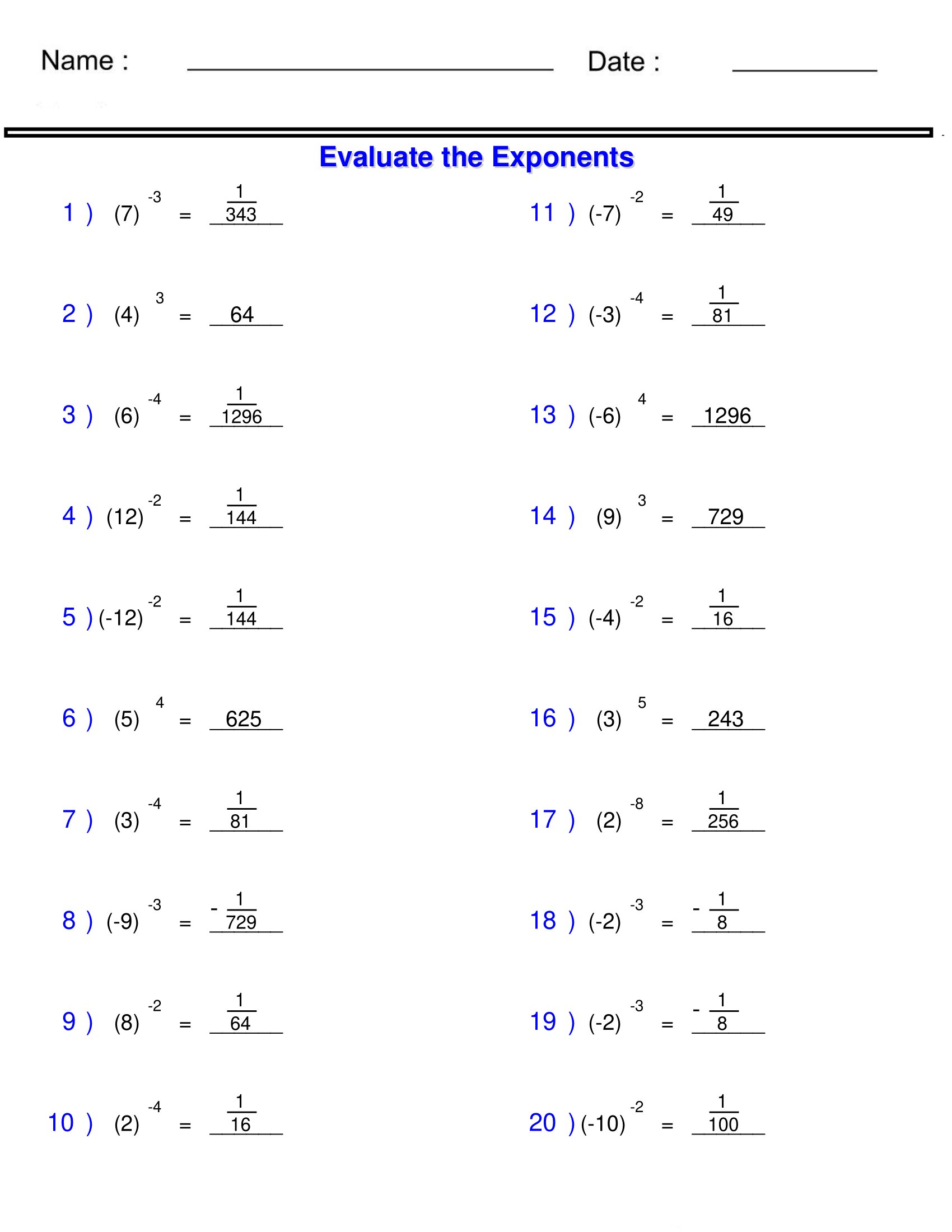
7 Essential Exponents and Radicals Worksheet Tips

Understanding exponents and radicals is pivotal for mastering mathematics, whether you're a student navigating through algebra or calculus, or someone interested in data analysis, engineering, or even financial planning. These mathematical tools are fundamental in dealing with large numbers or fractions, simplifying expressions, and solving complex equations. Here are seven essential tips to enhance your proficiency in working with exponents and radicals:
1. Master the Basics

Before diving deep into complex problems, ensure your foundation is solid:
- Exponents: Remember that a^n = a \times a \times \cdots \times a (n times). Understand positive and negative exponents, zero exponents, and how they affect the value of the base.
- Radicals: Know the properties of square roots, cube roots, and nth roots. Remember that \sqrt[n]{a} = a^{1/n} and the various rules for simplification.
💡 Note: Revisiting fundamental rules can clarify many complex problems.
2. Practice with Real Numbers

Exponents and radicals often make more sense when dealing with real numbers:
- Try simplifying expressions like 2^4 or \sqrt[3]{8}.
- Work on converting between exponential and radical forms. For example, 5^{1/3} and \sqrt[3]{5}.
Real number examples help ground abstract concepts in something tangible.

3. Leverage the Power of Patterns
Mathematics loves patterns, and so should you:
- Examine sequences like powers of 2, 3, or 10 to understand exponential growth.
- Explore patterns in roots, such as \sqrt{1} = 1, \sqrt{4} = 2, \sqrt{9} = 3, and so on.
Seeing these patterns helps in recognizing when to use specific techniques for simplification.
4. Understand the Relationship Between Exponents and Radicals

Exponents and radicals are two sides of the same coin:
- The key is recognizing that a^{1/n} = \sqrt[n]{a} and vice versa.
- Learning how to manipulate exponents to work with radicals, and vice versa, simplifies problem-solving.
| Exponent Form | Radical Form | Equivalent Expression |
|---|---|---|
| 2^{1/2} | \sqrt{2} | 1.414... |
| 8^{1/3} | \sqrt[3]{8} | 2 |

5. Get Comfortable with Negative and Fractional Exponents

These are not to be feared:
- Negative Exponents: Remember a^{-n} = \frac{1}{a^n}.
- Fractional Exponents: a^{m/n} = (a^{1/n})^m or a^{m/n} = (a^m)^{1/n}.
Both negative and fractional exponents are just tools to simplify expressions, so practice converting between forms regularly.
6. Use Real-World Applications

Mathematics becomes more relevant when linked to real life:
- Use exponents to calculate compound interest: A = P(1 + \frac{r}{n})^{nt}.
- Understand how radicals can model real-world problems like calculating the area of a sector or the length of an arc.
Applying concepts to real scenarios can deepen understanding and interest in the topic.
7. Solve Problems Step by Step

Mathematics, particularly with exponents and radicals, thrives on a systematic approach:
- Start with the innermost operations and work your way outwards.
- Keep track of your steps, especially when simplifying radicals, as each step can affect the final answer.
🗝️ Note: Detailed steps reduce errors and confusion in complex problems.
In summary, mastering exponents and radicals involves understanding their rules, practicing with real numbers, recognizing patterns, understanding their relationship, getting comfortable with different exponent forms, applying concepts to real-world scenarios, and methodically solving problems. These tips are not just for worksheets but for any mathematical endeavor involving these operations. With practice and perseverance, you'll find your proficiency in dealing with exponents and radicals improving, opening doors to advanced mathematics and its applications.
Why are exponents and radicals important in mathematics?

+
Exponents and radicals are essential for simplifying expressions, solving equations, and dealing with large numbers or fractions. They are the foundation for understanding advanced topics like logarithms, exponential functions, and calculus.
How can I remember the properties of exponents?

+
Utilize mnemonic devices, practice regularly, and try to understand the logic behind the rules. For example, the power of a power rule (( (a^m)^n = a^{mn} )) can be visualized as “the exponent multiplies exponents.”
What’s the best way to tackle worksheet problems with exponents and radicals?

+
Approach each problem methodically, simplify expressions step by step, ensure you understand each rule you apply, and review your answers by redoing the problem or using a different method to verify your solution.