Master Exponential Growth and Decay with This Worksheet

Understanding exponential growth and decay is fundamental in various fields including finance, physics, and biology. Whether you're predicting population growth, analyzing the depreciation of an asset, or studying radioactive decay, mastering these concepts can significantly enhance your analytical capabilities. In this post, we'll delve deep into the nuances of exponential functions, providing you with practical tools and insights through an interactive worksheet. By the end of this guide, you'll be equipped with the knowledge to apply exponential growth and decay models effectively.
Understanding Exponential Functions

Exponential functions come in two main forms:
- Exponential Growth: This occurs when a quantity increases at a consistent rate over time. The mathematical form is ( y = a \cdot e^{kx} ), where:
- a is the initial amount
- e is Euler’s number (approximately 2.71828)
- k is the growth rate
- x represents time
- Exponential Decay: Here, a quantity decreases at a consistent rate. The function looks like ( y = a \cdot e^{-kx} ), with the same variables as above but note the negative sign in the exponent for decay.
🔍 Note: The base e is used to represent natural exponential functions, but you might also see bases like 2 or 10 in different contexts.
Formulating Your Worksheet

To actively engage with these concepts, let’s create a worksheet that will help you understand:
1. Setting Up Your Worksheet

- Choose Your Scenarios: Select contexts where exponential growth or decay occurs. Examples might include:
- Population growth of a species
- Radioactive decay
- Investment growth
- Identify Variables: List the parameters like initial value, growth/decay rate, and time period. For example:
Scenario Initial Value (a) Growth/Decay Rate (k) Time (x) Investment Growth $1000 0.05 5 years Radioactive Decay 10g -0.693 3 hours 
- Formulate the Equations: Write the appropriate exponential function for each scenario.
2. Application of the Formulas

Now let’s apply these formulas to solve problems:
- Calculate Future Values: For the investment scenario, if you invest $1000 with a 5% annual growth rate, how much will you have after 5 years? Use the formula: [ y = 1000 \cdot e^{0.05 \cdot 5} \approx 1284.03]
- Determine Half-Life: In radioactive decay, how long does it take for half the initial mass to remain? If the decay constant is -0.693, then: [ t_{1⁄2} = \frac{\ln(2)}{-0.693} \approx 1 \text{ hour} ]
📊 Note: For exponential decay, the negative sign in the exponent indicates the rate of reduction over time.
Visualizing Growth and Decay
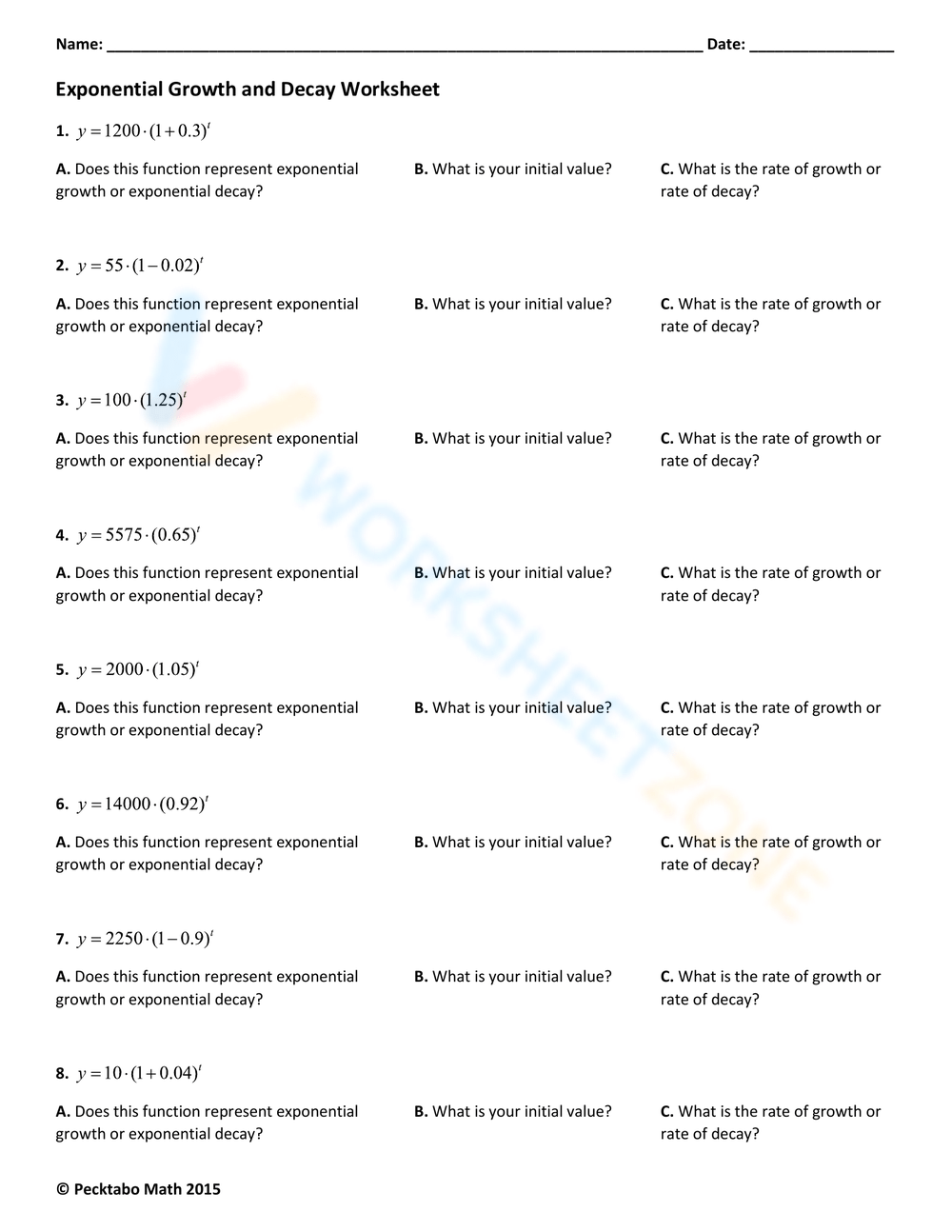
Creating visual representations can greatly aid in understanding:
- Graphing Functions: Use graphing tools to plot exponential functions. This helps in seeing how the value changes over time and comparing different growth/decay rates.
- Understanding Slopes: The slope of an exponential function increases (growth) or decreases (decay) at an accelerating rate. This is evident from the increasing or decreasing gradient on the graph.
Real-World Applications
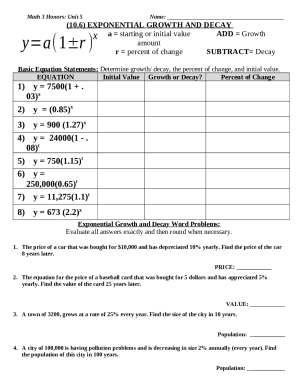
Let’s look at some practical applications:
- Finance: Exponential functions are used to predict investment growth or compound interest, helping in planning for future financial needs.
- Physics: Radioactive decay modeling is crucial for understanding and applying principles like half-life in nuclear physics.
- Biology: Growth models help in predicting population dynamics, understanding spread of diseases, or bacterial growth.
💡 Note: Exponential models are simplifications; real-world scenarios might include factors like carrying capacity in population growth or other decay processes that are not purely exponential.
In our journey through exponential growth and decay, we’ve navigated from theoretical foundations to practical applications. By using a worksheet to engage actively with these concepts, we’ve not only learned how to calculate future values or decay rates but also how to visualize and interpret these models in real-world scenarios. This understanding equips us to approach problems in finance, physics, biology, and beyond with a clearer lens, enhancing our decision-making and predictive capabilities. Remember, the key to mastering exponential functions lies in consistent practice and understanding the underlying mathematical principles, which will guide you in applying these concepts accurately in diverse situations.
What is the difference between growth and decay in exponential functions?
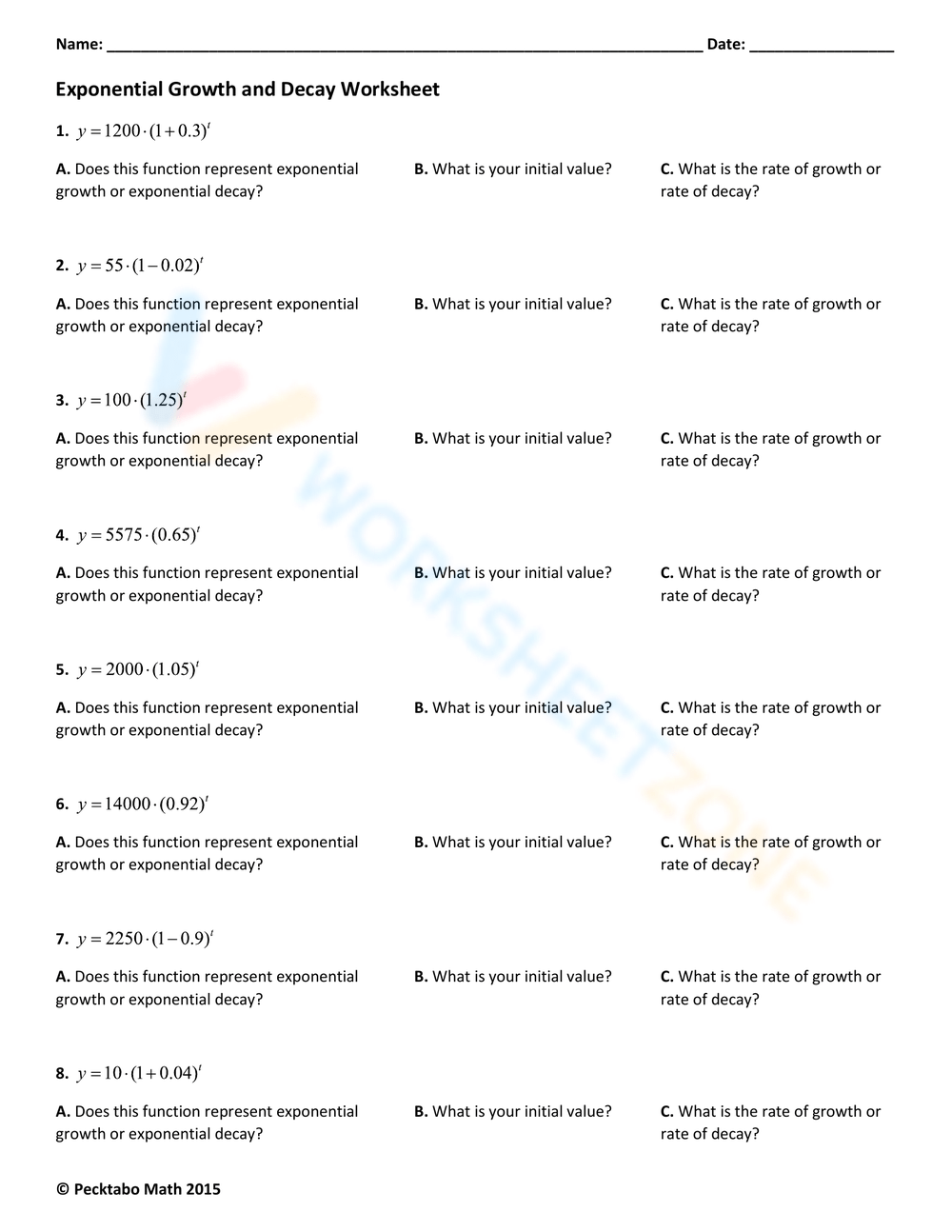
+
Exponential growth increases over time at a consistent rate, represented by a positive exponent in the function. Exponential decay, on the other hand, decreases over time at a consistent rate, shown by a negative exponent.
How can I find the half-life of a substance?

+
The half-life (t{1⁄2}) of a substance can be found using the formula ( t{1⁄2} = \frac{\ln(2)}{-k} ), where k is the decay constant.
Why do we use base e in exponential functions?

+
Base e (Euler’s number) is used because it makes many mathematical calculations simpler, particularly in calculus. It’s the base where the derivative of the natural logarithm function is equal to 1/x.
Can exponential functions model everything in nature?

+
While exponential functions are powerful, they are not universally applicable. Many natural processes require more complex models that account for environmental factors, interference, or other non-linear dynamics.