Master Trigonometric Functions with Our Easy-to-Follow Worksheet

The allure of trigonometry lies not only in its ancient roots but also in its modern applications. From determining the height of a pyramid to designing a bridge's curve, the practical uses of trigonometry are vast. If you're looking to master trigonometric functions, you'll need an easy-to-follow worksheet that simplifies the process, making learning efficient and practical.
Understanding Trigonometric Functions


At the heart of trigonometry are six fundamental functions: sine, cosine, tangent, cosecant, secant, and cotangent. These functions are the building blocks of a plethora of mathematical and real-world problems. Here’s a brief introduction to each:
- Sine (sin): The ratio of the length of the side opposite to the angle in question to the hypotenuse.
- Cosine (cos): The ratio of the adjacent side’s length to the hypotenuse.
- Tangent (tan): The ratio of the length of the opposite side to the adjacent side.
- Cosecant (csc): The reciprocal of sine.
- Secant (sec): The reciprocal of cosine.
- Cotangent (cot): The reciprocal of tangent.
📝 Note: Remember that these trigonometric functions also have specific values at key angles like 0°, 30°, 45°, 60°, and 90°.
Worksheet Structure


A well-structured worksheet can be a fantastic tool in mastering trigonometry. Here are the elements a worksheet should include:
- Basics Recap: Quick review of definitions and primary functions to kickstart your memory.
- Problem Sets: Assorted levels of difficulty to progressively challenge your skills.
- Graphing Exercises: Plotting graphs to visually comprehend trigonometric functions.
- Real-world Applications: Problems that translate theory into practical scenarios.
- Solutions Section: Worked examples to cross-reference your answers.
- Key Concepts: Summarize vital identities and formulas for easy reference.
Step-by-Step Guide to Using the Worksheet

Begin with Basics

Start your learning journey by going over the basic definitions and properties:
- Understand the unit circle and the related angles’ coordinates.
- Familiarize yourself with the trigonometric table for key values.
- Explore the relationships between sine, cosine, and tangent through right triangles.
Engage with Problem Sets
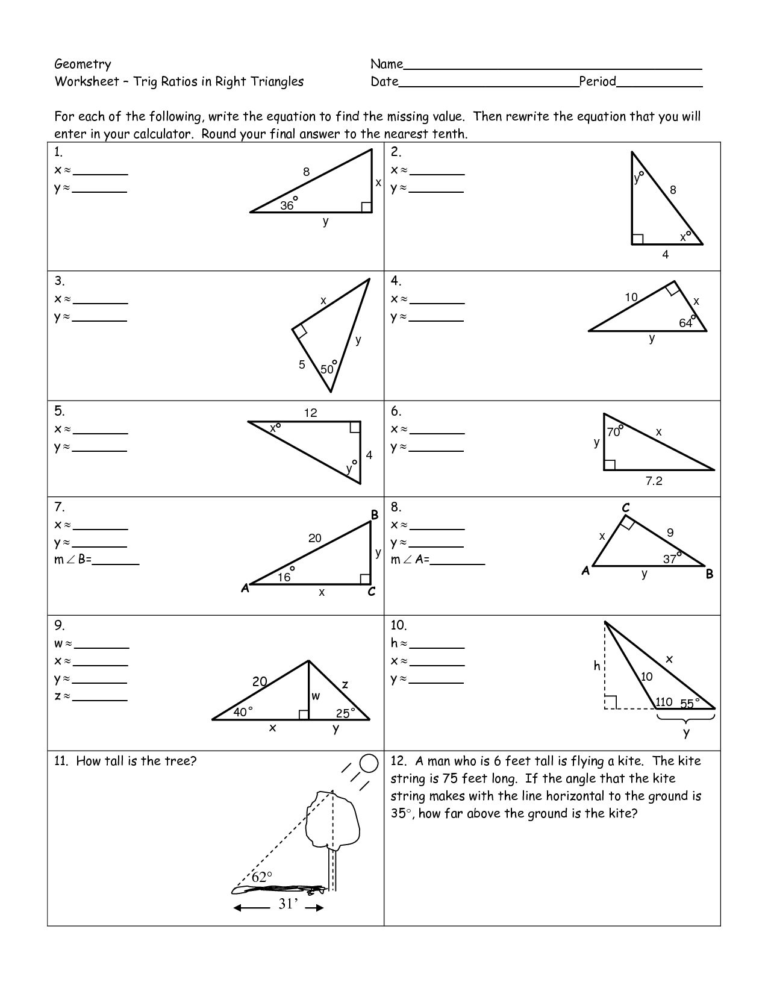
As you move forward:
- Try solving problems from basic trigonometry to more complex scenarios.
- Use the provided angles to calculate trigonometric values or solve for unknown angles.
- Employ the Pythagorean theorem where necessary, especially in right triangle problems.
Plot and Visualize

To deepen your understanding:
- Plot sine, cosine, and tangent on a Cartesian coordinate system.
- Examine how these functions behave over different periods.
- Understand the concepts of amplitude, phase shift, and vertical shift.
📝 Note: Graphing helps visualize the periodicity and symmetry of trigonometric functions, making abstract concepts more tangible.
Real-World Connection

Practical examples in your worksheet could be:
- Determining the optimal trajectory for a projectile.
- Calculating the necessary angles for satellite communication.
- Estimating the amount of paint needed for an oddly shaped wall.
Check Your Work

Lastly:
- Compare your answers with the solutions provided.
- Identify where you went right or where there might be a misunderstanding.
- Revisit key formulas and identities if needed to correct your approach.
As we wrap up, let's not forget the importance of application in truly understanding trigonometry. The principles you've learned are not only essential for passing exams but also for solving real-life problems. They open the door to fields like engineering, physics, and even architecture. Mastering trigonometric functions with our easy-to-follow worksheet isn't just about memorizing formulas; it's about applying these principles to real-world scenarios, fostering a deeper, more intuitive understanding of trigonometry's power.
How often should I practice trigonometry to become proficient?

+
Regular practice is key. Aim for at least 15-30 minutes daily to reinforce your understanding of trigonometry and build muscle memory with formulas and problem-solving techniques.
Can trigonometry worksheets be used to prepare for a physics course?

+
Yes, understanding trigonometry is crucial in physics, particularly in kinematics, dynamics, and periodic motion. The worksheet will help you with vector analysis, projectile motion, and oscillations.
What are the benefits of including graphing exercises in trigonometry worksheets?

+
Graphing exercises provide visual representations of trigonometric functions, helping learners to understand periodicity, amplitude, phase shifts, and symmetry. This visual learning aids in memory retention and conceptual understanding.
Do I need to know calculus to understand trigonometry fully?

+
Not necessarily for basic to intermediate trigonometry. However, for a deeper understanding of trigonometric functions, especially in their derivatives and integrals, calculus can be extremely beneficial.
Is trigonometry still relevant in today’s technology-driven world?

+
Definitely! Trigonometry underpins many modern applications like GPS, computer graphics, VR/AR technologies, architecture, and more, demonstrating its enduring relevance.



