Evaluating Functions Made Simple: Comprehensive Worksheet Guide

Welcome to our comprehensive guide on evaluating functions, where we'll dive into the methods, principles, and practical applications of this fundamental mathematical concept. Whether you're a student looking to master your algebra skills, an educator seeking resources to explain functions effectively, or someone curious about how functions work in real-world scenarios, this post is designed to clarify the process of evaluating functions step-by-step.
What are Functions?
At its core, a function is a mathematical relationship between two sets, called the domain and the range. It assigns each element of the domain to exactly one element in the range. Functions can be represented in various ways:
- Graphically - as curves on a coordinate plane
- Tabularly - in a table of values
- Algebraically - through equations
- Verbally - described in words
The Basics of Evaluating Functions
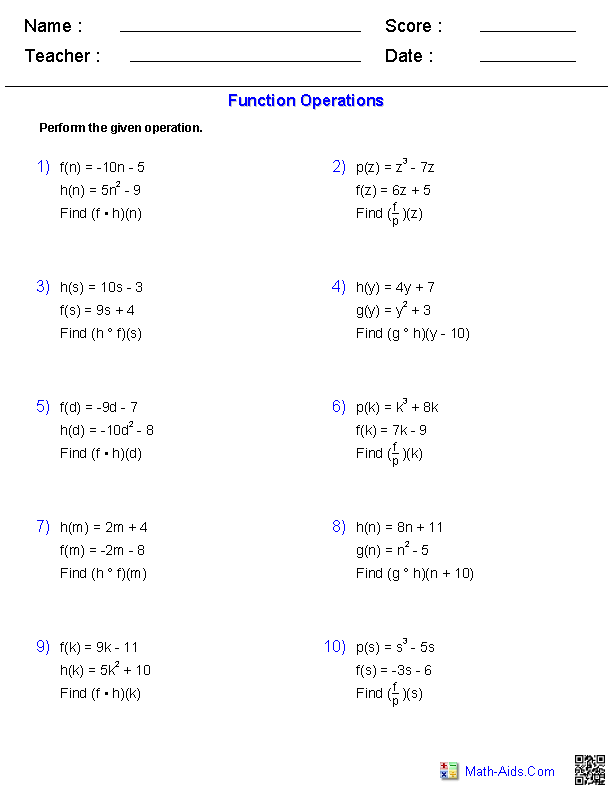
Evaluating a function involves substituting a value or an expression into the function in place of its variable(s) and then simplifying the expression. Here’s a quick look at how it works:
- If f(x) is your function and you need to find f(3), replace every x in the function with 3 and solve.
Let's take an example:
f(x) = 2x + 3
- To evaluate f(3), substitute 3 for x:
f(3) = 2(3) + 3 = 6 + 3 = 9
Why We Evaluate Functions
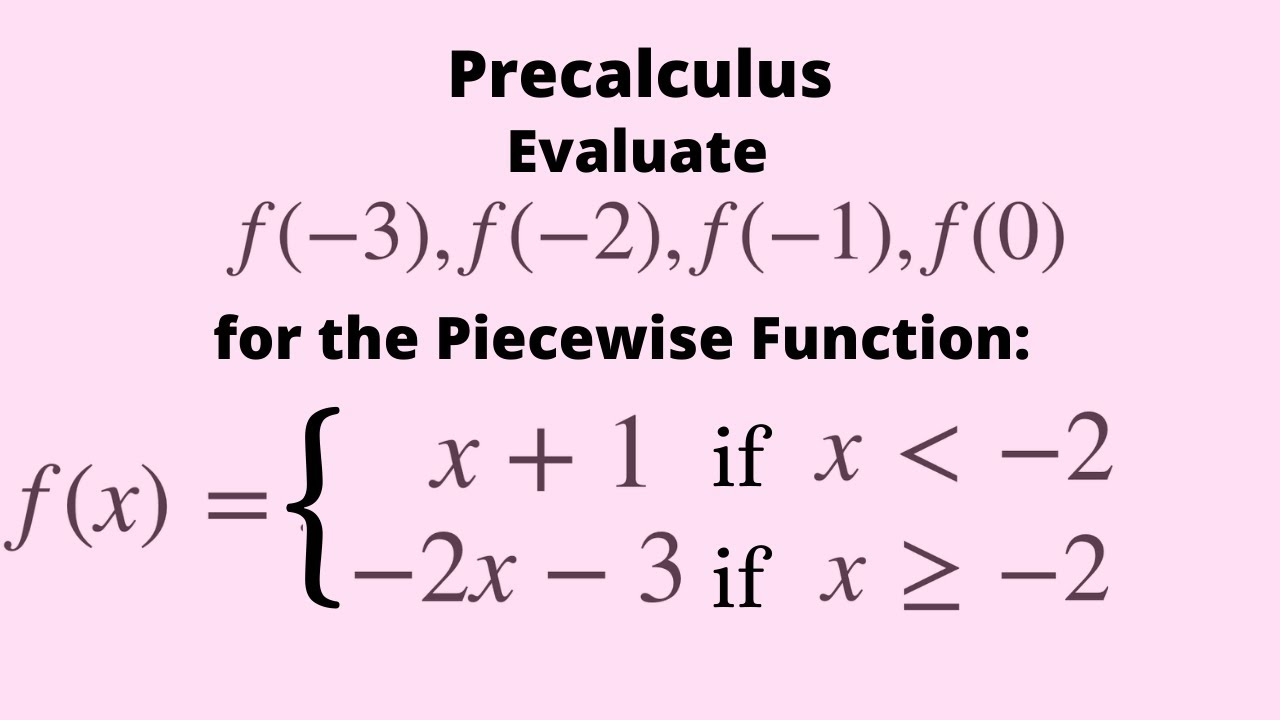
Evaluating functions helps in:
- Understanding relationships between variables
- Modeling real-life scenarios
- Preparing for calculus and higher mathematics
Step-by-Step Guide to Evaluating Functions
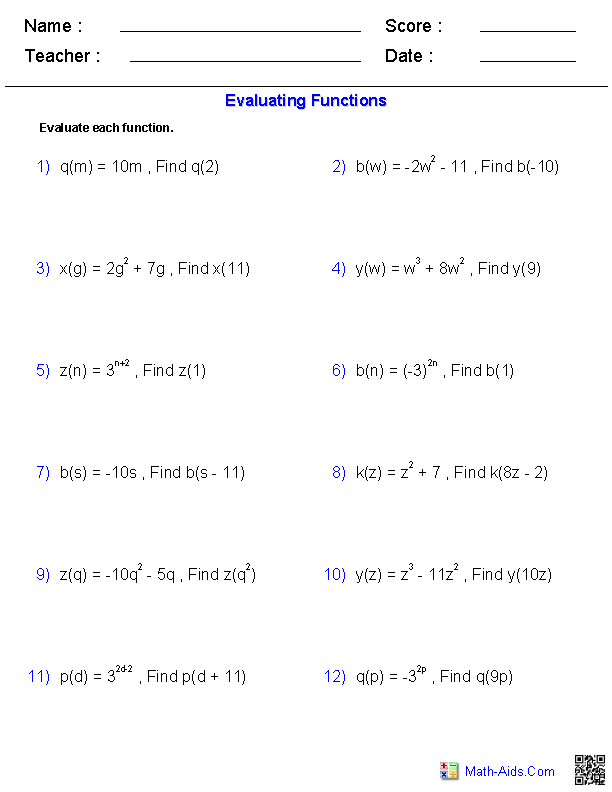
1. Identify the Function

Start with the given function. Let’s use:
f(x) = x^2 - 2x + 1
2. Determine the Input Value

If you’re asked to find f(a), ‘a’ is your input value.
3. Substitute the Input

Replace every x in the function with a:
f(a) = a^2 - 2a + 1
4. Simplify the Expression

Calculate the result:
f(a) = a^2 - 2a + 1 = a(a-2) + 1
(Here, we used factoring for simplicity, which might not always be necessary but can be useful for understanding the function’s behavior.)
5. Interpret the Result

Understand what your result represents in terms of the function’s properties:
- Is it a maximum or minimum value?
- Does it intersect with the x-axis or y-axis?
Evaluating Complex Functions
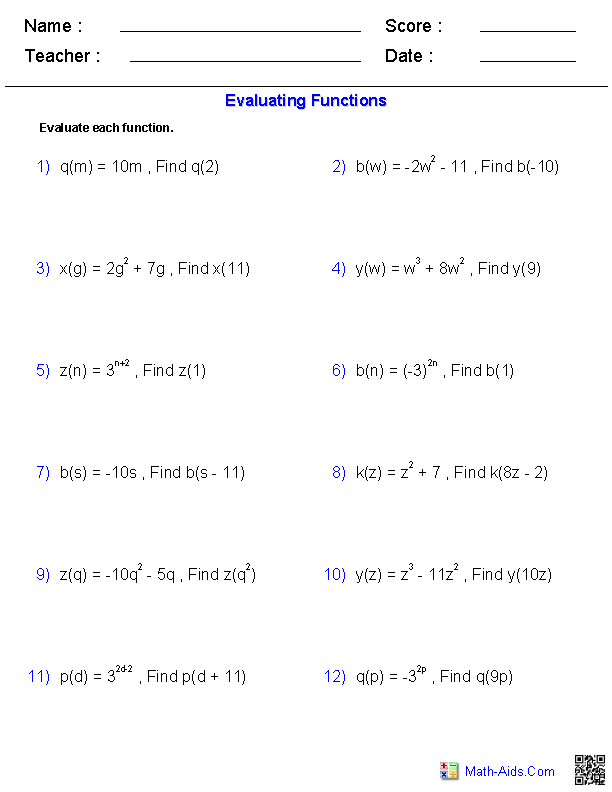
Functions with Multiple Variables

When dealing with functions like g(x, y), you’ll substitute values for each variable separately:
g(x, y) = 3x + 2y^2 - x/y
Functions with Composition
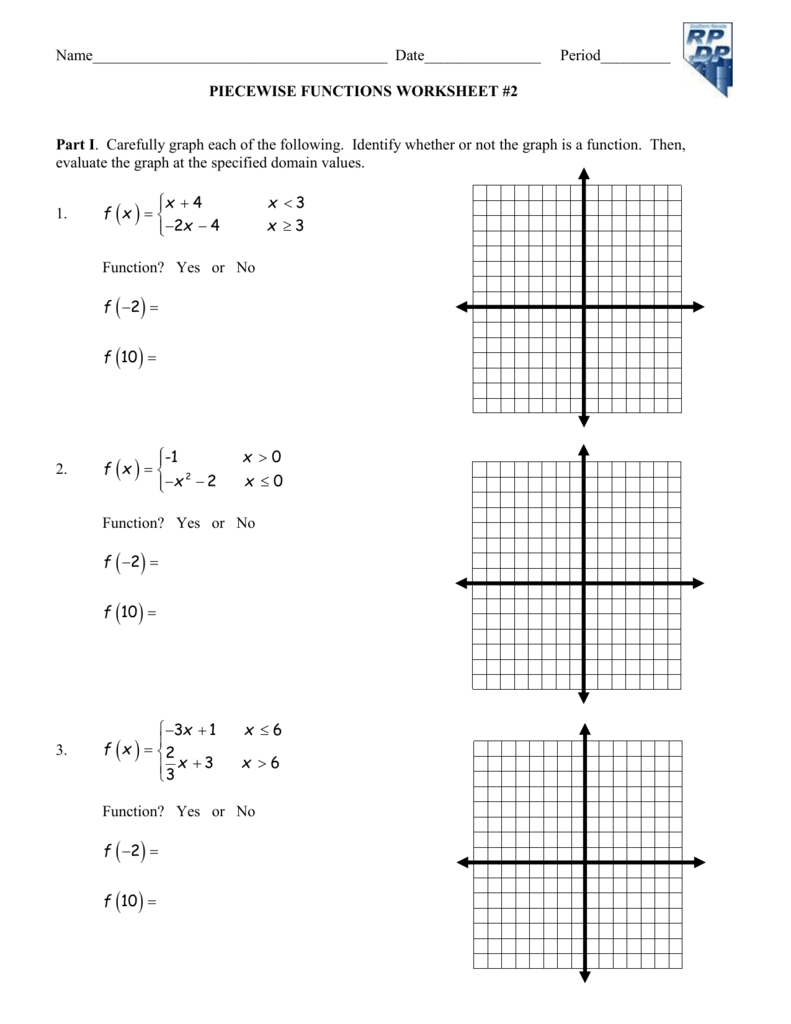
If you’re evaluating f(g(x)), you first evaluate g(x), then substitute its value into f:
- Let g(x) = x + 2 and f(x) = x^2
- To evaluate f(g(3)):
- Find g(3) = 3 + 2 = 5
- Then f(5) = 5^2 = 25
Using a Worksheet for Practice
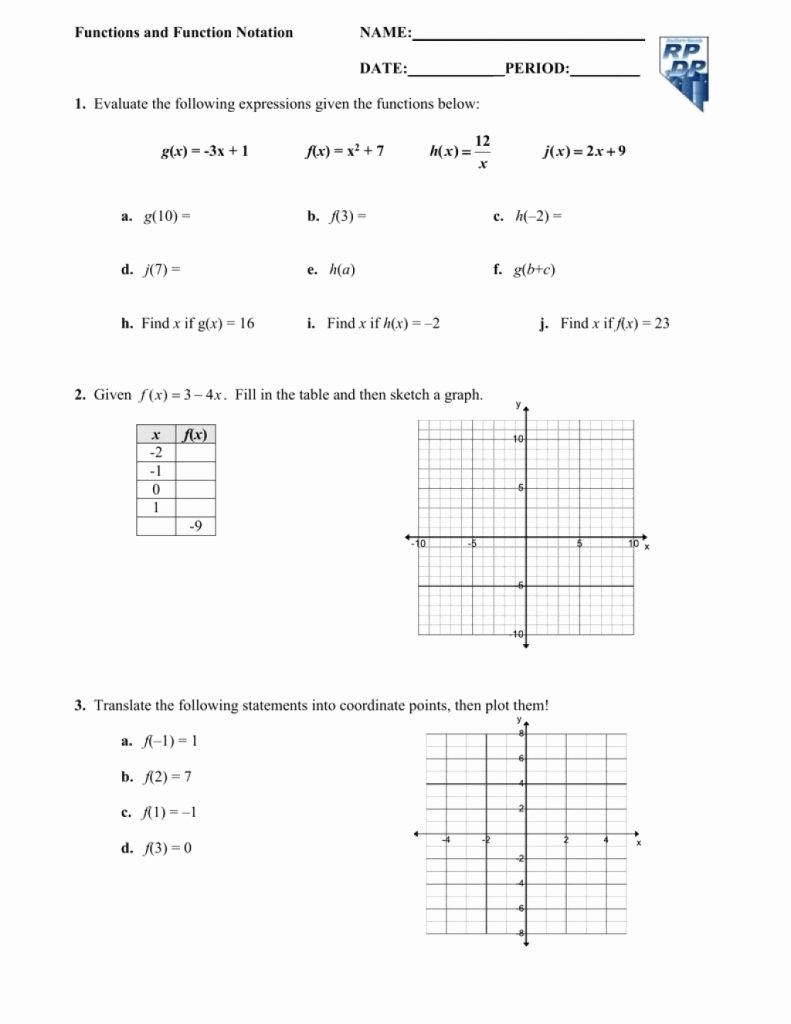
A worksheet can be an excellent tool for practicing function evaluation. Here’s how to set up and use one:
- Define the Function: Write the function at the top of your worksheet.
- Provide Input Values: List a set of values or expressions to evaluate.
- Evaluation Space: Leave space for students to work out each problem.
Here’s an example table to structure your worksheet:
| Function | Input | Evaluation |
|---|---|---|
| f(x) = 3x - 2 | 5 | |
| f(x) = 2x + 3 | -4 | |
| f(x) = x^2 - 4 | 3 |

📝 Note: A well-structured worksheet should provide a mix of linear, quadratic, and more complex functions to ensure thorough understanding and practice.
Summary

In this guide, we’ve covered the essentials of evaluating functions. From understanding what functions are to the practical steps of substitution and simplification, you now have the tools to tackle function evaluations in various mathematical and real-world contexts. Remember, the key to mastering this skill lies in consistent practice, ensuring you understand not just how to evaluate but why you are evaluating functions. Whether you’re solving simple linear functions or dealing with more complex compositions, the method remains consistent - identify, substitute, simplify, and interpret.
Why do we need to evaluate functions?

+
We evaluate functions to understand how changes in one variable affect another. This is crucial for problem-solving in various fields, from engineering to economics.
How do I know when to use functions?

+
Functions are used whenever there is a relationship between variables. Look for scenarios where one variable depends on another or when you need to model data.
What’s the difference between evaluating a function and solving an equation?
+Evaluating a function involves finding the value of the function for a given input, whereas solving an equation finds the value of the variable that makes the equation true.