Master Graph Functions with Ease: Evaluation Worksheet Guide
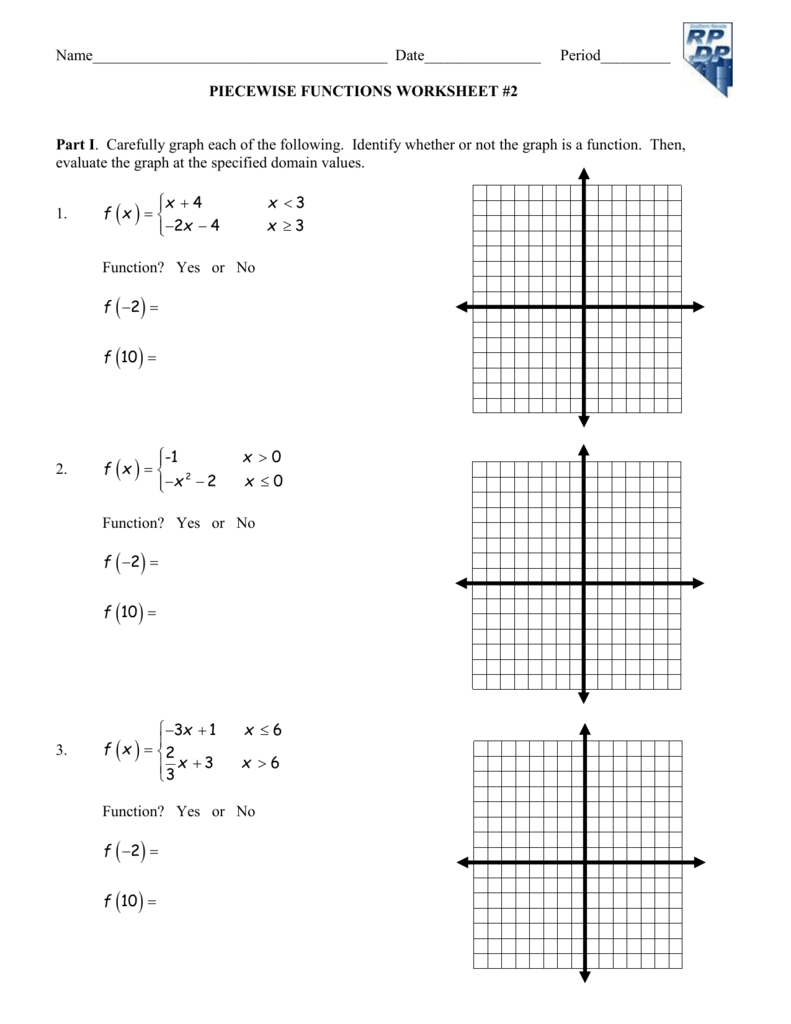
Graphing functions isn't just for mathematicians; it's an invaluable skill for anyone involved in data analysis, engineering, statistics, or even economics. Understanding how to evaluate functions, identify key features like domain and range, and solve complex problems involving these functions can empower you to analyze and interpret data more effectively. This comprehensive guide will walk you through an evaluation worksheet tailored to mastering graph functions, with steps, examples, and tips to help you excel.
Understanding Function Graphs
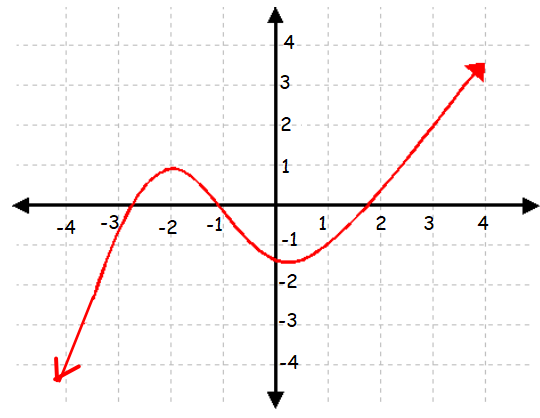

At the heart of graphing functions is the ability to understand what you're looking at. Here are the core concepts:
- Domain: The set of all possible input values (x) for which the function produces a defined output (y).
- Range: The set of all possible output values (y) that the function can produce.
- Asymptotes: Lines that a function approaches but never touches, often indicating limits of the function's behavior.
Key Features of Function Graphs

Understanding the following features will help you interpret and evaluate functions:
- Intercepts: Points where the graph crosses the x-axis (x-intercepts) or y-axis (y-intercepts).
- Symmetry: Does the graph look the same on both sides of the y-axis (even function) or does it have rotational symmetry (odd function)?
- Transformations: Understanding how shifting, reflecting, stretching, or compressing affects a function's graph.
Step-by-Step Guide to Evaluating Function Graphs

Step 1: Analyze the Domain and Range

To start, identify the domain and range:
- Determine the domain: Look for vertical asymptotes or holes where the function is undefined.
- Determine the range: Identify horizontal asymptotes, local maxima or minima, and any gaps in the graph.
Step 2: Identifying Intercepts

Intercepts provide crucial information about where the function starts or ends:
- To find x-intercepts, set y = 0 and solve for x.
- To find y-intercepts, set x = 0 and solve for y.
Step 3: Graph Analysis for Key Features
Here, you’ll delve into the graph’s key features:
- Look for asymptotes: Vertical asymptotes suggest where the function is undefined, while horizontal asymptotes indicate the function’s behavior as x approaches infinity.
- Identify symmetry: Even functions are symmetric about the y-axis, and odd functions are symmetric about the origin.
- Note any transformations: Translations, reflections, or stretches/compressions indicate how the function has changed from a base function.
Evaluating Functions with a Worksheet

Now, let's dive into using an evaluation worksheet to master graph functions:
Worksheet Preparation

Create or gather a worksheet with the following components:
- A list of functions to evaluate (polynomial, rational, trigonometric, exponential, etc.).
- Space for domain, range, intercepts, asymptotes, and transformations.
Evaluating Functions

- Plot the Graph: Use graphing software or sketch by hand, ensuring accuracy.
- Domain and Range: Use the graph to determine these.
- Intercepts: Identify x- and y-intercepts.
- Asymptotes and Symmetry: Note down any vertical, horizontal, or slant asymptotes and describe the symmetry.
- Transformations: Observe how the function deviates from its base form.
- Solve Problems: Use the graph to solve equations or interpret the function in context.
🌟 Note: Ensure to label your graph correctly and check your work for common mistakes like missing transformations or incorrect asymptotes.
Examples and Problem Solving

Let's take a look at an example:
Example Function: f(x) = 1/x


- Domain: x ≠ 0
- Range: All real numbers
- Intercepts: None
- Asymptotes: Vertical at x = 0; Horizontal at y = 0
- Symmetry: Odd function (symmetric about origin)
- Transformations: Reflection over the y-axis and a vertical stretch.
Using this information, we can solve problems like:
- Find the value of x for which f(x) = 2. (Answer: x = 0.5)
- What does the function approach as x approaches infinity? (Answer: 0, from both positive and negative sides).
Integrating Worksheet Practice
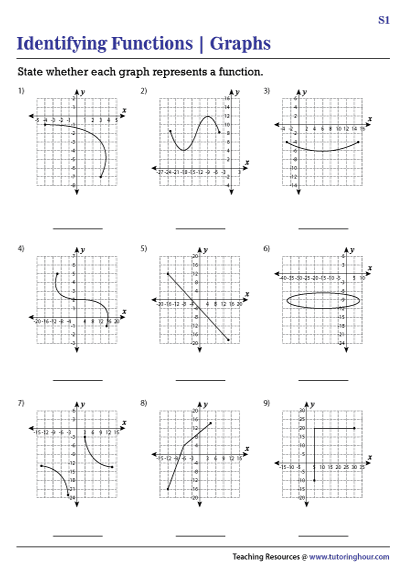
Regularly practicing with a worksheet helps:
- Sharpen Your Skills: Repetition makes graphing and evaluating functions second nature.
- Identify Weaknesses: Worksheet tasks reveal areas where you might need more focus.
⚠️ Note: Remember to verify your work, especially when dealing with complex functions. Graphing tools can complement your hand-drawn sketches for accuracy.
In wrapping up, mastering graph functions through an evaluation worksheet not only sharpens your mathematical skills but also enhances your ability to analyze and interpret data in various fields. By understanding the key features of function graphs, practicing with structured worksheets, and solving real-world problems, you equip yourself with a powerful analytical toolset.
What is the significance of understanding function graphs?

+
Understanding function graphs allows for better analysis and interpretation of data in fields like economics, engineering, physics, and computer science. It helps in visualizing relationships between variables, solving equations, and predicting outcomes.
Can you graph a function without a calculator?

+
Absolutely. While graphing calculators or software can make the task easier, hand-drawn graphs require understanding of function behavior, transformations, and key features like domain, range, and intercepts. This practice deepens your comprehension of the function.
How does symmetry relate to functions?

+
Symmetry in functions indicates specific types of even (y-axis symmetry) or odd (rotational symmetry about the origin) functions, which can simplify calculations and analysis. For instance, even functions are symmetrical, meaning f(-x) = f(x), while odd functions have f(-x) = -f(x).