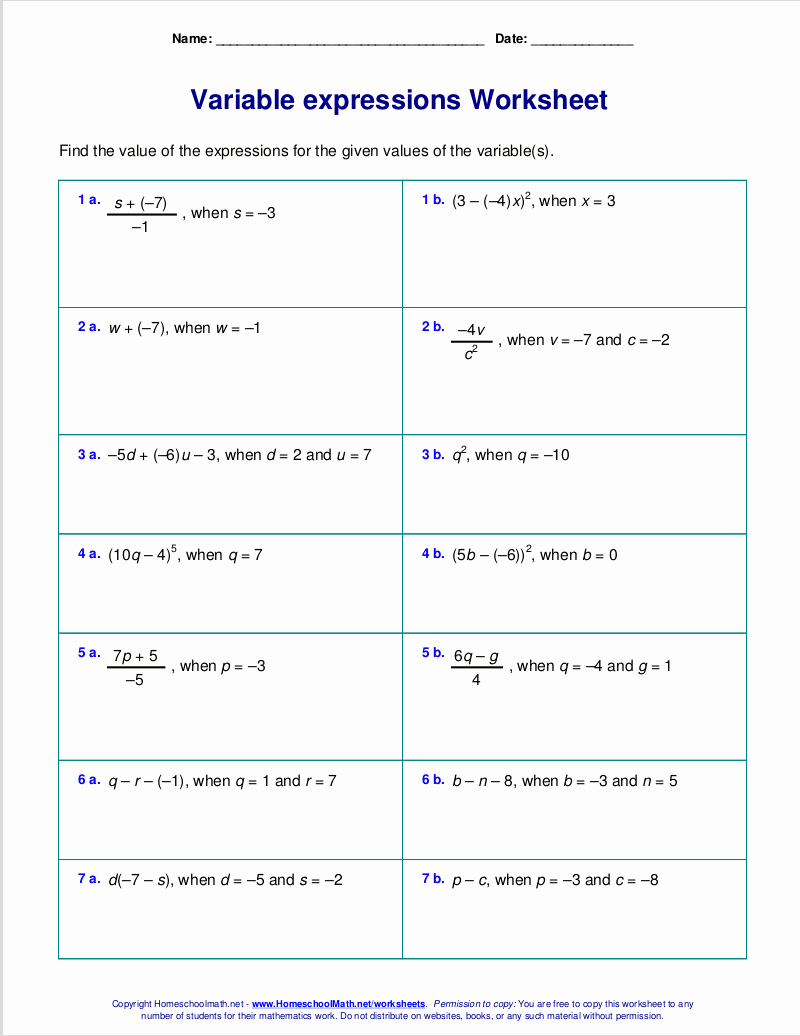
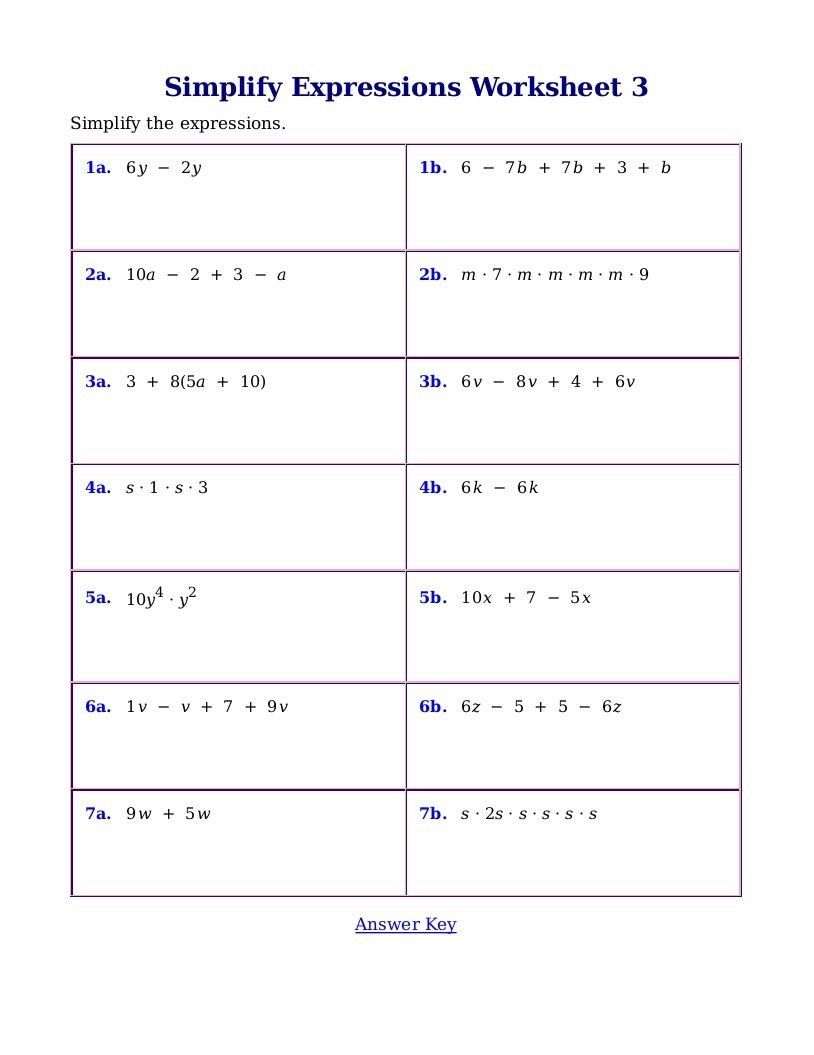
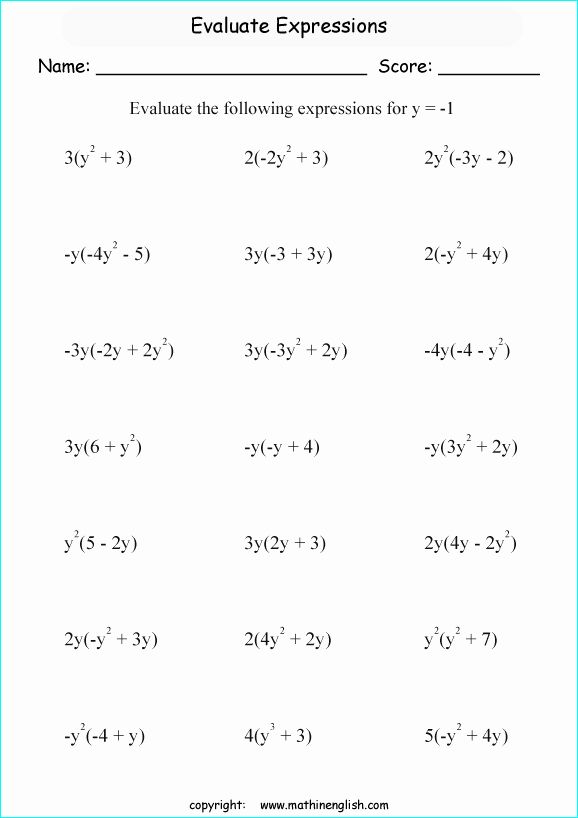
Master Math Skills: Evaluate Expressions Worksheet Guide

Understanding how to evaluate mathematical expressions is a fundamental skill for students at all levels. This guide will walk you through the process of evaluating expressions with a focus on techniques and exercises that ensure accuracy and efficiency. Whether you're preparing for a test or just looking to sharpen your math skills, this detailed exploration will equip you with the necessary tools to tackle algebraic expressions confidently.
Understanding Mathematical Expressions

At the heart of algebra lies the ability to understand and evaluate mathematical expressions. An expression is a combination of numbers, variables, and operations without an equal or inequality sign. For example, 3x + 4y - 5 is an algebraic expression where x and y are variables, and 3, 4, and 5 are constants.
The Basics of Evaluating Expressions
- Identify the order of operations: The sequence in which operations are performed can change the outcome of an expression. Here are the standard steps:
- Parentheses
- Exponents or square roots
- Multiplication and Division (from left to right)
- Addition and Subtraction (from left to right)
- Substitute values: If you're given values for variables, you substitute these into the expression before performing any operations.
Practical Examples

Simple Expression

Let’s take the expression 2x + 3y - 1 with x = 4 and y = 2:
- Substitute the values: 2(4) + 3(2) - 1
- Multiply first: 8 + 6 - 1
- Then, perform addition and subtraction: 8 + 6 = 14; 14 - 1 = 13
The result is 13.
Complex Expression

Consider the expression 3(a - b)^2 + 4, where a = 5 and b = -1:
- Substitute the values: 3((5 - (-1))^2) + 4
- Simplify inside parentheses: 3(6^2) + 4
- Evaluate the exponent: 3(36) + 4
- Perform multiplication and addition: 108 + 4 = 112
The result is 112.
Tips for Effective Evaluation

- Be systematic: Follow the order of operations without deviation.
- Double-check: After evaluating an expression, consider if the result makes sense. If the numbers seem unusually large or small, recheck your work.
- Use brackets: When substituting variables, use brackets to keep track of your operations more easily.
Common Mistakes to Avoid
| Mistake | Example | Correction |
|---|---|---|
| Ignoring the Order of Operations | 3 + 2 * 4 = 20 | Correctly: 3 + (2 * 4) = 11 |
| Not Distributing Over Parentheses | a(b - c) = ab - c | Correctly: a(b - c) = ab - ac |
| Incorrect Substitution | 5y^2 for y = 2 is not 5(2)^2 | Correctly: 5(2^2) = 5 * 4 = 20 |

🔍 Note: Be particularly careful when working with exponents, as they can drastically change the value of an expression.
Worksheet Exercises for Evaluation

Practice makes perfect. Here are some exercises to help reinforce your understanding:
Basic Substitution

- Evaluate 2x - 3 for x = 5
- Calculate y^2 + 4y + 5 for y = -3
Expressions with Multiple Operations

- Evaluate (a + b)^2 - c for a = 3, b = 4, and c = 7
- Find the value of x^3 - 2x + 5 for x = -2
🧮 Note: Practice not only aids in memorizing steps but also in understanding the behavior of mathematical expressions.
Wrapping Up

Evaluating expressions correctly requires a solid grasp of algebra basics, attention to detail, and an understanding of the logical flow of operations. By systematically following the order of operations, ensuring accurate substitution, and being aware of common pitfalls, you can develop proficiency in evaluating expressions with confidence. This guide should serve as a stepping stone to mastering this essential mathematical skill, providing you with the tools to conquer both simple and complex expressions effortlessly.
What is the difference between an expression and an equation?
+
An expression does not contain an equal sign or inequality sign, whereas an equation equates two expressions using an equal sign.
How can I practice evaluating expressions efficiently?

+
Use structured practice problems that involve different types of expressions. Focus on drills where you have to calculate expressions with various combinations of variables, constants, and operations. Consistency and variety in your practice will enhance your speed and accuracy.
What if my expression includes fractions or decimals?

+
Evaluating expressions with fractions or decimals follows the same rules. However, you must be extra careful with the order of operations and ensure that your arithmetic with these numbers is accurate. Sometimes, converting fractions to decimals or vice versa can make the calculations easier.