Estimating Quotients: Easy Worksheets with Answers

The process of estimating quotients is a fundamental skill in mathematics that involves making educated guesses about the result of a division problem. This skill not only aids in mental arithmetic but also lays a groundwork for more advanced computational methods. In this comprehensive guide, we will explore what estimating quotients entails, why it's important, and provide practical worksheets to help learners of all ages practice this essential technique.
Understanding Estimation

Estimation is the art of making approximations rather than exact calculations. When we talk about estimating quotients, we're referring to finding a close, but not necessarily exact, value for the result of a division problem. Here’s why it matters:
- Practical Application: In daily life, exact figures are often not necessary. A quick estimation can save time and is useful for quick decisions.
- Building Number Sense: Estimation improves your understanding of numbers and their relationships, a key component of mathematical literacy.
- Error Detection: Estimation can serve as a sanity check for calculations, helping to spot mistakes.
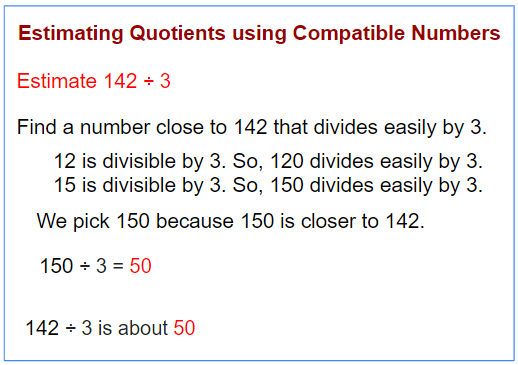
Steps to Estimate Quotients

Estimating quotients involves several straightforward steps:
- Round the numbers: Both the dividend and divisor should be rounded. For example, 398 can be rounded to 400, and 16 can be rounded to 20.
- Divide: Perform the division using the rounded numbers. If you're dividing 400 by 20, you get 20.
- Refine if needed: Sometimes, you might need to adjust your estimation based on how close the original numbers were to the rounded figures.
📝 Note: Remember, in estimation, precision is not the goal; getting a good approximation is what matters.
Practical Worksheets for Estimating Quotients

Here are some worksheets designed to help with practice:
| Problem | Estimated Quotient | Notes |
|---|---|---|
| 178 ÷ 45 | 180 ÷ 50 = 3.6 | Rounding down 178 to 180 and up 45 to 50 |
| 95 ÷ 23 | 100 ÷ 25 = 4 | 95 rounded up to 100, 23 rounded up to 25 |
| 629 ÷ 72 | 630 ÷ 70 = 9 | 629 rounded to 630, 72 rounded to 70 |
| 345 ÷ 9 | 350 ÷ 10 = 35 | 345 rounded up to 350, 9 rounded to 10 |

📝 Note: These worksheets are great for mental math practice and can be used in educational settings or for self-study.
Advantages of Estimating Quotients

Why should one bother with estimation?
- Quick Decision Making: In situations where exact numbers are not critical, estimation can lead to swift decisions.
- Developing Math Fluency: Regular estimation practice sharpens calculation skills and intuitive understanding of numbers.
- Applicability in Real-Life: Whether it's estimating the time for a journey or figuring out the approximate cost of an item, estimation is practical.
Conclusion

Estimating quotients is more than just a mathematical exercise; it's a skill that supports quick thinking and problem-solving. From everyday decisions to complex calculations, the ability to estimate provides a solid foundation for mathematical competence. By practicing with worksheets and understanding the underlying principles, anyone can become adept at this valuable technique, enhancing their numerical estimation capabilities.
Why is estimating quotients useful?

+
Estimating quotients helps in making quick mental calculations, detecting errors in manual calculations, and in situations where exact numbers are not necessary.
Can estimating quotients be taught to young children?
+
Absolutely! Simple estimation exercises can be incorporated into children’s math lessons to foster number sense and critical thinking from an early age.
How accurate should an estimation be?

+
Accuracy in estimation depends on the context. For everyday decisions, a rough estimate might suffice, whereas in more critical situations, you might aim for greater precision.
What are some common mistakes when estimating quotients?

+
Common mistakes include improper rounding, not considering the context for appropriate precision, or neglecting the implications of small errors in the initial numbers.