Master the Art of Estimating Quotients Easily

Understanding how to estimate quotients can be an invaluable skill, not only for tackling division in mathematics but also in real-world applications where quick estimations can enhance decision-making processes. In this comprehensive guide, we'll delve into various techniques that can help you master quotient estimation, making it easier, more intuitive, and less daunting.
Why Estimating Quotients is Important

Estimating quotients isn’t just about simplifying arithmetic; it’s about building an intuitive understanding of numbers and relationships between them. Here are some key reasons:
- Quicker Calculations: When exact division isn’t necessary, estimation speeds up the process.
- Problem-Solving: It aids in critical thinking by providing an approximate idea of outcomes.
- Real-Life Applications: From budgeting to sharing resources, quick quotient estimations are applicable in daily life.
- Building Number Sense: It develops your ability to predict results, understand number properties, and foster mathematical confidence.
📝 Note: Estimation skills aren't just for mathematicians; they're for anyone looking to make sense of numbers in various contexts.
Key Techniques for Quotient Estimation

Here, we’ll explore different strategies to make quotient estimation an effortless part of your numerical toolkit:
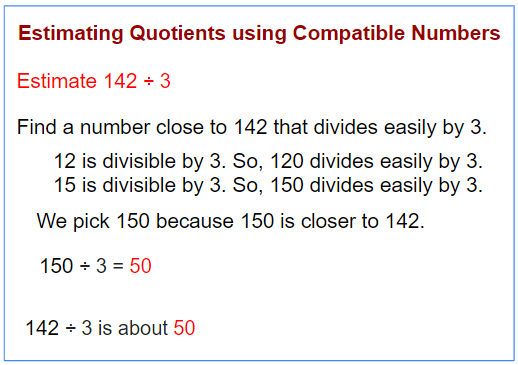
1. Rounding to Easier Numbers

By rounding numbers to simpler values, division becomes manageable. For instance:
- 548 ÷ 19 → Estimate as 550 ÷ 20 = 27.5
- 783 ÷ 23 → Estimate as 780 ÷ 24 = 32.5
⚠️ Note: While rounding, ensure the relative impact of rounding both numbers in the same direction.
2. Use of Benchmarks

Divide numbers by common benchmarks like 10, 5, 2, or numbers ending in zero or five:
| Dividend | Divisor | Estimation | Benchmark Used |
|---|---|---|---|
| 147 | 29 | ~ 5 | 150 ÷ 30 |
| 948 | 14 | ~ 68 | 950 ÷ 15 |

3. Fraction Estimation

Estimating with fractions can give you a good idea of the quotient:
- 395 ÷ 75 can be estimated as (4⁄3) × (3⁄1) = 4 (as 395 is roughly 1⁄3 of 1185, and 75 is just over 1⁄3 of 225).
4. Proportional Estimation

Using proportionality can help you gauge the quotient quickly:
- If 3 apples fit into a basket, how many baskets for 39 apples?
→ Estimate: 39 ÷ 3 = 13 baskets
5. Over/Under Estimation

Deliberately over- or under-estimating can bracket your quotient:
- 498 ÷ 23: Over-estimate to 500 ÷ 25 = 20, Under-estimate to 450 ÷ 25 = 18; so the quotient is between 18 and 20.
🔍 Note: Practice is key to developing an instinct for when to use over/under estimation effectively.
Advanced Strategies for Estimation

For more complex scenarios, these advanced techniques might be handy:
1. The “Decomposition” Technique

This involves breaking the problem into smaller, manageable parts:
- 894 ÷ 37 can be simplified to (900 - 6) ÷ 37, which could be approximated by 900 ÷ 36 = 25 (since 37 is slightly larger).
2. The “Digit Placement” Strategy

Shift the divisor and dividend to make the division easier:
- 742 ÷ 26 could be approached as 74.2 ÷ 2.6 = 28.538 ≈ 29
📐 Note: When using this method, pay attention to the decimal place shift to avoid miscalculation.
Estimating Quotients in Real-Life Scenarios
The practical application of these techniques can save time and reduce errors:
Budgeting

When managing a budget, if you need to divide your total funds by the number of months, weeks, or even days, estimation can be a lifesaver. For instance:
- If you have 14,783 to spend over 365 days, estimating 15,000 ÷ 365 ≈ 41.1, you'd know you can spend roughly 41 a day.
Meal Planning

Planning meals for a group can be simplified with estimation:
- If you need to divide 18 eggs by servings for 6 people, estimating 18 ÷ 6 = 3 means each person gets roughly 3 eggs.
👪 Note: Estimation is particularly useful when exact measurements aren't critical, such as in portion sizes for a large group.
Business Decisions

In business, estimating can help with pricing, inventory management, and forecasting:
- If your product costs $12.99 to produce and you want to sell at a 40% markup, estimating 12.99 ÷ 1.4 ≈ 9.28 gives you an approximate wholesale price.
In summarizing these insights, the ability to estimate quotients empowers us with a versatile skillset, enhancing our decision-making in numerous contexts. We’ve explored why this skill is essential, simple yet effective strategies, advanced techniques, and how these methods apply to real-world scenarios. The overarching theme is that with practice, estimating quotients becomes an intuitive process, allowing for quick mental calculations that inform our everyday choices and strategic planning. Now let’s address some common questions regarding estimating quotients.
Why is estimating quotients useful in daily life?
+
Estimating quotients allows for quick calculations and decision-making in real-life situations like budgeting, meal planning, time management, and business. It helps in understanding the scale and making informed choices swiftly.
Can I use estimation for all division problems?
+
While estimation can be applied to most situations where exact precision isn’t necessary, for tasks requiring precise measurements (like engineering, pharmacology, or financial accounting), it’s crucial to use exact division for accuracy.
How do I know if my estimation is accurate?
+
The best way to check the accuracy of your estimation is to practice. Over time, as you refine your skills, you’ll develop a sense for how close your estimations are to the exact answer. You can also occasionally verify with exact division to calibrate your estimations.



