Master Equations with Variables on Both Sides Easily

Understanding how to solve equations with variables on both sides is an essential skill in algebra, as it equips students with the ability to manipulate and solve more complex equations. Whether you're a student trying to grasp these concepts or a teacher looking to enhance your students' understanding, this guide will take you through the process step-by-step.
The Basics of Algebraic Equations

Before we dive into solving equations with variables on both sides, it’s crucial to grasp some fundamental concepts:
- Equations: An equation is a statement that shows two expressions are equal.
- Variables: These are letters or symbols that represent unknown quantities.
- Coefficients: These are the numerical values associated with a variable in an equation.

Solving Equations with Variables on Both Sides

Step-by-Step Guide

Let’s take an example to demonstrate the process:
Solve: 3x + 4 = 8 + 2x
Step 1: Move Variables to One Side
- First, decide which side to keep the variables on. Most often, we move them to the left side:
- To keep x on the left side, subtract 2x from both sides:
3x + 4 - 2x = 8 + 2x - 2x
Step 2: Simplify
- This simplifies to:
x + 4 = 8
Step 3: Move Constants to One Side
- Subtract 4 from both sides to isolate the variable:
x + 4 - 4 = 8 - 4
Step 4: Solve for x
- The equation now reads:
x = 4
✏️ Note: You can move terms to either side of the equation, but consistency in your method can make solving easier.
Common Mistakes to Avoid

Here are some common pitfalls when solving these equations:
- Not Isolating the Variable: Always ensure you isolate the variable on one side.
- Mistakes in Sign Changes: When moving terms, make sure to change the sign correctly.
- Distribution Errors: Remember to distribute correctly when simplifying expressions like -2(x + 3).

Advanced Techniques for Complex Equations

Combining Like Terms and Simplifying

When dealing with equations like 2x + 5 = 7x + 3, you need to:
- Combine like terms on both sides:
- 2x remains on the left.
- On the right, subtract 2x from both sides to get:
- This simplifies to:
- Subtract 3 from both sides:
- Divide by 5:
2x + 5 - 2x = 7x + 3 - 2x
5 = 5x + 3
5 - 3 = 5x
x = 2⁄5
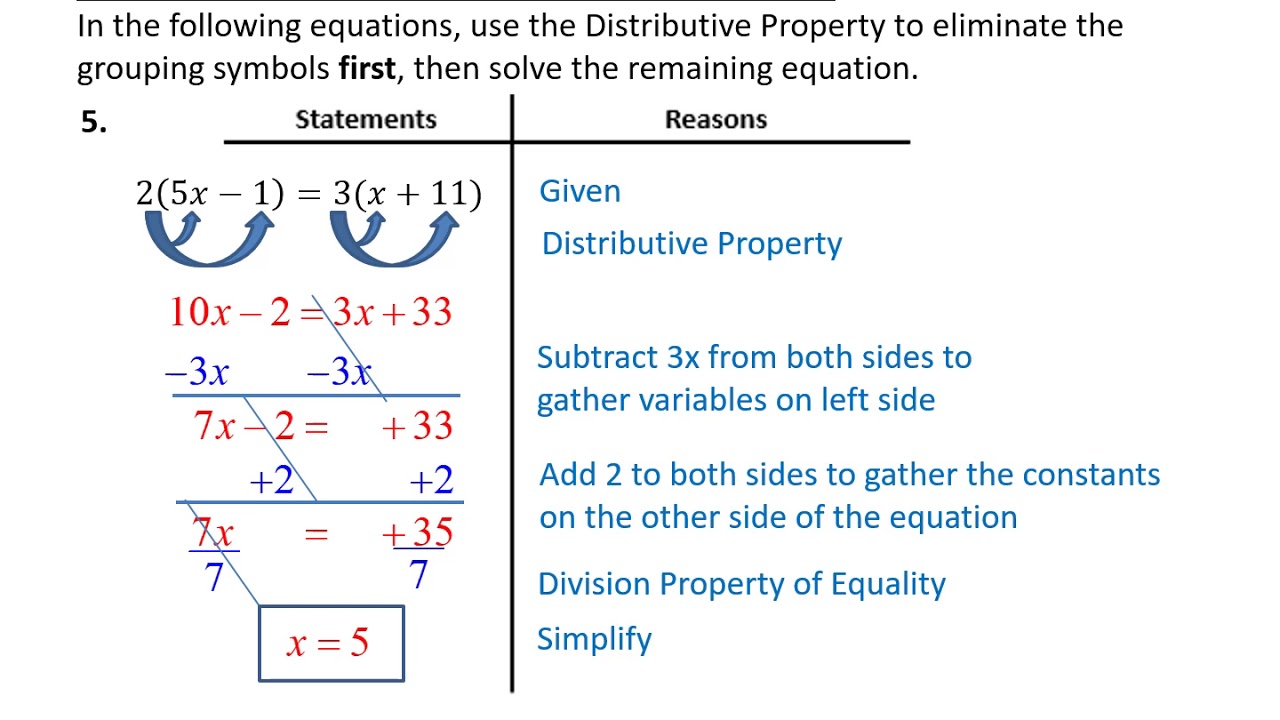
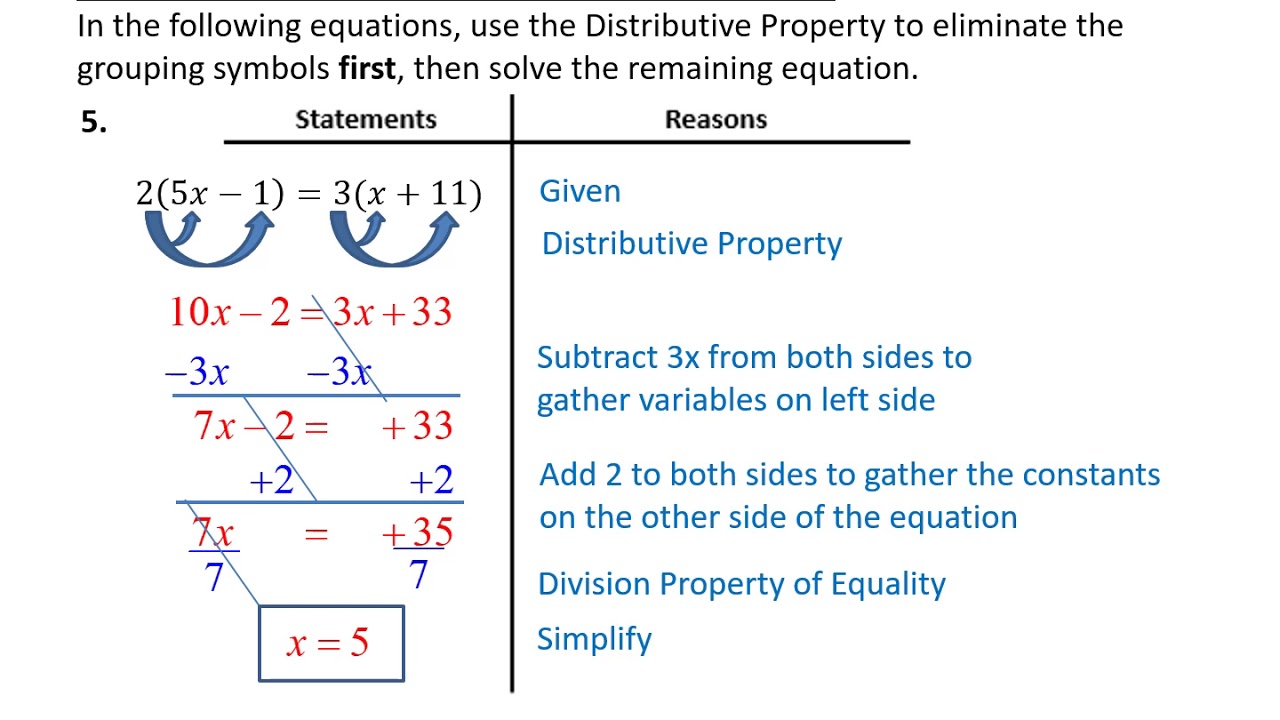
Using Distributive Property

Consider the equation 4(x + 1) = 6x - 8:
- Distribute 4 to x + 1:
4x + 4 = 6x - 8
4 = 6x - 8 - 4x
4 = 2x - 8
4 + 8 = 2x
12 = 2x x = 12 / 2 x = 6
📚 Note: Using the distributive property correctly is key to simplifying complex equations.
Understanding Equation Transformations

Transforming an equation involves changing its form without changing its solutions:
- Adding or Subtracting: This changes only one side of the equation. For instance, if you add 3 to both sides of 2x = 6, you get 2x + 3 = 9.
- Multiplying or Dividing: Multiplying both sides by the same non-zero number changes the coefficients. For example, multiplying 2x = 6 by 2 gives 4x = 12.
🔍 Note: You can perform any operation on both sides of an equation as long as it’s done consistently to maintain equality.
By following these steps and techniques, you'll find solving equations with variables on both sides to be more manageable. This skill not only aids in passing algebra tests but also in understanding higher math concepts. So, practice regularly, focus on avoiding common mistakes, and you'll soon become adept at manipulating algebraic equations with ease.
What if there are fractions in the equation?
+
Use the least common denominator (LCD) to eliminate fractions. For example, for 3/4x = 2⁄5, multiply both sides by 20 (LCD of 4 and 5): 3/4x * 20 = 2⁄5 * 20, which simplifies to 15x = 8, then solve for x.
Can I use these methods with equations involving decimals?

+
Yes, the same principles apply. Treat decimal numbers as fractions, multiply by a power of 10 to clear the decimals, and proceed with normal methods.
What do I do when there are no solutions or infinitely many solutions?
+
This occurs when simplifying results in either a false statement (e.g., 2 = 3) or an identity (e.g., 5 = 5). If false, there’s no solution; if true, there are infinitely many solutions.



