Solve Equations With Fractions in 5 Easy Steps
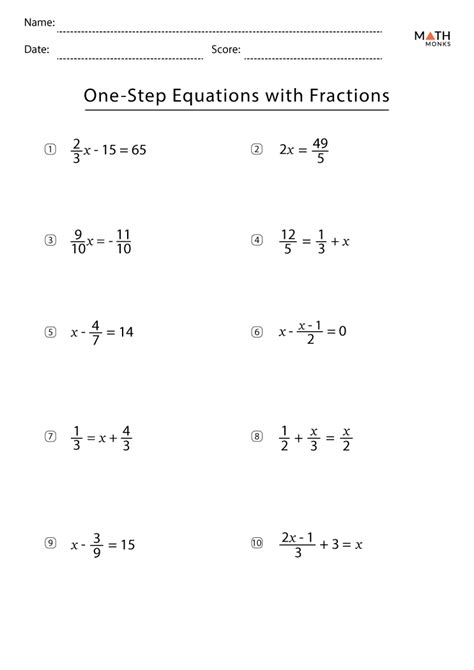
Solving Equations with Fractions: A Step-by-Step Guide

Solving equations with fractions can be a daunting task, especially for those who struggle with math. However, with the right approach and techniques, it can be made easier. In this article, we will break down the process of solving equations with fractions into 5 easy steps. By following these steps, you will be able to solve equations with fractions with confidence.
Step 1: Identify the Type of Equation

Before solving an equation with fractions, it’s essential to identify the type of equation you are dealing with. Is it a linear equation, quadratic equation, or a rational equation? This will help you determine the best approach to solve the equation.
Types of Equations:
- Linear equation: ax + b = c
- Quadratic equation: ax^2 + bx + c = 0
- Rational equation: a/b = c/d
📝 Note: Make sure to identify the type of equation to choose the correct method for solving.
Step 2: Eliminate the Fractions

To eliminate the fractions, you need to multiply both sides of the equation by the least common denominator (LCD) of the fractions. The LCD is the smallest number that is divisible by both denominators.
Example:
1/2x + 1⁄4 = 3⁄4
LCD = 4
Multiply both sides by 4:
4(1/2x + 1⁄4) = 4(3⁄4)
2x + 1 = 3
📝 Note: Make sure to multiply both sides of the equation by the LCD to eliminate the fractions.
Step 3: Simplify the Equation
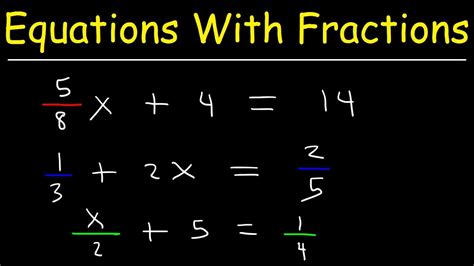
After eliminating the fractions, simplify the equation by combining like terms and performing any necessary operations.
Example:
2x + 1 = 3
Subtract 1 from both sides:
2x = 2
Divide both sides by 2:
x = 1
Step 4: Check for Extraneous Solutions

When solving rational equations, it’s essential to check for extraneous solutions. Extraneous solutions are values of the variable that are not valid solutions to the original equation.
Example:
x = 1
Check if x = 1 is a valid solution:
1⁄2(1) + 1⁄4 = 3⁄4
1⁄2 + 1⁄4 = 3⁄4
3⁄4 = 3⁄4
x = 1 is a valid solution.
Step 5: Write the Final Answer
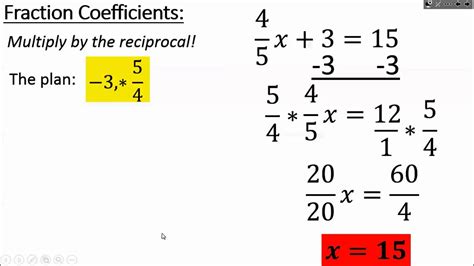
The final step is to write the final answer in the required format.
Example:
x = 1
📝 Note: Make sure to write the final answer in the required format.
What is the least common denominator (LCD)?

+
The LCD is the smallest number that is divisible by both denominators.
How do I eliminate fractions in an equation?

+
Multiply both sides of the equation by the LCD to eliminate the fractions.
What is an extraneously solution?

+
An extraneously solution is a value of the variable that is not a valid solution to the original equation.
In conclusion, solving equations with fractions can be made easier by following these 5 easy steps: identify the type of equation, eliminate the fractions, simplify the equation, check for extraneously solutions, and write the final answer. By mastering these steps, you will be able to solve equations with fractions with confidence.
Related Terms:
- Equations with fractions worksheet pdf
- Equations With Fractions Worksheet Kuta
- solving equations with fractions printable
- equations with fractions grade 11
- equations with fractions worksheet kuta
- solve equations with fraction coefficients