Mastering Domain and Range: Piecewise Functions Worksheet

Understanding functions, especially piecewise functions, is essential in mathematics. Piecewise functions allow us to define different behaviors for different intervals of the domain. This post will delve into the world of domain and range for piecewise functions, providing insights on how to identify, analyze, and master these critical aspects. Whether you're preparing for an exam or just looking to sharpen your math skills, this guide will equip you with the tools you need.
What Are Piecewise Functions?
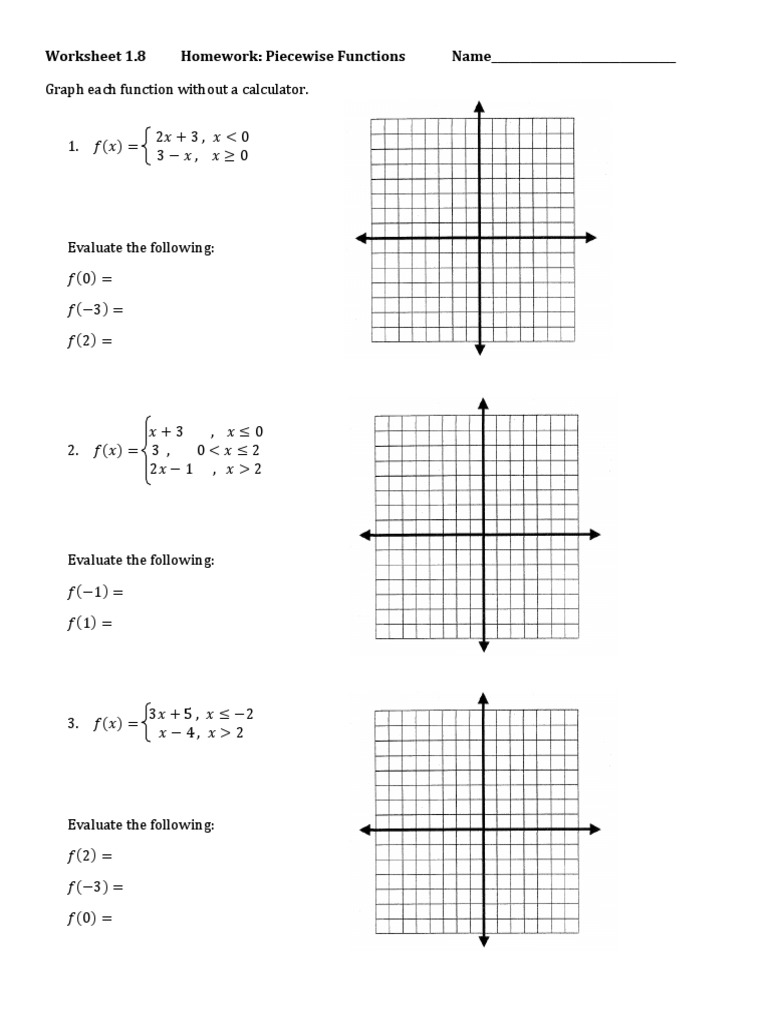
A piecewise function, as the name suggests, is made up of several pieces, each defined over its own interval of the domain. Here’s a basic example:
- f(x):
- when x < -1, f(x) = x + 2
- when -1 ≤ x ≤ 1, f(x) = x²
- when x > 1, f(x) = 2x - 1
Each piece describes the function's behavior within its specific domain interval. Understanding how to work with these functions is crucial for finding their domain and range.
Finding the Domain of Piecewise Functions

The domain of a piecewise function is the union of all domains of its component pieces:
- Identify each piece’s domain: Each piece has its own interval.
- Combine them: The overall domain includes all x-values from each piece, ensuring no overlap or contradiction between pieces.
Example Analysis:

Let’s use our previous example:
| Function | Domain |
|---|---|
| f(x) = x + 2 | x < -1 |
| f(x) = x² | -1 ≤ x ≤ 1 |
| f(x) = 2x - 1 | x > 1 |

📝 Note: The domain of this piecewise function is all real numbers. Pay attention to how each interval connects or overlaps.
Understanding the Range of Piecewise Functions

The range of a piecewise function requires understanding how each piece behaves within its domain:
- Analyze each piece: Look at the maximum and minimum values each piece can take.
- Combine results: The range is the union of all these sets of output values.
Visualizing the Range:
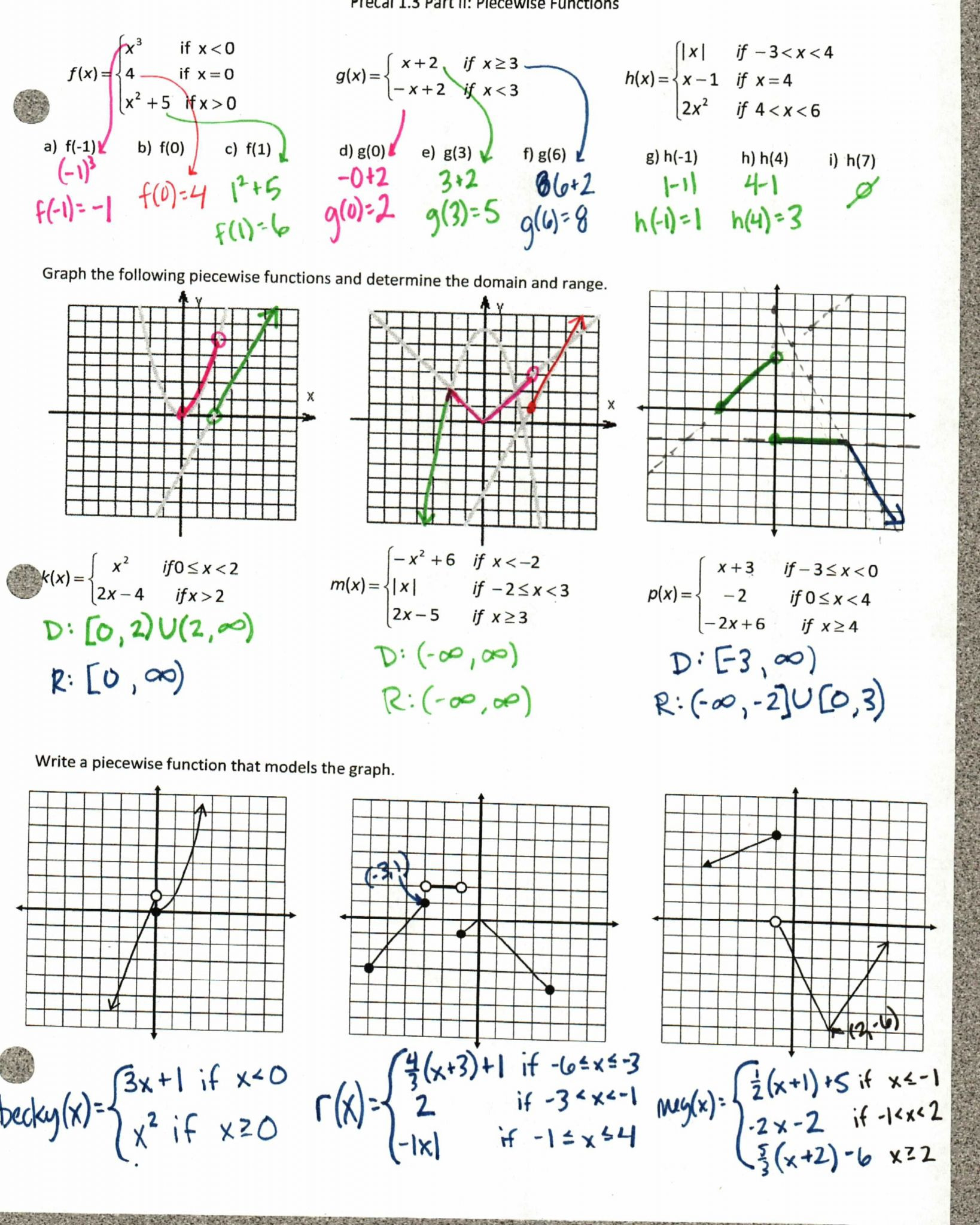
Graphing the function can be particularly helpful. Here’s how to proceed:
- Plot each piece on its domain to understand the function’s behavior visually.
- Identify the highest and lowest points of each piece on the y-axis.
- Ensure you consider any points of intersection or transitions between pieces.
Example:

Using our previous example:
- For x < -1, f(x) = x + 2, which gives all values less than or equal to 1.
- For -1 ≤ x ≤ 1, f(x) = x², which gives values from 0 to 1.
- For x > 1, f(x) = 2x - 1, which gives all values greater than 1.
Combining these results, the range for this piecewise function is all real numbers except for the interval (0, 1).
By now, you should have a grasp of the basic strategies to identify the domain and range of piecewise functions. However, there are intricacies to consider:
Advanced Concepts and Considerations

Discontinuity

Piecewise functions can have points of discontinuity, where the function’s behavior changes abruptly at specific points, often where the pieces connect. Here are some types:
- Jump Discontinuity: When the function has different left-hand and right-hand limits at a point.
- Removable Discontinuity: If a piece is undefined at a point but can be made continuous by redefining the function at that point.
- Infinite Discontinuity: Where the function approaches positive or negative infinity at a point.
Endpoints and Intervals

Attention to endpoints is crucial:
- Open Intervals: Use of ( ) to exclude endpoints.
- Closed Intervals: Use of [ ] to include endpoints.
- Half-Open or Half-Closed: A combination of ( ] or [ ) to indicate specific inclusion or exclusion.
Final Thoughts

This exploration of domain and range within piecewise functions provides a foundational understanding for further mathematical explorations. By mastering these concepts, you’re not only improving your problem-solving skills but also your ability to model real-world phenomena where behaviors change based on conditions. Remember, practice and consistent application will cement these skills. The examples and techniques shared here should guide your journey in mastering piecewise functions.
How can I ensure my piecewise function doesn’t have gaps or overlaps?

+
To avoid gaps or overlaps, carefully define each piece’s domain to ensure no overlapping intervals or gaps between them. Make sure the endpoints are appropriately included or excluded to meet the function’s continuity requirements.
What if a piecewise function has a point of discontinuity?

+
At points of discontinuity, the function’s value might differ on either side. Consider these when analyzing the function’s behavior, range, and overall continuity.
Can a piecewise function have an infinite domain?

+
Yes, if the intervals of the function pieces cover all real numbers or if one piece extends infinitely in one direction, the domain can indeed be infinite.
What role do graphing tools play in understanding piecewise functions?

+
Graphing tools help visualize how the function behaves within its domain, making it easier to identify the range, continuity, and potential points of discontinuity or intersections.
How should I interpret a piecewise function with complex pieces?

+
Analyze each piece individually, considering its domain and range. Then, combine these insights to understand the function’s behavior as a whole.



