5 Tips to Master Domain and Range Graph Analysis
Understanding the domain and range of functions is fundamental in mathematics, especially when dealing with graphical representations. Whether you're tackling problems in algebra, calculus, or advanced data analysis, knowing how to read and interpret these elements can significantly enhance your problem-solving skills. Here are five expert tips to help you master the analysis of domain and range through graphical methods:
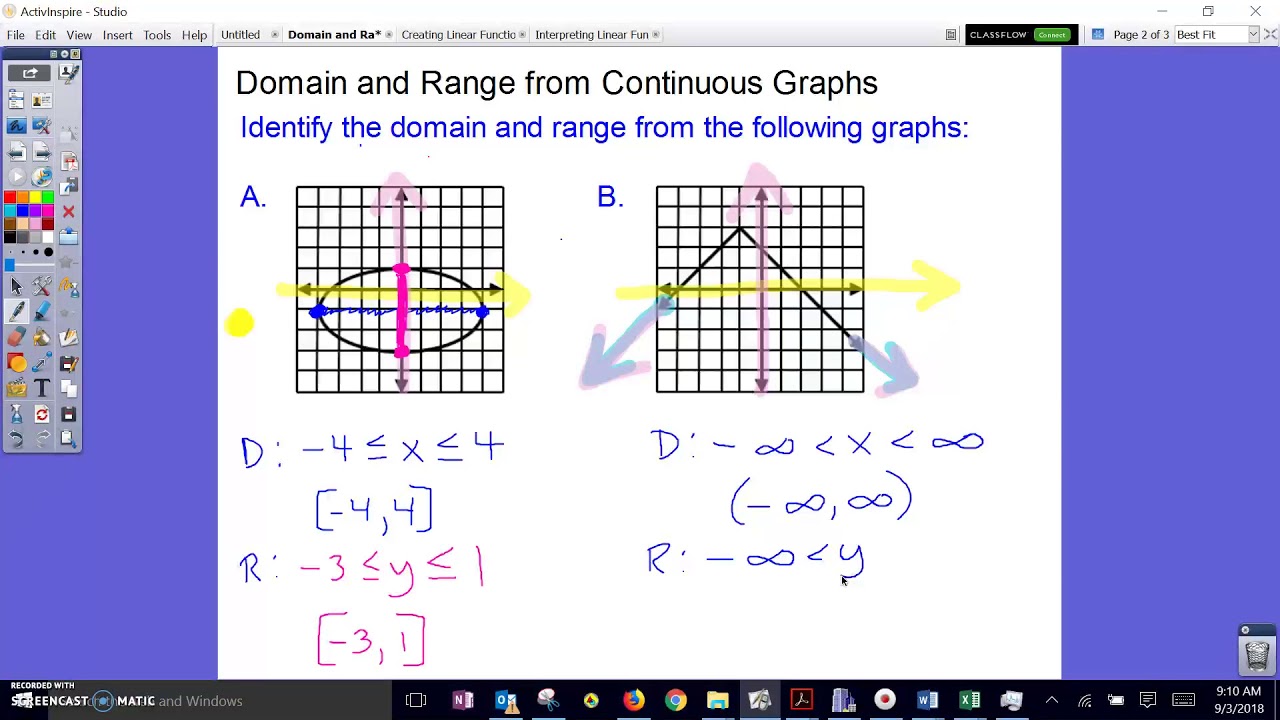
Tip 1: Understand the Basics of Domain and Range

Before diving into complex graphical analysis, ensure you have a solid understanding of what domain and range mean:
- Domain: All possible input values (x-values) for which the function is defined.
- Range: All possible output values (y-values) that the function can take.

🎓 Note: Understanding the theoretical basis is crucial before you start interpreting graphs to avoid misinterpretation of data.
Tip 2: Identify Vertical and Horizontal Lines

A quick way to determine the domain and range from a graph involves looking at vertical and horizontal lines:
- Domain: Any vertical line should intersect the graph at least once. If there's a gap or an asymptote, that vertical line doesn't intersect, indicating that x-values in that region are not in the domain.
- Range: Similarly, any horizontal line should cross the graph. If there's no intersection at all or an asymptote, that y-value is not in the range.
📌 Note: This method is particularly useful for complex functions with discontinuities or asymptotes.
Tip 3: Use Intervals Notation

When reporting the domain and range, using interval notation can provide a clear, concise description:
- For open intervals: Use ( ) when the endpoints are not included.
- For closed intervals: Use [ ] when the endpoints are included.
- Use ∞ (infinity) for unbounded ends.
| Function | Domain | Range |
|---|---|---|
| f(x) = 1/x | (-∞, 0) ∪ (0, ∞) | (-∞, 0) ∪ (0, ∞) |
| f(x) = √x | [0, ∞) | [0, ∞) |

Tip 4: Analyze Piecewise Functions

Graphical analysis of piecewise functions requires an understanding of each piece's domain and how these pieces combine:
- Examine each segment of the piecewise function separately.
- Combine the domains of all segments, noting any points of discontinuity or overlap.
- Consider both the domain and range for each segment when determining the overall range.
💡 Note: Graphing software can greatly simplify the visualization and analysis of piecewise functions.
Tip 5: Consider Transformations
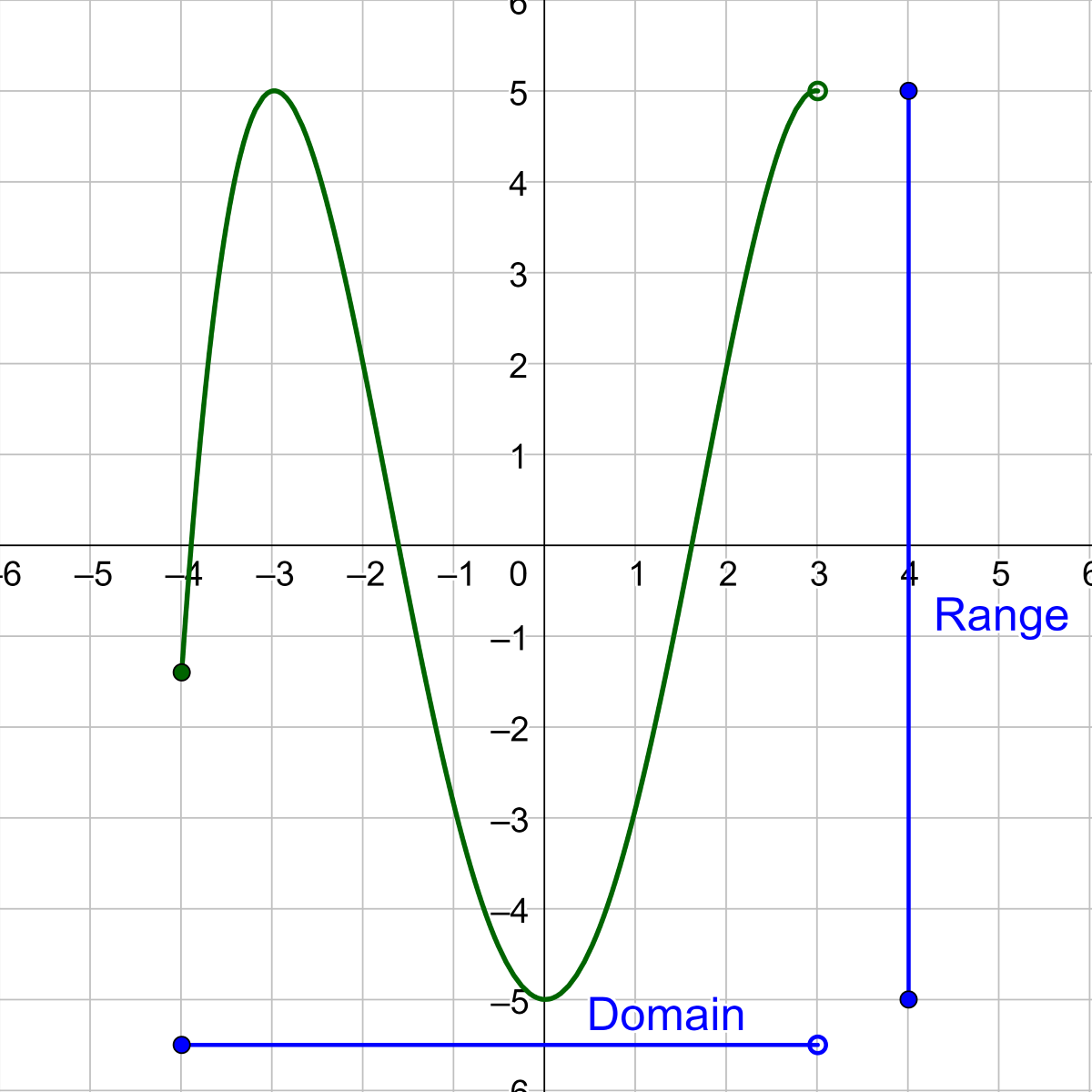
Understanding how transformations affect domain and range can be a game-changer:
- Vertical shifts: Add or subtract to the y-values. A vertical shift does not change the domain but can alter the range.
- Horizontal shifts: Add or subtract to the x-values. This changes the domain but leaves the range unchanged if the function maintains its shape.
- Reflections: Reflecting a function changes the range but not the domain.
- Stretching and compressing: Multiplying by constants can change both the domain and range depending on whether it's vertical or horizontal stretching/compressing.
Mastering these transformations helps in visualizing how a function changes and interpreting its domain and range from the graph.
📈 Note: Remember that vertical transformations do not affect the domain, but horizontal transformations can change it significantly.
In the process of mastering domain and range analysis, the journey involves not just reading graphs but understanding the mathematical principles behind them. Each graph tells a story of function behavior, and by following these tips, you can become fluent in interpreting this mathematical narrative. Whether solving an algebraic equation, plotting data points, or modeling real-world phenomena, these insights into domain and range will serve as your toolkit for effective problem-solving.
What if a function has a discontinuity?

+
If a function has a discontinuity, you’ll need to exclude that x-value from the domain and possibly adjust the range if the gap affects the possible y-values the function can produce.
Can the domain or range of a function be infinite?
+
Yes, both the domain and range can be infinite. For instance, polynomial functions can have domains and ranges that extend to positive or negative infinity.
How do you handle undefined values in functions?

+
If a function is undefined at certain points (like division by zero), those points are not included in the domain, and you’ll need to express that in your analysis.