5 Easy Worksheets for Division with Partial Quotients

Learning division can be a challenging task for students, but with the right strategies, it can become much easier and even enjoyable. One such strategy is using partial quotients, an intuitive method that breaks down the division process into smaller, more manageable parts. This approach not only simplifies the division but also makes the learning process engaging for students. In this post, we'll delve into five easy worksheets designed to teach division using partial quotients, ensuring that your students or children grasp this method effectively.
Understanding Partial Quotients


Before we jump into the worksheets, it’s essential to understand what partial quotients are:
- Partial Quotients: Instead of finding the entire quotient at once, you determine smaller parts of the quotient step by step, subtracting these from the dividend until you reach zero or a number less than the divisor.
This method allows for:
- Less reliance on memorization of multiplication facts.
- Students to see the division process visually.
- Building confidence as they solve larger numbers through smaller steps.
Worksheet 1: Introduction to Partial Quotients

In this first worksheet, we aim to familiarize students with the concept:
- Simple Division: Start with basic examples like 24 ÷ 3 where partial quotients could be 8 (3x8=24).
- Visual Representation: Show the division process graphically, subtracting parts of the dividend until zero.
- Fill in the Blanks: Provide exercises where students fill in partial quotients or draw on the visual diagram.
💡 Note: Use empty boxes to represent spaces where students can write or draw the steps involved in partial quotients.
Worksheet 2: Two-Digit Divisors

Progress to divisions with two-digit divisors:
| Problem | First Partial Quotient | Subtraction | Next Partial Quotient | Final Answer |
|---|---|---|---|---|
| 65 ÷ 15 | 3 | (15x3) = 45 | 1 (last step) | 4 remainder 1 |

- Estimate: Teach students to estimate the largest multiplier without going over the dividend.
- Multiple Partial Quotients: Continue subtracting until students reach a number where they know the next partial quotient.
Worksheet 3: Long Division with Partial Quotients
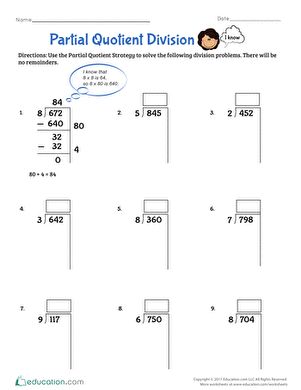
This worksheet introduces students to long division using the partial quotients method:
- Setup: Teach how to set up the long division problem.
- Repeated Subtraction: Show repeated subtractions of partial quotients until students reach a remainder they can work with.
- Checkpoint: Add checkpoints where students verify their steps.
💡 Note: Emphasize the importance of checking subtraction and multiplication steps to ensure accuracy.
Worksheet 4: Word Problems

Applying partial quotients to real-life scenarios:
- Contextual Problems: Use scenarios where division is necessary (e.g., sharing items equally among friends).
- Varied Contexts: Include different contexts like sharing money, measuring ingredients, or arranging seats in a theater.
- Step-by-Step Guide: Students must detail each step they take to solve the problem.
Worksheet 5: Challenges and Advancements
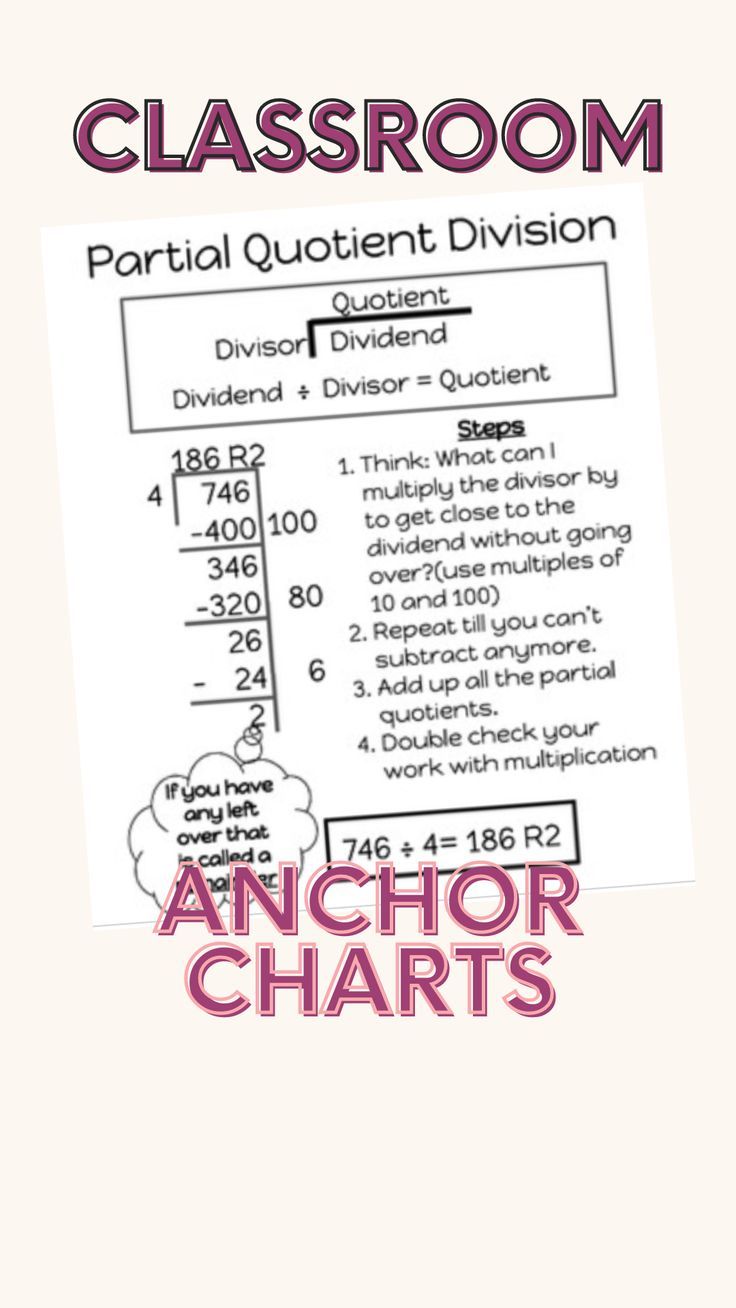
This worksheet challenges students with more complex problems:
- Higher Divisors: Introduce larger divisors where partial quotients are more steps apart.
- Decimal Results: Include problems where the final answer includes decimals.
- Summarize: Have students summarize the steps they took in solving each problem, reinforcing the learning process.
In closing, the method of partial quotients offers a practical and visual way to teach division. Through these five worksheets, students can progress from basic understanding to solving more advanced division problems, all while building confidence in their math skills. This approach not only aids in understanding the concept of division but also in applying it to various scenarios, enhancing both practical math application and cognitive development. Using these worksheets, educators and parents can guide students towards mastering division with greater ease and less anxiety, making math an adventure rather than a challenge.
Why is using partial quotients beneficial for learning division?
+
Partial quotients break down the division process into smaller, more manageable steps, reducing the complexity and making it easier for students to understand the underlying concepts of division.
How can I help a student who struggles with traditional long division?

+
Consider introducing partial quotients. This method can be less intimidating because it focuses on simpler subtractions, allowing students to build up to the total quotient incrementally.
Can partial quotients be used for any division problem?

+
Yes, partial quotients can be applied to any division problem, although it becomes more efficient with larger numbers or more complex divisions.