Mastering Division of Fractions: Free Worksheet Included

Understanding the division of fractions can be a pivotal step in mastering mathematics, especially for students transitioning into more advanced arithmetic or algebra. In this comprehensive guide, we will delve into the world of fraction division, providing insights, techniques, and a free downloadable worksheet to enhance your learning experience.
Why Divide Fractions?

Division of fractions might seem like an esoteric operation, but it is fundamental in real-world applications. From cooking recipes that require you to scale down servings to understanding ratios and proportions in engineering, dividing fractions opens up a multitude of practical scenarios:
- Resizing recipes: Need to make half of a recipe? Dividing fractions helps here.
- Construction: Understanding material measurements or reducing dimensions proportionally.
- Mathematics: Foundational for algebraic manipulation and solving complex equations.

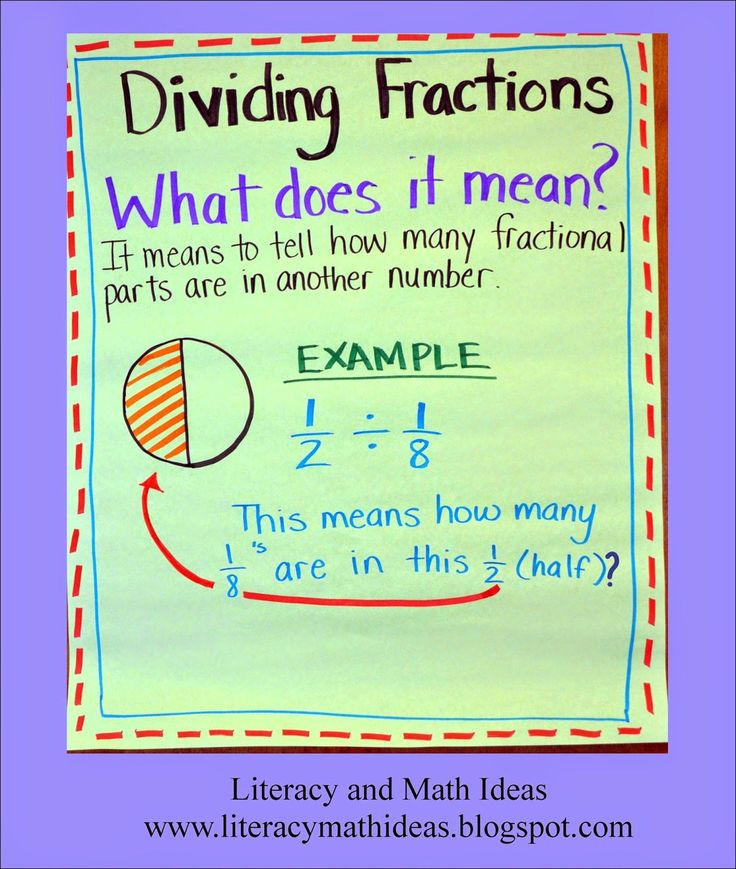
The Basic Concept

Before we get into the nitty-gritty of the division process, let’s understand the fundamental idea:
Dividing by a fraction is the same as multiplying by its reciprocal. This reciprocal transformation simplifies the operation from division to multiplication, which is generally more intuitive.
How to Divide Fractions

Follow these steps to divide fractions:
- Identify the reciprocal: The reciprocal of a fraction is obtained by swapping the numerator and the denominator.
- Multiply instead of divide: Instead of dividing by the second fraction, multiply by its reciprocal.
- Simplify the result: Reduce the product to its simplest form if possible.
Example
Let’s take the division:
(\frac{3}{4} ÷ \frac{1}{2} )
Here, the second fraction’s reciprocal is ( \frac{2}{1} ). So, we have:
(\frac{3}{4} \times \frac{2}{1} = \frac{3 \times 2}{4 \times 1} = \frac{6}{4} )
Simplifying (\frac{6}{4}), we get (\frac{3}{2}) or (1 \frac{1}{2}).
💡 Note: While this method makes division of fractions more approachable, ensure you understand the conceptual reasoning behind reciprocal multiplication.
Common Pitfalls and Tips
Here are some common mistakes to avoid and tips for successful fraction division:
- Remember to flip: Always find the reciprocal before multiplying.
- Simplify first: If possible, simplify fractions before dividing to reduce complexity.
- Sign matters: Be mindful of negative signs; the final sign depends on how many negative fractions are involved.
- Mixed numbers: Convert mixed numbers to improper fractions first to make division simpler.
| Fraction | Reciprocal |
|---|---|
| \frac{1}{2} | 2 |
| \frac{5}{6} | \frac{6}{5} |
| 1 | 1 |

💡 Note: A fraction and its reciprocal will always multiply to 1 when multiplied by each other.
Worksheet: Divide and Conquer

To truly master dividing fractions, practice is key. Here’s a free downloadable worksheet with exercises to help you:
Download the Fraction Division WorksheetThe worksheet includes:
- Simple division of fractions
- Word problems to apply division in real-life scenarios
- Exercises with mixed numbers
📝 Note: While practicing, aim for accuracy and speed to increase your proficiency.
In conclusion, dividing fractions may initially seem daunting, but with the right approach and consistent practice, it becomes straightforward. By understanding the reciprocal concept, applying practical tips, and engaging with structured exercises like the ones provided in the worksheet, you'll not only grasp the mechanics but also appreciate the logic behind this arithmetic operation. Remember, mathematics is not just about numbers; it's about the beauty of problem-solving, and fractions division is just one fascinating piece of that puzzle.
Why is division of fractions performed by multiplying by the reciprocal?

+
This method allows us to convert division into multiplication, making the arithmetic operation simpler to visualize and compute.
Can you divide a fraction by a whole number?

+
Yes, you can. Treat the whole number as a fraction with a denominator of 1, then proceed with the reciprocal multiplication.
How do you simplify fractions after dividing?

+
After performing the multiplication, look for the greatest common divisor (GCD) of the numerator and the denominator to reduce the fraction to its simplest form.