5 Easy Ways to Divide Whole Numbers by Fractions

In the vast landscape of mathematics, understanding how to manipulate different number types is essential. Today, we're diving into a seemingly complex yet straightforward operation: dividing whole numbers by fractions. Whether you're helping with homework or need this knowledge for real-world applications like scaling recipes or measurements, mastering this operation can streamline your mathematical tasks.
The Concept: Dividing by a Fraction

Dividing a whole number by a fraction might initially sound intimidating, but it's based on a simple principle. Here's the golden rule:
Dividing by a fraction is the same as multiplying by its reciprocal.
Let's break this down:
- When you divide by a fraction, you're essentially asking how many fractions fit into the whole number.
- The reciprocal of a fraction, a/b, is b/a. This inverts the fraction, swapping the numerator and the denominator.
Why This Works

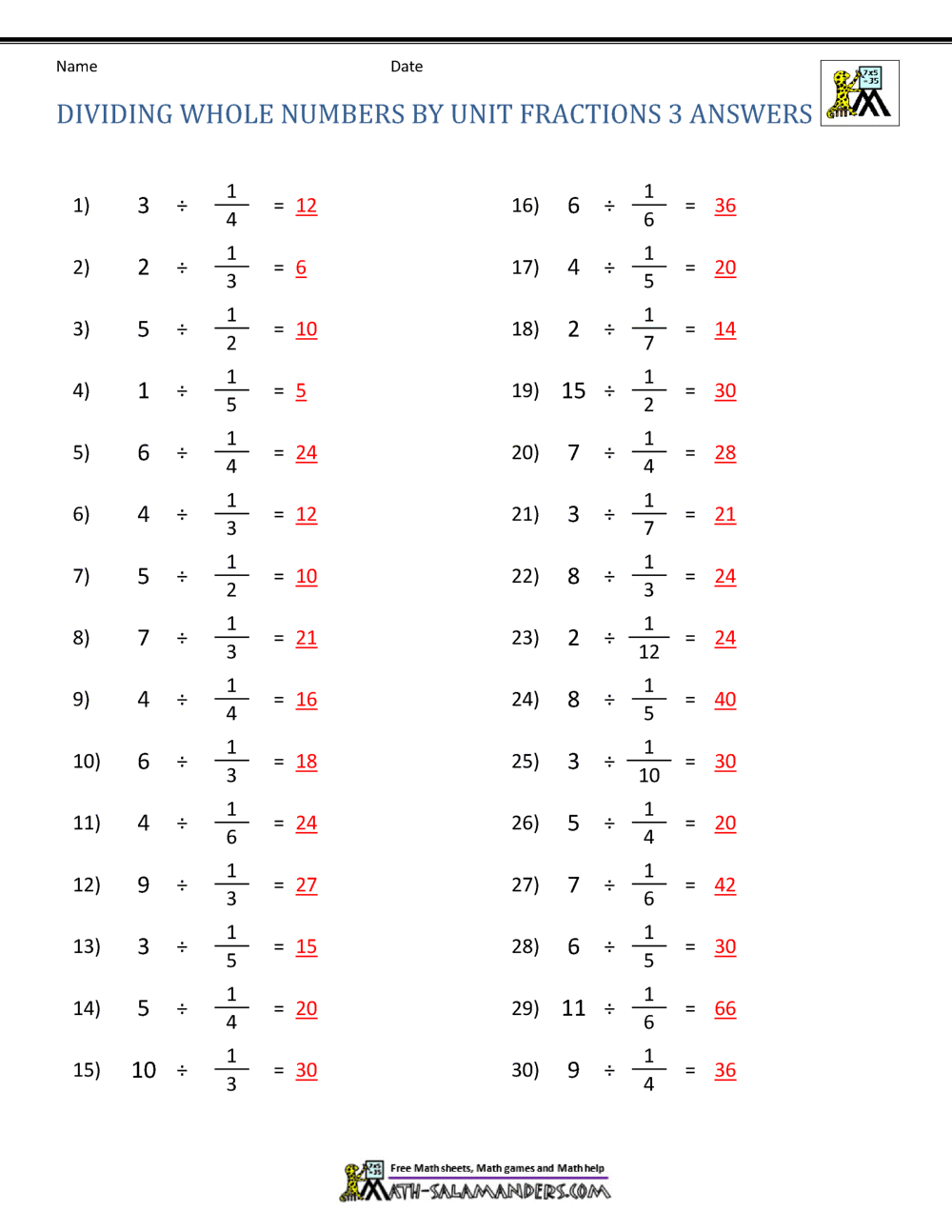
Consider dividing 6 by 1/2. Instead of dividing, you ask, "How many halves are in 6?"
Mathematically: 6 ÷ 1/2 = 6 * 2 (since 2 is the reciprocal of 1/2).
It's all about understanding that dividing by a fraction means you're scaling up, and the reciprocal scales down the fraction back into a whole number context.
Step-by-Step Methods

Method 1: Multiply by the Reciprocal
- Identify the Reciprocal: The reciprocal of a/b is b/a.
- Multiply: Multiply the whole number by this reciprocal.
- Simplify: If possible, simplify the result or leave it in its lowest terms.
For example:
| Problem: | 8 ÷ 1/4 |
| Reciprocal: | 1/4's reciprocal is 4/1 |
| Multiplication: | 8 * 4/1 = 32 |
✏️ Note: Remember, if your fraction is improper, convert it to a mixed number or whole number before finding the reciprocal.
Method 2: Keep, Change, Flip
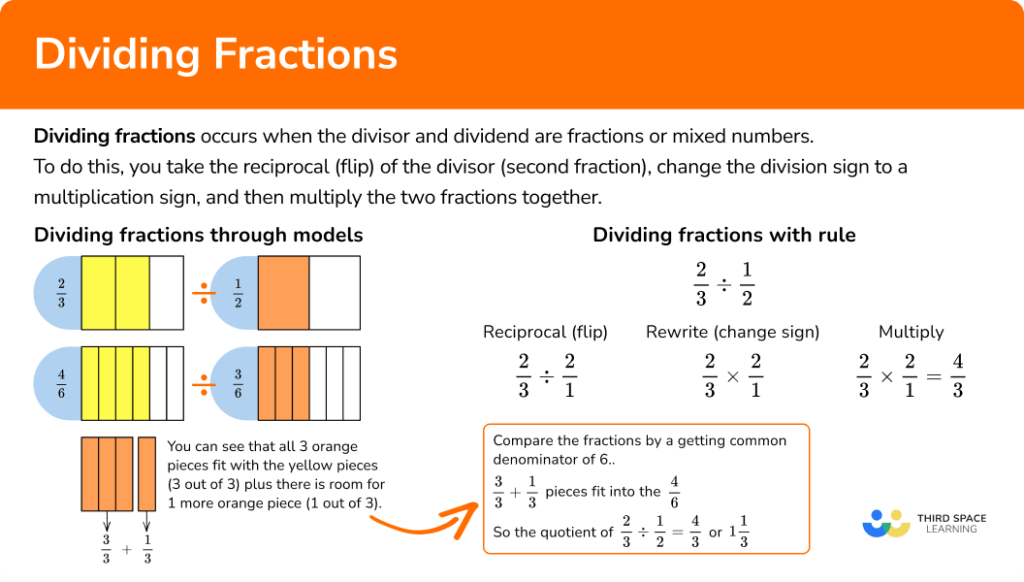
Another straightforward method often taught in schools:
- Keep: Keep the whole number as is.
- Change: Change the division sign to multiplication.
- Flip: Flip the fraction, turning it into its reciprocal.
For example:
| Problem: | 5 ÷ 2/3 |
| Keep: | 5 |
| Change: | ÷ becomes * |
| Flip: | 3/2 |
| Result: | 5 * 3/2 = 7 1/2 or 15/2 |
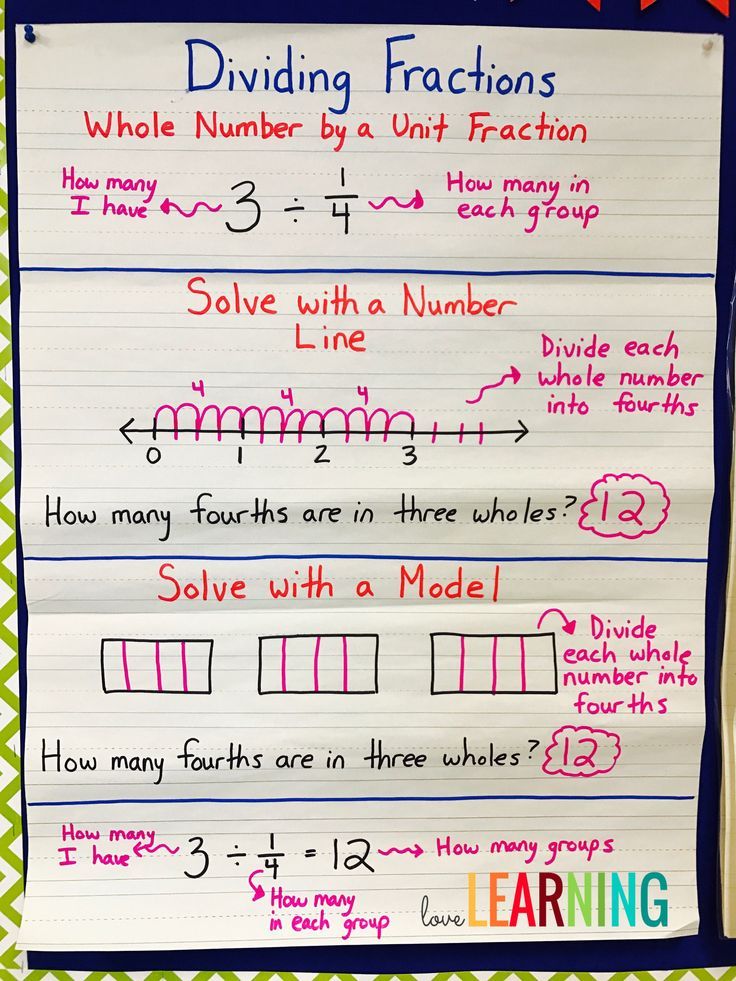
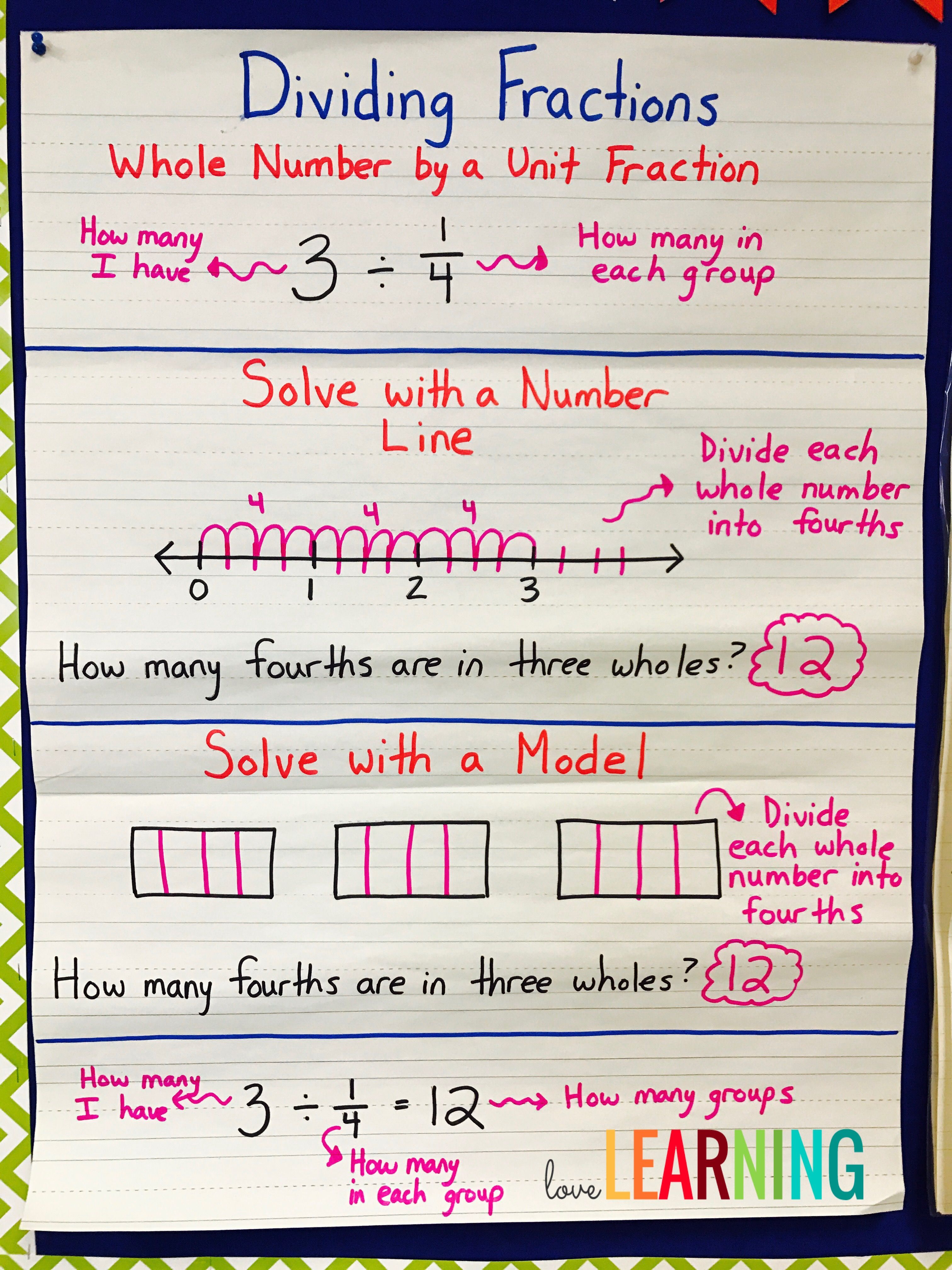
Method 3: Visual Approach

For those who prefer a visual explanation:
- Imagine the whole number divided into equal parts.
- Each part represents one unit of the fraction's denominator.
- How many such parts are needed to reach the whole number?
Method 4: Conceptual Understanding

This method focuses on understanding the underlying relationship between whole numbers and fractions:
- Think about how many of the fraction's denominator fit into the whole number.
- If you're dividing 9 by 1/3, consider that each third of the whole number is 3; thus, you'll need 27 thirds to reach 9.
Method 5: Using a Common Denominator
A slightly more complex but useful approach:
- Convert the whole number to a fraction with a denominator matching the divisor fraction's numerator.
- Now, you're essentially dividing two fractions.
- Follow Method 1 or Method 2 to find your result.
Let’s wrap up the main content by acknowledging the versatility of these methods. Each method offers a different perspective on the same problem, making the concept of dividing whole numbers by fractions accessible to all learning styles. Whether you’re a visual thinker, prefer algebraic transformations, or need a practical, hands-on approach, there’s a method for everyone. Mastering these techniques not only enriches your mathematical toolkit but also deepens your understanding of fractions and their operations.
To conclude, dividing whole numbers by fractions is a straightforward process once you understand the foundational principles. By learning to multiply by the reciprocal, using visual aids, or comprehending the conceptual relationship, you unlock the ability to handle fractions with confidence. This knowledge extends beyond the classroom, providing practical solutions in various daily applications. Keep practicing, and soon, dividing by fractions will be second nature.
Why do we multiply by the reciprocal to divide by a fraction?
+
Multiplying by the reciprocal effectively inverts the fraction, allowing us to turn division into multiplication, which is easier to perform and conceptualize.
Can you divide by a mixed number?

+
Yes, convert the mixed number to an improper fraction first, then apply any of the methods described above.
What if the result isn’t a whole number?

+
The result will often be a mixed number or a fraction. Simplify the result if possible or leave it as a mixed number or improper fraction.
Can these methods be used for real-world problems?

+
Absolutely! These methods are directly applicable in scenarios like dividing recipes, measurements, or scaling quantities in various practical situations.
Is there a visual method to help with understanding?
+Yes, visualizing how many parts of the fraction fit into the whole number can be an effective way to conceptualize the division.