7 Tips for Mastering Mixed Numbers Division Worksheet

In the world of mathematics, especially when dealing with fractions and division, mastering the division of mixed numbers can be a significant challenge for students. However, with the right strategies and a lot of practice, this hurdle can be turned into a valuable skill. This guide aims to simplify the process and provide you with practical tips to conquer the complexities of mixed numbers division worksheet exercises.
Understanding Mixed Numbers
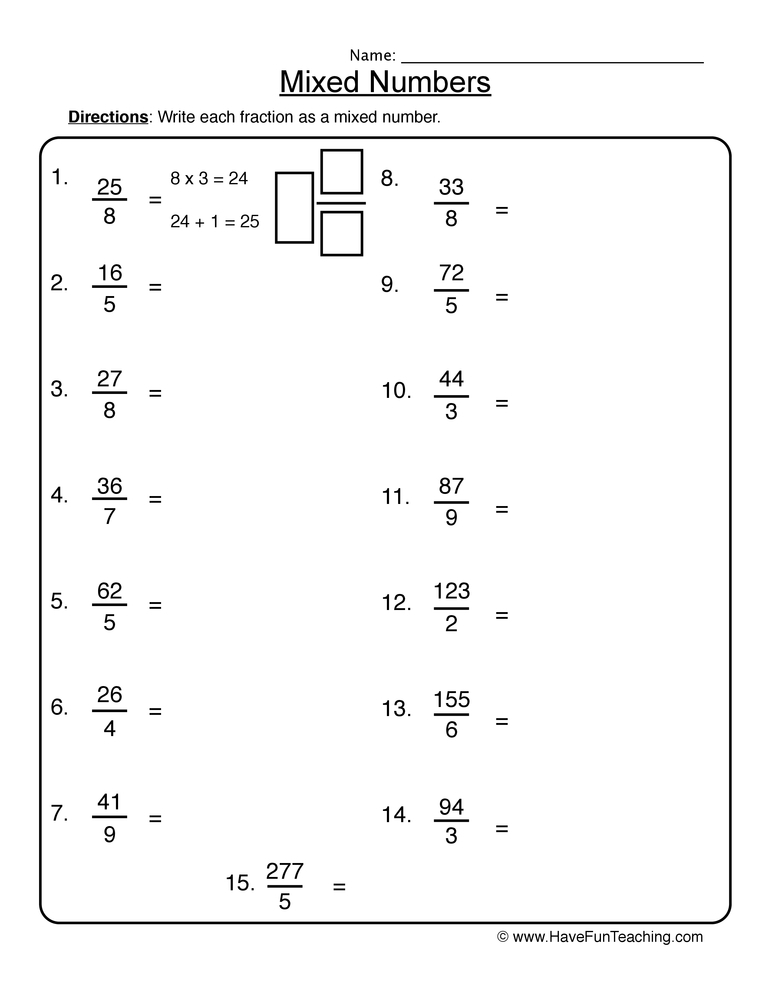

Before diving into division, it’s crucial to understand what a mixed number is. A mixed number is a combination of a whole number and a fraction. For instance, 2 3⁄4 means two whole units and three-quarters of another unit. To work with mixed numbers effectively:
- Convert them to improper fractions: Convert mixed numbers into improper fractions to simplify the division process. For example, 2 3⁄4 can be converted into 11⁄4 by multiplying the whole number by the denominator, then adding the numerator and placing this sum over the original denominator.
Steps to Divide Mixed Numbers
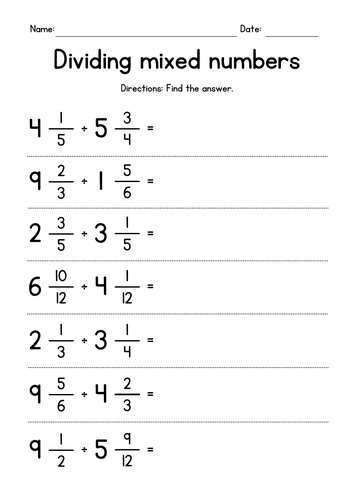
Dividing mixed numbers involves several steps, which become intuitive once understood:
- Convert to Improper Fractions: As previously mentioned, convert each mixed number into an improper fraction.
- Invert the Divisor: Division by a fraction is equivalent to multiplication by its reciprocal. So if you’re dividing by 3⁄5, you would multiply by 5⁄3 instead.
- Multiply: Now, multiply the dividend (first fraction) by the reciprocal of the divisor (the second fraction).
- Simplify: If possible, simplify the resulting fraction. If the answer is an improper fraction, convert it back to a mixed number.
Practicing with Mixed Numbers Division Worksheets


Here are some tips to make your practice sessions more effective:
- Variety of Problems: Use worksheets that cover different levels of difficulty to build your confidence gradually.
- Focus on Speed: Time yourself when solving problems to improve your speed and accuracy.
- Check Work: Always check your work by performing multiplication to ensure the original dividend divided by your answer equals the original divisor.
📝 Note: Ensure your answer is in the simplest form to avoid confusion in further calculations.
Advanced Tips for Mastering Mixed Number Division

Here are some advanced techniques to enhance your skills:
- Memorize Common Reciprocals: Knowing common reciprocals, like 1⁄2, 1⁄4, etc., speeds up the division process.
- Use Estimation: Estimate answers before calculating to gauge the result’s reasonableness.
- Apply Cross-Multiplication: Use cross-multiplication for verification of division by converting the division problem into a ratio for checking purposes.
Practicing Mixed Numbers in Real-Life Scenarios

Applying your skills to real-life problems:
- Recipes: Adjust ingredient quantities by dividing or multiplying mixed numbers.
- Measurements: Convert measurements into mixed numbers for division, like dividing 3 1⁄2 yards of fabric into pieces.
- Finance: Use mixed numbers to calculate proportions of income, investments, or debts.
Overcoming Common Mistakes
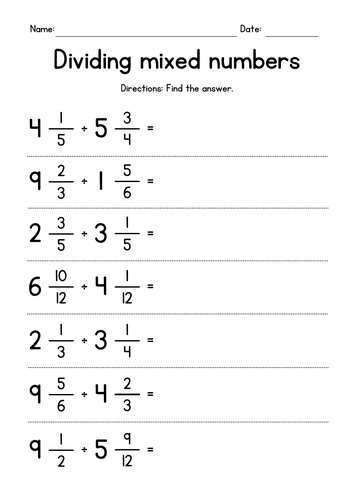
Here are some common errors to watch out for:
- Misconversion of Mixed Numbers: Ensure the conversion from mixed numbers to improper fractions is done correctly.
- Forgetting to Invert: Division requires multiplying by the reciprocal of the divisor; missing this step will lead to incorrect answers.
- Calculation Errors: Take care with arithmetic; small mistakes can lead to significant errors in division.
⚠️ Note: Regular review and practice can help mitigate these common pitfalls.
Leveraging Technology for Practice

Use digital tools to enhance your learning experience:
- Online Worksheets: Websites offer interactive mixed number division worksheets for practice.
- Calculators: Use calculators with fraction capabilities to check your answers.
- Educational Apps: Engage with math apps that feature mixed number division exercises.
By following these tips and engaging regularly with mixed numbers division worksheet, you can turn this challenging topic into one of your strengths. The key is consistent practice, understanding the foundational principles, and applying these concepts in various scenarios to develop a robust grasp of mixed number division.
What is the difference between a mixed number and an improper fraction?

+
A mixed number is a combination of a whole number and a fraction, like 2 3⁄4. An improper fraction, on the other hand, is when the numerator is greater than or equal to the denominator, like 11⁄4. Mixed numbers can be converted to improper fractions for division.
Why is it necessary to convert mixed numbers to improper fractions before dividing?

+
Converting mixed numbers into improper fractions simplifies the division process because dividing by a fraction is equivalent to multiplying by its reciprocal. Mixed numbers would require additional steps to handle the whole number part, making the operation more complex.
How can I check if my mixed number division is correct?

+
After dividing, multiply the result (answer) by the original divisor. If the product equals the original dividend, then your division is correct.