Dividing Fractions by Whole Numbers: Fun Worksheet Guide

Understanding how to divide fractions by whole numbers is a fundamental concept in mathematics, often introducing students to more complex operations involving rational numbers. This guide provides an engaging and easy-to-follow approach through a series of worksheets that not only make learning fun but also reinforce key mathematical principles.
Why Divide Fractions by Whole Numbers?
Dividing fractions by whole numbers is more than just a step in a math curriculum; it's a practical skill with real-life applications:
- Sharing Items: When you need to distribute an item into equal portions.
- Scaling Recipes: Adjusting recipes for different serving sizes.
- Probability Calculations: Understanding chances in more intricate scenarios.
Understanding Division of Fractions

Before diving into the worksheets, here's a quick overview:
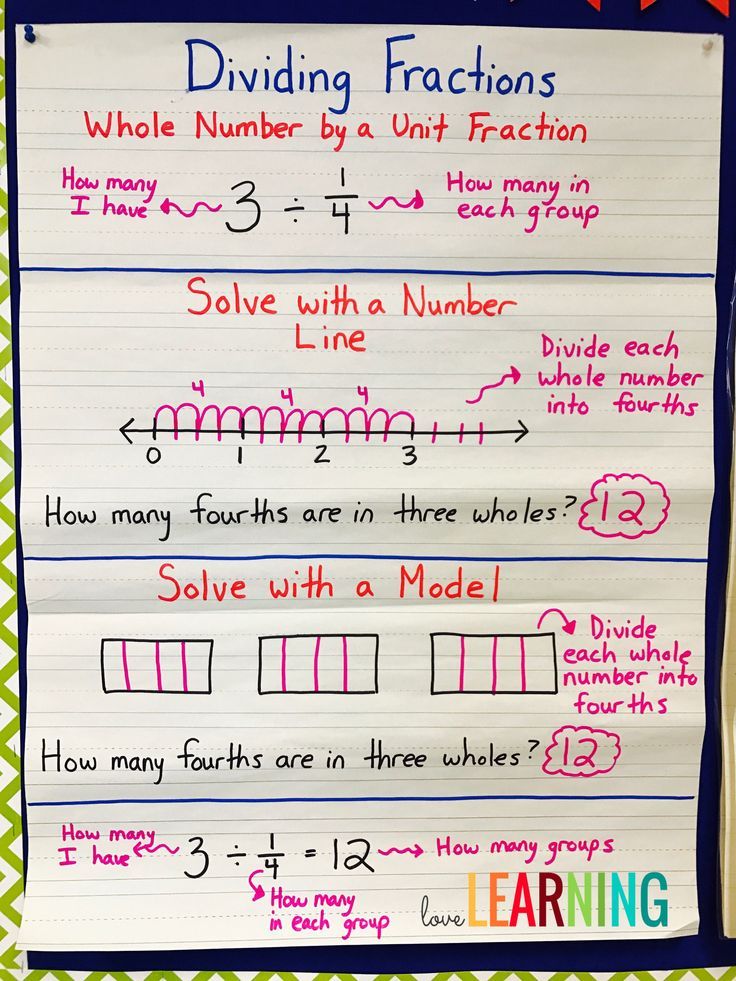
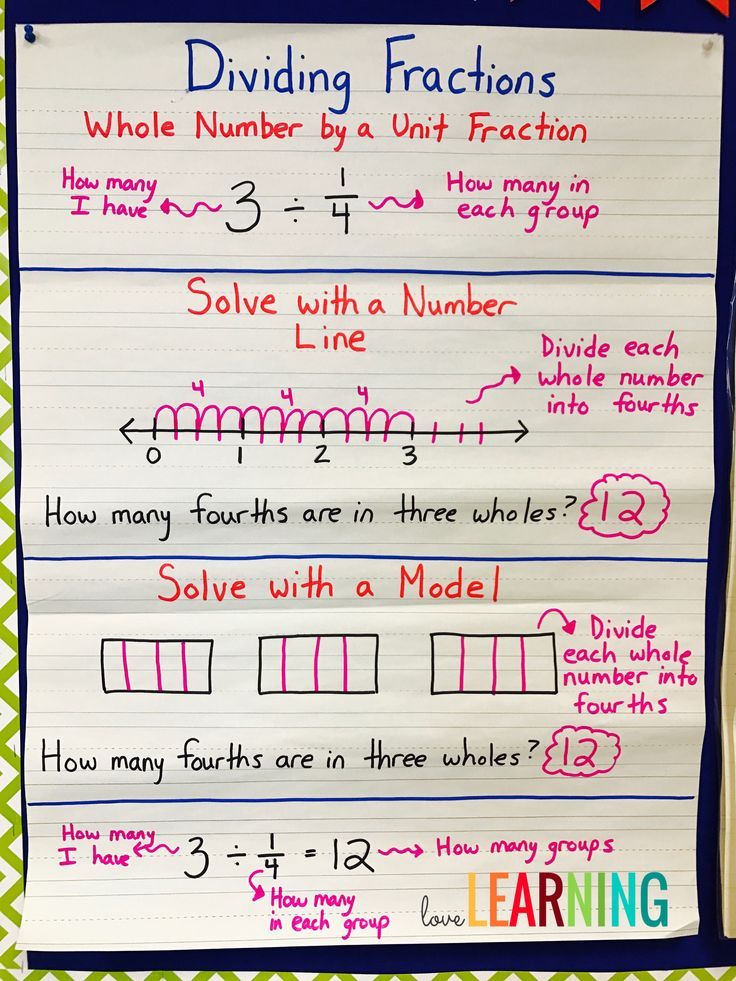
- Reciprocal Rule: To divide a fraction by a whole number, you multiply the fraction by the reciprocal of that number. For example, to divide \frac{1}{4} by 2, you multiply \frac{1}{4} by \frac{1}{2}.
- Visualization: Imagine dividing a pizza into four slices. Now, if you divide those four slices into two equal groups, you get \frac{1}{2} of each slice, or \frac{1}{8} of the whole pizza.
The Worksheet Journey

Worksheet 1: Basic Division

This worksheet introduces students to the concept with simple, easy-to-visualize examples:
| Problem | Answer |
|---|---|
| (\frac{3}{5}) ÷ 2 = | (\frac{3}{10}) |
| (\frac{2}{7}) ÷ 3 = | (\frac{2}{21}) |

Students solve these basic problems, which help them understand how to find the reciprocal and multiply.
Worksheet 2: Intermediate Practice

Here, students encounter slightly more challenging scenarios:
- (\frac{5}{9}) ÷ 4
- (\frac{7}{11}) ÷ 5
📘 Note: Encourage students to convert these division problems into multiplication for easier computation.
Worksheet 3: Real-World Applications

This worksheet applies division of fractions to real-world problems:
- A group of 15 people shares (\frac{3}{4}) of a cake. How much cake does each person get?
- If (\frac{1}{3}) of a field is split into 5 equal parts, how much of the field is each part?
Tips for Teaching and Learning

- Visualization: Use visual aids like pie charts or fraction bars to make the concept tangible.
- Real-World Examples: Connect the math to everyday situations to foster practical understanding.
- Peer Learning: Encourage students to explain their solutions to peers for better retention.
- Practice: Repetition through varied exercises helps solidify the understanding of how division works with fractions.
Summing Up

This guide has walked you through a practical and engaging approach to dividing fractions by whole numbers. Through step-by-step worksheets, visual aids, and real-world applications, students can not only grasp this mathematical concept but also enjoy the process. The key is to make the learning journey interactive, intuitive, and relevant to daily life, ensuring that students not only understand but also appreciate the utility of fractions in different contexts.
Why do we multiply by the reciprocal when dividing fractions?
+
Multiplying by the reciprocal essentially turns the division problem into multiplication, which is an easier operation to perform with fractions. This is because division by a number is equivalent to multiplying by its reciprocal.
Can you divide a fraction by zero?
+
No, division by zero is undefined because zero does not have a reciprocal, and you would be attempting to find an infinite number of parts, which isn’t mathematically possible.
How does dividing fractions by whole numbers differ from multiplying fractions?

+
While multiplying fractions involves multiplying numerators and denominators, dividing by a whole number requires converting that whole number into a fraction with a numerator of 1 and then multiplying by its reciprocal. This shifts the denominator in a way that effectively divides the original fraction.