5 Engaging Ways to Teach Dividing Fractions With Models

When it comes to teaching students about dividing fractions with models, educators face the challenge of turning what can be an abstract concept into a tangible and understandable lesson. Models offer a visual and practical approach that can illuminate the otherwise complex process of dividing fractions. Here are five engaging methods to help your students grasp this concept effectively.
1. Fraction Bars for Dividing Fractions
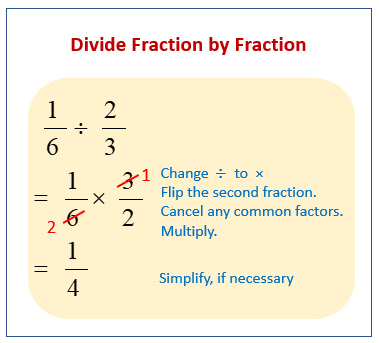
Fraction bars are among the most straightforward and visually intuitive models for division of fractions:
- Introduce the Concept: Begin by explaining that dividing by a fraction is equivalent to multiplying by its reciprocal. A visual representation helps students to understand why this works.
- Set Up the Problem: Use fraction bars to represent the problem. For instance, to divide 3⁄4 by 1⁄2, you’ll need fraction bars representing these fractions.
- Model the Division: Demonstrate how many times 1⁄2 can fit into 3⁄4 by aligning the bars. Since 3⁄4 is larger than 1⁄2, you can fit 1⁄2 three times into 3⁄4, but with a remaining fraction.
- Introduce the Reciprocal: Explain that flipping the second fraction (1⁄2 becomes 2⁄1) will make the calculation more straightforward, showing that 3⁄4 x 2⁄1 equals 6⁄4 or 1 1⁄2.
- Practice: Allow students to practice with their own fraction bars, setting up various problems and finding the answers through visual modeling.
📘 Note: Fraction bars should be color-coded or differently patterned to avoid confusion, and having multiple sets per student can facilitate different examples.
2. Number Lines for Dividing Fractions

Number lines can provide a linear visual model for dividing fractions:
- Set Up: Draw a number line for the numerator (the dividend) and divide it into parts representing the denominator of the divisor. For example, for 3⁄4 divided by 1⁄2, draw a line from 0 to 1 and mark it off into two halves.
- Divide: Mark off how many times 1⁄2 fits into 3⁄4. In this case, 3⁄4 is 3 out of 4 equal parts on the line, and since you divide by 1⁄2, it means you need to see how many half units fit into these 3 parts.
- Answer: Each unit of 1⁄2 fits twice, leading to a total of 3 x 2, which equals 6 halves. This can be simplified to 1 1⁄2.
- Visual Impact: This visual aid helps students understand that division by fractions is an inverse operation to multiplication by its reciprocal.
3. Area Models for Dividing Fractions

Area models offer a geometric approach to understanding fraction division:
- Setup: Create a rectangle with one side representing the numerator of the dividend and the other the denominator of the divisor. For 3⁄4 ÷ 1⁄2, use a 4 x 3 grid for 3⁄4 and divide it into 2 rows for 1⁄2.
- Area Division: Determine how many parts of 1⁄2 fit into the 3⁄4 rectangle. In this case, each row contains 3⁄4, and since you need to divide by 1⁄2, you’ll find that there are 3 such rectangles, leading to 3 x 2 = 6 halves.
- Explanation: This model visually reinforces the concept of division as the number of smaller parts fitting into the larger whole.
4. Using Real-Life Contexts for Dividing Fractions

Connecting abstract math to real-life scenarios can make division of fractions more relatable:
- Contextualize: Create stories or scenarios where dividing fractions is a natural solution. For example, dividing a pizza in half (1⁄2) when you have 3⁄4 of it left.
- Visualize: Use models like paper, a pizza model, or manipulative toys to represent the fractions.
- Problem Solve: Ask students to find how many equal parts the 3⁄4 pizza can be divided into if each person gets 1⁄2 of it.
- Relatable Outcome: Students can see that if you have 3⁄4 pizza and divide by 1⁄2, you get 1.5 pizzas, which makes more sense in a real-life context.
💡 Note: Incorporating students’ interests in your examples can increase engagement and motivation to learn.
5. Interactive Digital Tools for Dividing Fractions

Digital tools can make learning dynamic and interactive:
- Digital Models: Use online manipulatives or educational software that allows students to drag and drop fraction pieces. This provides a kinesthetic approach to learning.
- Step-by-Step Guides: Digital tools can guide students through the division process, showing each step visually and interactively.
- Practice: Offer virtual practice with instant feedback, which helps reinforce the concept.
- Accessibility: Ensure that the digital resources are accessible to all students, including those with visual impairments, through appropriate design and audio descriptions.
By using these five engaging methods, educators can demystify the division of fractions, making it more approachable for students. Models help create a bridge between abstract mathematical concepts and real-world understanding. Whether through hands-on manipulatives like fraction bars, number lines, or area models, or through interactive digital tools, students can solidify their comprehension of this fundamental operation. Each method fosters a unique pathway to grasp the idea that dividing by a fraction is akin to multiplying by its inverse, a cornerstone of mathematical literacy.
What if my students are struggling with basic fraction concepts?

+
Start with more concrete models like fraction circles or pie models. Ensure they understand the concept of a whole, halves, thirds, etc., before moving to division of fractions.
Can these models be used for other fraction operations?

+
Absolutely. These models are versatile and can be adapted for addition, subtraction, and multiplication of fractions as well, providing a holistic approach to learning fractions.
How can I assess students’ understanding using these models?

+
You can give students problems and ask them to use fraction bars or number lines to solve and explain their solutions. Monitor their ability to represent fractions correctly and accurately perform the division.
Are there resources for creating or finding these models?

+
Yes, online educational marketplaces, educational blogs, and teaching resources often provide printable fraction manipulatives, digital tools, or detailed instructions on how to create your own.