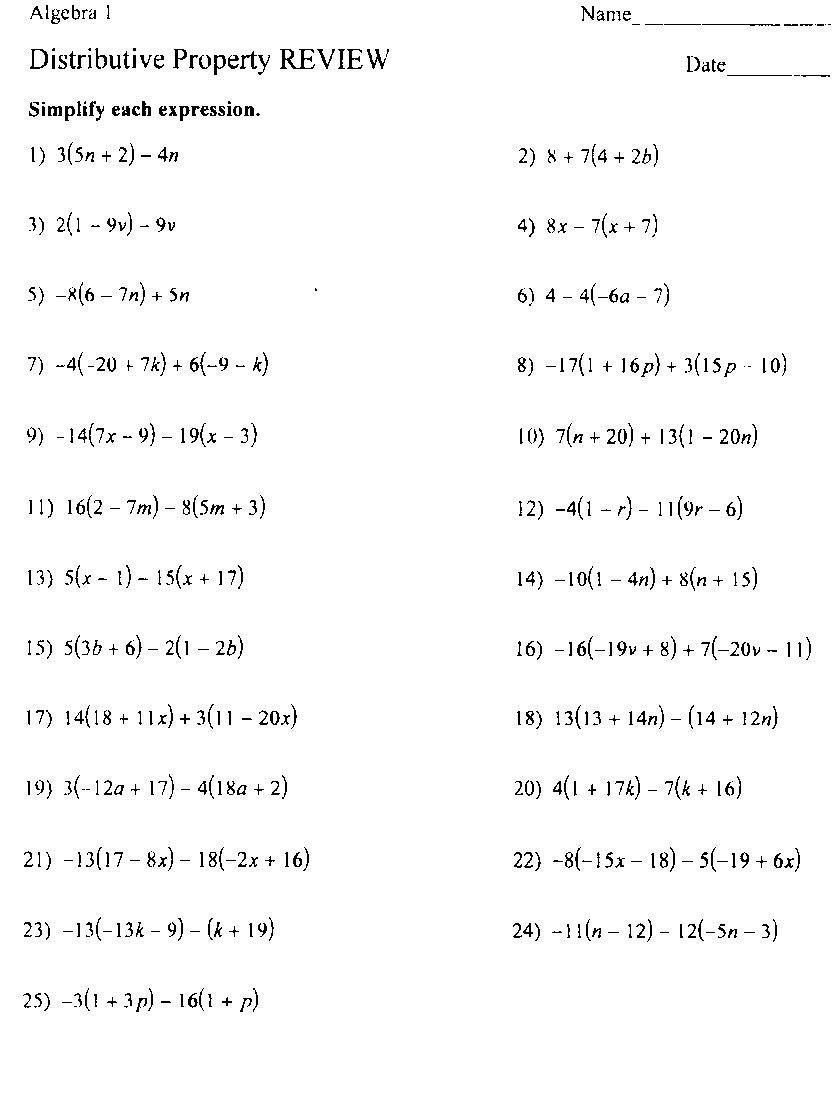
Master Distributive Property with Free Worksheets

In the realm of mathematics, particularly in algebra, mastering the distributive property is crucial for simplifying expressions, solving equations, and understanding how numbers interact. This property not only lays the foundation for algebraic manipulation but also enhances a student's ability to handle more complex mathematical operations. In this extensive blog post, we'll delve deep into the distributive property, its applications, and how you can sharpen your skills through practice with free worksheets.
What is the Distributive Property?

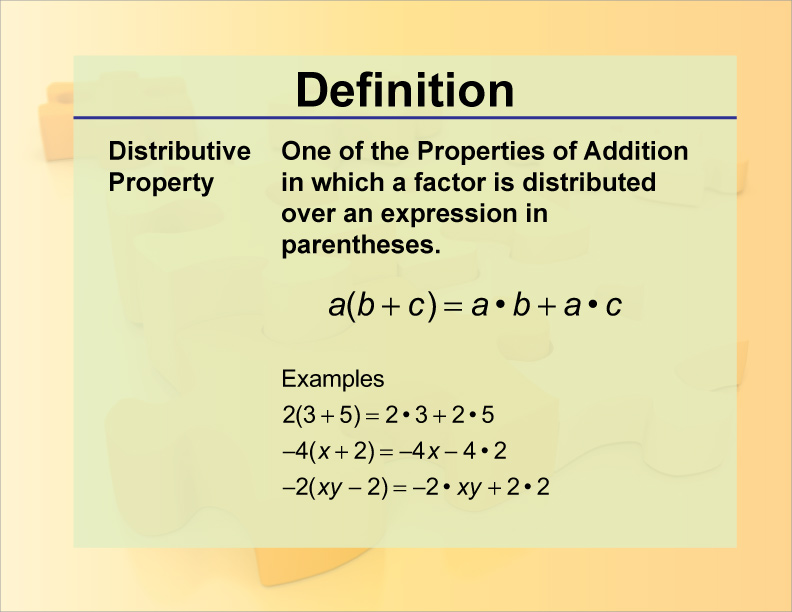
The distributive property is a fundamental rule that states:
- a(b + c) = ab + ac
This property allows the term outside the parentheses to be multiplied by each term inside the parentheses separately. Here’s a breakdown:
- If you have a number or variable a multiplied by a group of terms inside parentheses (b + c), you can distribute the a to each term inside, giving you the result of ab + ac.
Why is the Distributive Property Important?

Understanding the distributive property is vital because:
- It helps in simplifying expressions, making them easier to solve.
- It forms the basis for polynomial multiplication, factoring, and solving equations.
- It allows for mental calculations, which is handy in everyday life and quick checks.
💡 Note: The distributive property applies to addition and subtraction, making it versatile in algebra.
Examples of the Distributive Property in Action
Let’s explore some examples to illustrate how the distributive property works:
-
Example 1: 3(4 + 5) = 3 * 4 + 3 * 5 = 12 + 15 = 27
-
Example 2: 6(x - 2) = 6x - 12
-
Example 3: -2(5y + 3) = -10y - 6
👉 Note: Note that the sign changes when distributing a negative value.
Using Distributive Property Worksheets to Practice

The best way to master any concept in mathematics, including the distributive property, is through consistent practice. Here are steps and strategies to get the most out of using free distributive property worksheets:
-
Choose the Right Level

Select worksheets that match your current skill level. If you’re just starting, choose problems involving basic numbers before moving to variables and more complex terms.
-
Understand the Setup

Look at the instructions or example problems provided on the worksheet to ensure you understand how to use the property correctly.
-
Practice Mindfully

Don’t just solve the problems mechanically; understand each step:
- Identify the term to be distributed.
- Distribute it to each term inside the parentheses.
- Combine like terms if necessary.
-
Check Your Work

Use answer keys or redo problems to check your accuracy. Mistakes help in learning, so be patient and persistent.
✨ Note: Regular practice with worksheets can reinforce the concept and increase your speed and accuracy.
Worksheets for Different Skill Levels
| Skill Level | Description | Example |
|---|---|---|
| Beginner | Worksheets with simple numbers and basic variables. | 2(x + 3) |
| Intermediate | Includes mixed operations or negative values. | -4(2y - 5) |
| Advanced | Complex polynomials and binomial distribution. | (x + 2)(x - 3) |

Each level presents its own set of challenges, helping to build a comprehensive understanding of the distributive property.
Maximizing Your Learning with Free Resources

Here are ways to make the most out of the freely available educational resources:
- Track Your Progress: Keep a log or use apps to monitor your improvement over time.
- Join Study Groups: Collaborate with peers to discuss problems and learn from different perspectives.
- Teach Others: Explain the distributive property to someone else to solidify your own understanding.
- Use Online Platforms: Websites like Khan Academy or OpenStax offer interactive lessons alongside worksheets.
In wrapping up our journey through the distributive property and its practice with free worksheets, remember that this property is not just a rule but a cornerstone of algebra. By understanding and applying the distributive property, you're unlocking a world of mathematical clarity, from simplifying basic expressions to tackling complex polynomials. Each example we've discussed showcases how this property can make seemingly difficult problems more manageable. Worksheets tailored to different skill levels serve as your training ground, fostering an environment where errors become learning opportunities. Practice, as emphasized, is not just about repetition but about deepening your comprehension and honing your problem-solving skills.
Can the distributive property be used in division?

+
Yes, you can also distribute division when dividing a sum or difference by a number. For example, (a + b) ÷ c = a ÷ c + b ÷ c.
How do you know when to apply the distributive property?

+
Look for expressions where you have multiplication or division next to an addition or subtraction operation. If there’s a term outside the parentheses or if you see a sum or difference inside, consider using the property to simplify or solve the expression.
What if I don’t want to use the distributive property?

+
While you don’t always have to use the distributive property, it’s often the most efficient way to solve or simplify algebraic expressions. However, other methods like factoring or expanding can also be used depending on the situation.