5 Ways to Solve Direct and Inverse Variation Problems

Many students find the concept of direct and inverse variation both intriguing and challenging. Variations are essentially mathematical relationships that explain how two quantities interact with each other. Here are five effective ways to solve these problems, ensuring a deeper understanding and practical application:
Understanding the Basics of Variation
Before diving into the methods, it’s crucial to understand what direct and inverse variations mean:
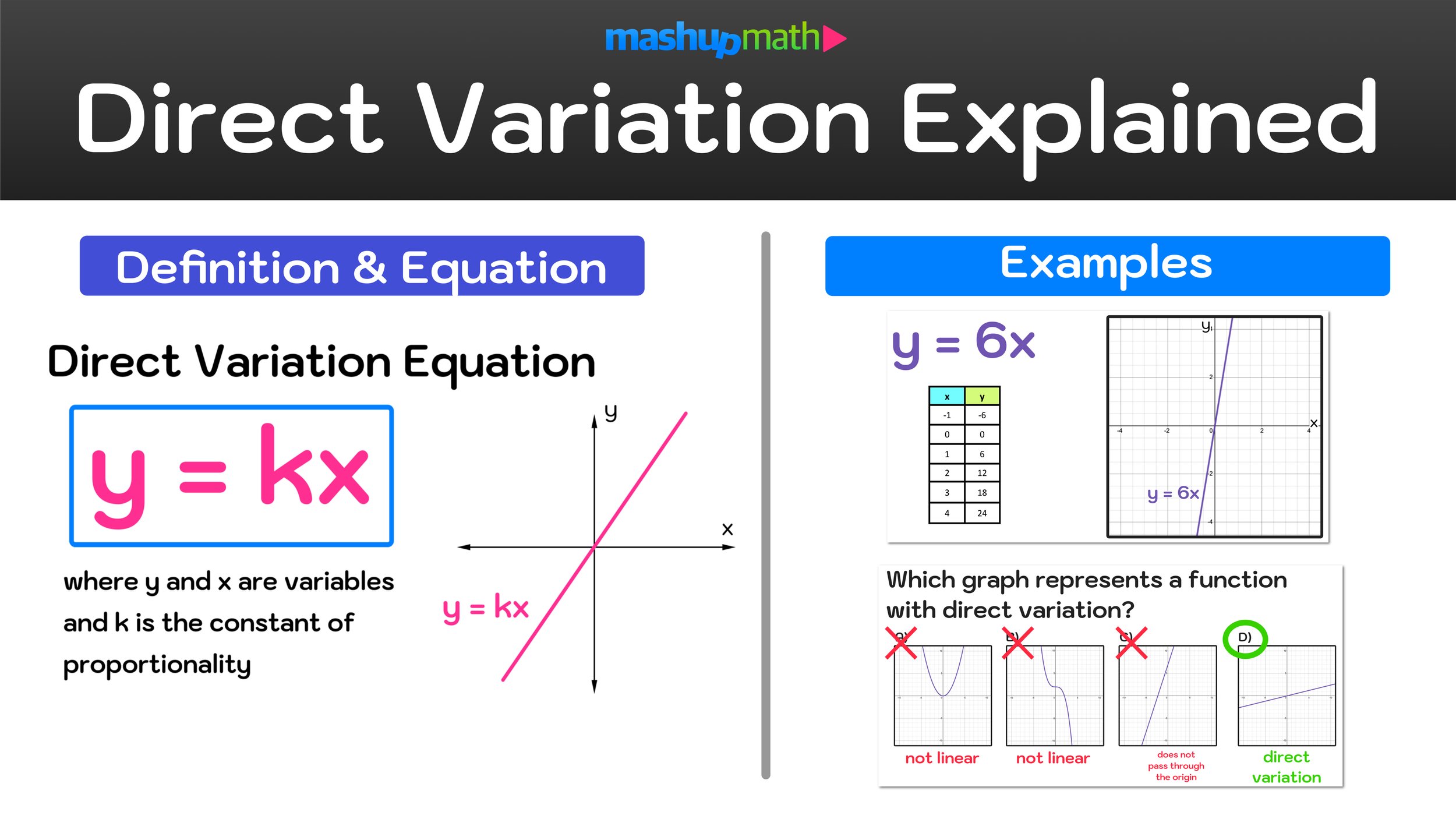
- Direct Variation: Two variables y and x are said to vary directly if their ratio is constant, which can be written as y = kx where k is the constant of proportionality.
- Inverse Variation: Here, if y and x vary inversely, the product of the two variables is constant. This means xy = k or y = k/x.
1. Identify the Type of Variation

The first step in solving any variation problem is to identify whether you are dealing with direct or inverse variation:
- Look for statements like “is proportional to,” “varies directly as,” or “inversely proportional to.”
- Use context clues from word problems or graphs to determine the relationship.
🔍 Note: Noticing small details in the problem statement can significantly influence which type of variation you are dealing with.
2. Use the Variation Formula

Once you’ve identified the type of variation, apply the appropriate formula:
- For Direct Variation: y = kx or k = y/x.
- For Inverse Variation: xy = k or y = k/x.
By solving for k, you establish a key piece of information needed to find missing variables.
3. Set Up a Proportion

If you know two sets of related values, you can set up a proportion to find an unknown variable:
| Direct Variation | Inverse Variation |
|---|---|
| y1/x1 = y2/x2 | x1y1 = x2y2 |

This method works by assuming that the constant of proportionality k is the same across all pairs of related values.
4. Plug in Known Values

When dealing with variations, often you are given one set of data and asked to find another. Here’s how you do it:
- Plug in the known values into the variation equation to solve for k.
- Once you have k, use it to find the unknown variable in the next set of values.
This technique ensures that your calculations are accurate and based on real data.
5. Graphical Representation

Graphing is an excellent visual aid for understanding variations:
- In direct variation, the graph will yield a straight line through the origin with a slope of k.
- For inverse variation, the graph will form a hyperbola, never touching the axes, as x and y get closer to zero, y becomes very large or very small.
This method provides a visual confirmation of the mathematical relationship, making complex data more digestible.
By using these five methods, students and professionals can master the art of solving direct and inverse variation problems. Each technique offers a different approach to understanding and applying the concepts of proportionality. Mastering variations not only helps in math but also in various real-life scenarios where relationships between variables are crucial. It's about seeing the pattern, understanding the logic behind it, and then applying that logic to solve problems effectively.
What is the difference between direct and inverse variation?
+
In direct variation, the ratio of two variables remains constant (y/x = k). In inverse variation, the product of two variables remains constant (xy = k). This means that as one variable increases in direct variation, the other increases proportionally; whereas, in inverse variation, as one variable increases, the other decreases proportionally.
How can I identify if two quantities vary directly or inversely?

+
Look for key phrases like “proportional to” or “varies directly as” for direct variation, or “inversely proportional to” for inverse variation. Also, observe the behavior of the variables: if they increase together or one decreases as the other increases.
Why is it important to solve for the constant of proportionality?

+
The constant of proportionality, k, gives us the fundamental relationship between the variables. Knowing k allows us to predict one variable’s value when the other is known, making problem-solving in various fields like physics, chemistry, and engineering easier and more precise.