Coulombic Attraction Worksheet Answers: Simplified Solutions for Students

Understanding the forces at play between charged particles is a cornerstone of studying chemistry and physics. Coulombic attraction, defined by Coulomb's Law, is fundamental to explaining these interactions. This post aims to demystify Coulombic attraction by offering comprehensive solutions to common worksheet problems, guiding students through the calculations, and ensuring an intuitive grasp of the underlying principles.

What is Coulombic Attraction?

At its core, Coulombic attraction is the electrostatic force between two charged particles, which can either be attractive or repulsive. The magnitude of this force depends on the product of their charges and inversely on the square of the distance between them. Here’s the formula:

[ F = k_e \frac{{|q_1 \cdot q_2|}}{{r^2}} ]

• F is the magnitude of the Coulomb force (Newtons)
• k_e is Coulomb's constant (8.99 × 10⁹ Nm²/C²)
• q₁, q₂ are the charges (Coulombs)
• r is the distance between the centers of the charges (meters)

🔍 Note: The force is positive when the charges attract each other (unlike charges) and negative when they repel (like charges).

Worksheet Solutions

Problem 1

Calculate the Coulombic attraction between two particles with charges of -2 C and +3 C, separated by a distance of 10 meters.

Let’s apply the Coulombic force formula:

[ F = k_e \frac{{|(-2) \cdot (+3)|}}{{10^2}} ] [ F = 8.99 \times 10^9 \times \frac{6}{100} ] [ F \approx 5.39 \times 10^8 \text{ N} ]

🔍 Note: Here, the charges are opposite, so the force is attractive.

Problem 2

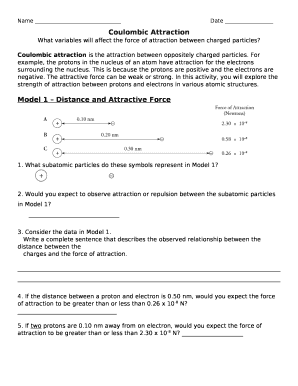
Given two equally charged ions with q₁ = q₂ = +1.6 × 10^-19 C, placed 1 nm apart, find the force between them.

Using the formula:

[ F = k_e \frac{{|(+1.6 \times 10^{-19})^2|}}{{1 \times 10^{-9}}^2} ] [ F = 8.99 \times 10^9 \times \frac{(1.6 \times 10^{-19})^2}{1 \times 10^{-18}} ] [ F \approx 2.31 \times 10^{-9} \text{ N} ]

🔍 Note: Since the charges are the same, this force is repulsive.

Problem 3

What would be the electric force between an electron and a proton when they are 0.53 × 10^-10 meters apart?

The charges for an electron and a proton are -1.6 × 10^-19 C and +1.6 × 10^-19 C respectively.

[ F = k_e \frac{{|(-1.6 \times 10^{-19}) \cdot (+1.6 \times 10^{-19})|}}{{0.53 \times 10^{-10}}^2} ] [ F \approx 8.99 \times 10^9 \times \frac{2.56 \times 10^{-38}}{2.809 \times 10^{-20}} ] [ F \approx 8.24 \times 10^{-8} \text{ N} ]

🔍 Note: The force here represents the strong attractive force between an electron and a proton in a hydrogen atom.

Problem 4

If the force between two charges is 2.5 × 10^-10 N when they are 2 meters apart, determine the product of their charges.

Rearranging the Coulomb’s Law for the product of charges:

[ |q_1 \cdot q_2| = \frac{F \cdot r^2}{k_e} ] [ |q_1 \cdot q_2| = \frac{(2.5 \times 10^{-10}) \cdot 2^2}{8.99 \times 10^9} ] [ |q_1 \cdot q_2| \approx 1.11 \times 10^{-20} \text{ C}^2 ]

In this final section, we've dissected several problem scenarios to demonstrate how Coulombic attraction works in practice. From these examples, we understand that the force's strength can be manipulated through charge magnitude, distance, or even the medium in which the charges exist. It's these interactions, often at play on microscopic levels, that dictate so many of the natural phenomena we observe daily.

Overall, mastering the concepts of Coulombic attraction not only facilitates a deeper comprehension of electromagnetism but also serves as a cornerstone for understanding molecular bonding, conductivity, and many other physical and chemical properties. It's an exquisite example of how simple mathematical relationships can unravel the complexities of our universe.