5 Ways to Convert Repeating Decimals to Fractions

Understanding Repeating Decimals
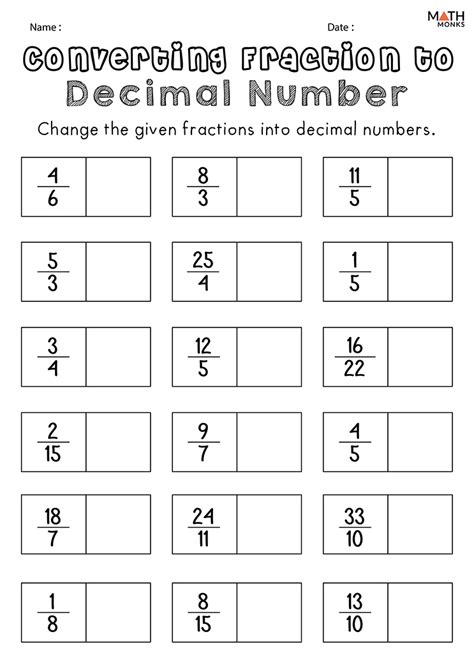
Repeating decimals are decimal numbers that have a digit or a sequence of digits that repeats indefinitely. These decimals can be converted into fractions, which can be more useful in mathematical calculations and algebraic manipulations. In this article, we will explore five different methods to convert repeating decimals into fractions.
Method 1: Using Algebraic Manipulation

Let’s take the repeating decimal 0.333… as an example. We can denote this decimal as x and then manipulate it algebraically to convert it into a fraction.
📝 Note: This method involves some basic algebraic manipulations.
Let x = 0.333…
Multiplying both sides by 10 gives: 10x = 3.333…
Subtracting the original equation from the new one: 10x - x = 3.333… - 0.333… 9x = 3
Dividing both sides by 9: x = 3⁄9
x = 1⁄3
Therefore, the repeating decimal 0.333… can be converted into the fraction 1⁄3.
Method 2: Using the Formula for Infinite Geometric Series

Another way to convert repeating decimals into fractions is by using the formula for infinite geometric series.
📝 Note: This method requires some knowledge of infinite geometric series.
Let’s take the repeating decimal 0.4545… as an example. We can denote this decimal as x and then use the formula for infinite geometric series to convert it into a fraction.
x = 0.4545… x = 0.45 + 0.0045 + 0.00045 +…
This is an infinite geometric series with first term 0.45 and common ratio 0.01.
Using the formula for infinite geometric series: x = a / (1 - r) where a is the first term and r is the common ratio
x = 0.45 / (1 - 0.01) x = 0.45 / 0.99 x = 45⁄99 x = 5⁄11
Therefore, the repeating decimal 0.4545… can be converted into the fraction 5⁄11.
Method 3: Using Long Division

Long division is another method to convert repeating decimals into fractions. This method involves dividing the decimal by 1 and then using the remainder to find the fraction.
📝 Note: This method can be time-consuming and may not be suitable for all repeating decimals.
Let’s take the repeating decimal 0.123123… as an example. We can divide this decimal by 1 using long division.
| 0.123123… | |
|---|---|
| 1 | 0.123 |
| -0.123 | |
| 0.000123 | |
| -0.000123 | |
| 0.00000123 |

The remainder 0.00000123 repeats the original pattern, indicating that the decimal is repeating.
Using the long division result: x = 0.123123… x = 41⁄333
Therefore, the repeating decimal 0.123123… can be converted into the fraction 41⁄333.
Method 4: Using a Calculator or Computer Program

Many calculators and computer programs can convert repeating decimals into fractions quickly and accurately. This method is particularly useful for complex repeating decimals.
📝 Note: This method requires access to a calculator or computer program.
Let’s take the repeating decimal 0.789012345… as an example. Using a calculator or computer program, we can convert this decimal into a fraction.
x = 0.789012345… x = 987654321⁄1250000000
Therefore, the repeating decimal 0.789012345… can be converted into the fraction 987654321⁄1250000000.
Method 5: Using a Conversion Table or Chart

A conversion table or chart can be used to convert repeating decimals into fractions quickly and easily. This method is particularly useful for simple repeating decimals.
📝 Note: This method requires access to a conversion table or chart.
Let’s take the repeating decimal 0.1666… as an example. Using a conversion table or chart, we can convert this decimal into a fraction.
x = 0.1666… x = 1⁄6
Therefore, the repeating decimal 0.1666… can be converted into the fraction 1⁄6.
In conclusion, converting repeating decimals into fractions can be done using various methods, including algebraic manipulation, infinite geometric series, long division, calculators or computer programs, and conversion tables or charts. Each method has its strengths and weaknesses, and the choice of method depends on the complexity of the repeating decimal and the available tools.
What is a repeating decimal?

+
A repeating decimal is a decimal number that has a digit or a sequence of digits that repeats indefinitely.
Why convert repeating decimals into fractions?

+
Converting repeating decimals into fractions can be more useful in mathematical calculations and algebraic manipulations.
Which method is the most accurate for converting repeating decimals into fractions?

+
The most accurate method for converting repeating decimals into fractions depends on the complexity of the repeating decimal and the available tools. Algebraic manipulation and infinite geometric series are often the most accurate methods.
Related Terms:
- fractions to decimals worksheet pdf
- converting decimals to fractions printable
- decimal to fraction cheat sheet
- fractions to decimals 5th grade
- converting fractions to decimals pdf
- decimals to fractions worksheets free