5 Tips for Converting Fractions to Decimals Easily
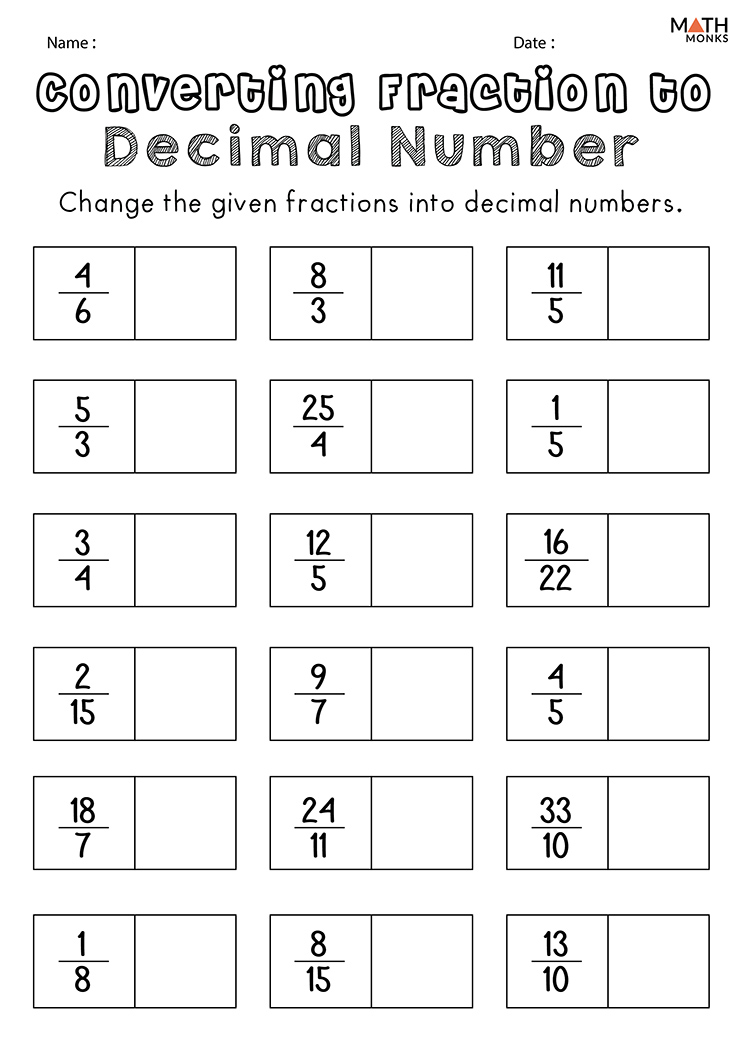
Converting fractions to decimals can often seem daunting, especially when you encounter complex fractions or need to do it without a calculator. However, with the right techniques, this mathematical process can become straightforward and even enjoyable. Here, we'll explore five effective tips that will make converting fractions to decimals easy for students, educators, or anyone looking to master basic arithmetic.
1. Understanding the Decimal Point

Decimals are essentially a different representation of fractions. Every fraction can be expressed as a decimal, and understanding how decimal points work is the first step:
- Decimal Representation: A decimal number like 0.75 is equivalent to the fraction 75⁄100 or simplified to 3⁄4. Understanding this equivalence can clarify the relationship between fractions and decimals.
- Place Value: Know that the position of each digit after the decimal point represents a power of 10. The tenths place is 10^-1, the hundredths place is 10^-2, and so forth.
2. Long Division Method

The long division method is a traditional approach to convert fractions to decimals:
- Set up the fraction as a division problem where the numerator (top number) is divided by the denominator (bottom number).
- Continue dividing until you either get a remainder of zero or reach a sufficient level of precision.
🎓 Note: If the decimal repeats indefinitely, represent it with a bar over the repeating digits, like 0.\overline{3} for 1⁄3.
3. Utilize Multiples of 10

When dealing with common fractions where the denominator is a multiple of 10, converting to a decimal can be very simple:
- If the denominator is 10, you simply move the decimal point of the numerator one place to the left.
- For denominators that are multiples of 10, like 100 or 1000, adjust the decimal point accordingly.
4. Conversion Using a Calculator

While learning the manual methods is essential for understanding the concept, calculators can be a handy tool for quick conversion:
- Enter the numerator, press the division sign, then enter the denominator, and press equals.
- Remember that repeated decimals should still be recognized even with calculator assistance.
💡 Note: It’s good to double-check manual methods with a calculator for verification.
5. Simplify Fractions Before Conversion

Before converting fractions to decimals, simplifying can often make the process easier:
- Find the greatest common divisor (GCD) of the numerator and denominator, and divide both by this GCD.
- This simplification reduces the numbers you have to work with, making conversion quicker and less error-prone.
In summary, converting fractions to decimals involves understanding the fundamental principles of decimals, utilizing basic arithmetic operations like long division, making use of multiples of 10 for simpler conversions, employing technology as a tool for efficiency, and simplifying fractions beforehand. These methods not only simplify the process but also build a deeper understanding of number systems, enhancing overall mathematical proficiency.
Why do some fractions have repeating decimals?
+
Fractions with denominators that are not factors of any power of 10 can produce repeating decimals. This occurs because the fraction’s denominator and 10 do not share common factors other than 1, leading to non-terminating decimal expansions.
How do I know if my decimal conversion is correct?

+
You can verify your decimal conversion by converting it back to a fraction or using a calculator to check. If the original fraction and the converted decimal are equivalent when you perform these checks, your conversion is correct.
Is there a quick method for converting mixed numbers?
+
To convert a mixed number to a decimal, first convert the fraction part to a decimal, then add the whole number to this decimal. For example, for 2 3⁄4, convert 3⁄4 to 0.75, then add 2 to get 2.75.