Mixed Numbers to Improper Fractions: Easy Conversion Worksheet

Why Convert Mixed Numbers to Improper Fractions?

Understanding how to convert mixed numbers into improper fractions is a fundamental mathematical skill with practical applications in numerous areas including cooking, engineering, and finance. This transformation can simplify mathematical operations and provide a clearer way to visualize data or measurements.
Understanding Mixed Numbers and Improper Fractions

A mixed number consists of a whole number combined with a proper fraction, for instance, 3 1/2. Conversely, an improper fraction is one where the numerator (top number) is greater than or equal to the denominator (bottom number), like 7/2. Here’s a simple way to think about the differences:
- Mixed Number: 3 1/2, where 3 is the whole number and 1/2 is the fraction.
- Improper Fraction: 7/2, which represents 7 parts out of 2.
Step-by-Step Conversion Process

1. Identify the Whole Number and Fraction

Let’s say you have the mixed number 5 2⁄3:
- Whole Number: 5
- Numerator of Fraction: 2
- Denominator of Fraction: 3
2. Multiply the Whole Number by the Denominator

Multiply the whole number (5) by the denominator (3):
5 x 3 = 15
3. Add the Result to the Numerator

Add the result (15) to the numerator (2) of the fraction:
15 + 2 = 17
4. Write the Improper Fraction

Place the new numerator (17) over the original denominator (3):
The improper fraction is now 17⁄3.
🔍 Note: When working with large numbers, keep calculations tidy by keeping denominators in mind before making computations.
Why This Conversion Matters

The ability to convert mixed numbers into improper fractions allows for:
- Easy Addition and Subtraction: It streamlines the process of performing basic arithmetic operations with fractions.
- Simplification: Improper fractions can often be reduced to their simplest form, reducing the complexity of mathematical expressions.
- Comparison: Comparing and ordering fractions becomes more straightforward with improper fractions.
Practical Examples
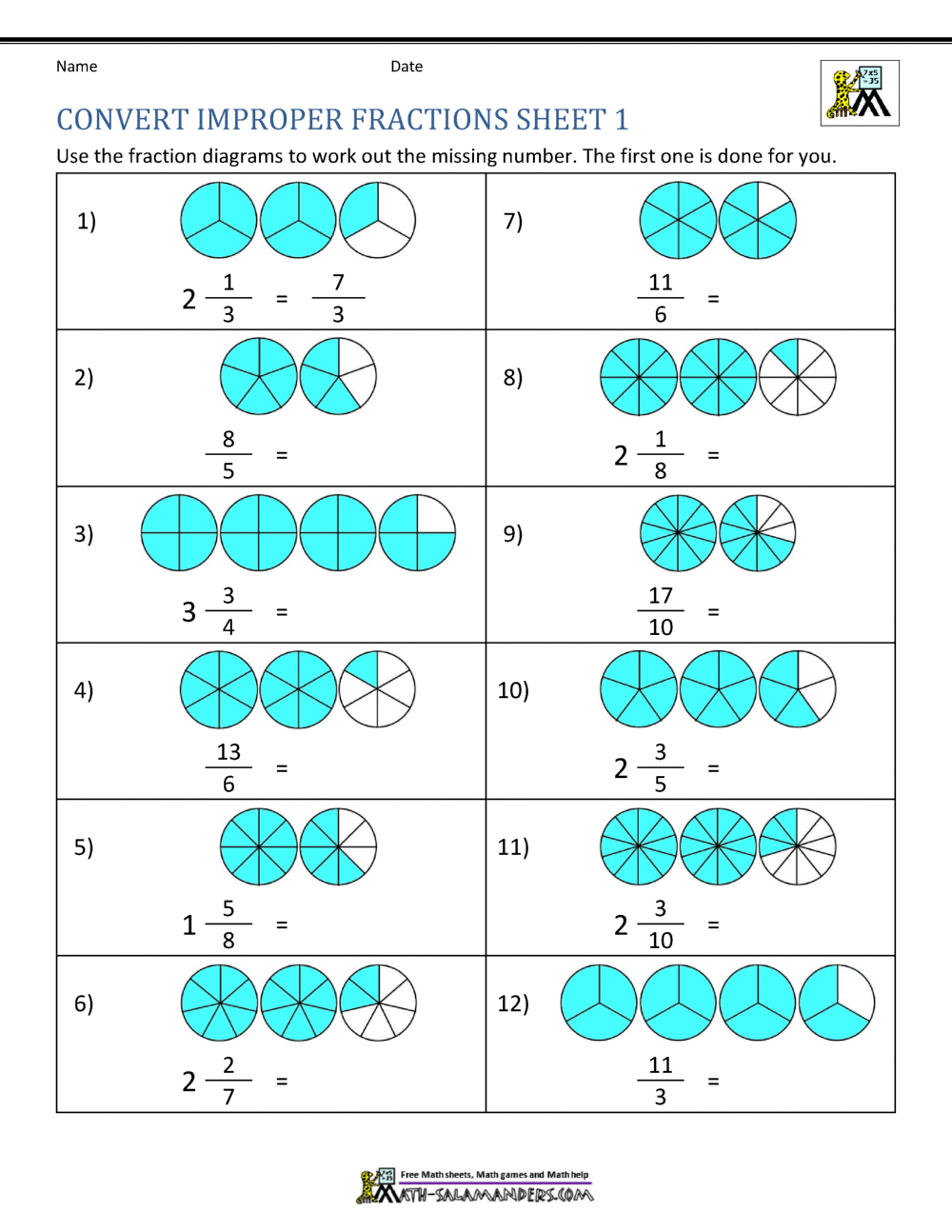
| Mixed Number | Improper Fraction |
|---|---|
| 2 3/4 | 11/4 |
| 5 7/8 | 47/8 |
| 1 1/2 | 3/2 |

📝 Note: In some real-world applications, you might find mixed numbers more intuitive. However, for accuracy in mathematical models or calculations, improper fractions are preferable.
When to Use Mixed Numbers vs. Improper Fractions?

Both forms have their uses:
- Mixed Numbers: Useful when measuring physical items or expressing quantities in a form that mimics real-world scenarios.
- Improper Fractions: Preferrable for mathematical operations, reducing fractions, and when precision in computation is essential.
In summary, mastering the conversion from mixed numbers to improper fractions expands your mathematical toolkit, allowing for more versatile problem-solving and precision in various fields. Whether you're a student, an engineer, or just someone interested in enhancing your numerical literacy, this skill will prove invaluable in interpreting and manipulating numbers effectively.
What are mixed numbers and improper fractions?

+
Mixed numbers combine a whole number with a fraction, while improper fractions have the numerator greater than or equal to the denominator.
How do I know when to use mixed numbers or improper fractions?

+
Mixed numbers are often intuitive for everyday use, particularly when dealing with tangible objects. Conversely, improper fractions are more efficient for mathematical operations and when precision is necessary.
Can all mixed numbers be converted to improper fractions?

+
Yes, every mixed number can be transformed into an improper fraction following the steps outlined in this article.