Converse Inverse Contrapositive Made Easy With This Worksheet

Understanding Converse, Inverse, and Contrapositive Statements

When it comes to logical reasoning and mathematical proofs, understanding the concepts of converse, inverse, and contrapositive statements is crucial. These concepts are often confusing, even for experienced mathematicians and logicians. In this article, we will delve into the world of converse, inverse, and contrapositive statements, and provide a worksheet to help you practice and reinforce your understanding.
What are Converse, Inverse, and Contrapositive Statements?

To begin with, let’s define each of these terms:
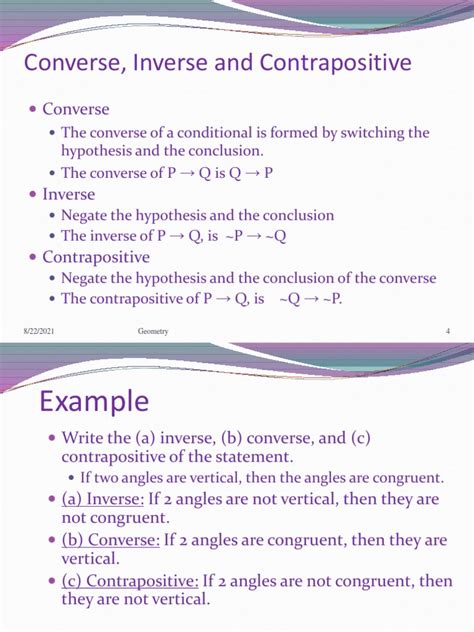
- Converse: The converse of a statement is formed by switching the hypothesis and conclusion. For example, if we have the statement “If it is raining, then the streets are wet,” the converse would be “If the streets are wet, then it is raining.”
- Inverse: The inverse of a statement is formed by negating both the hypothesis and conclusion. For example, if we have the statement “If it is raining, then the streets are wet,” the inverse would be “If it is not raining, then the streets are not wet.”
- Contrapositive: The contrapositive of a statement is formed by negating both the hypothesis and conclusion, and then switching them. For example, if we have the statement “If it is raining, then the streets are wet,” the contrapositive would be “If the streets are not wet, then it is not raining.”
How to Identify Converse, Inverse, and Contrapositive Statements

Identifying converse, inverse, and contrapositive statements can be tricky, but with practice, you’ll become more comfortable. Here are some tips to help you:
- Converse: Look for statements that start with “If” and have a clear hypothesis and conclusion. Switch the hypothesis and conclusion to form the converse.
- Inverse: Look for statements that start with “If” and have a clear hypothesis and conclusion. Negate both the hypothesis and conclusion to form the inverse.
- Contrapositive: Look for statements that start with “If” and have a clear hypothesis and conclusion. Negate both the hypothesis and conclusion, and then switch them to form the contrapositive.
Worksheet: Converse, Inverse, and Contrapositive Statements

Here’s a worksheet to help you practice identifying converse, inverse, and contrapositive statements:
| Original Statement | Converse | Inverse | Contrapositive |
|---|---|---|---|
| If it is raining, then the streets are wet. | If the streets are wet, then it is raining. | If it is not raining, then the streets are not wet. | If the streets are not wet, then it is not raining. |
| If a number is even, then it is divisible by 2. | If a number is divisible by 2, then it is even. | If a number is not even, then it is not divisible by 2. | If a number is not divisible by 2, then it is not even. |
| If a person is over 18, then they are eligible to vote. | If a person is eligible to vote, then they are over 18. | If a person is not over 18, then they are not eligible to vote. | If a person is not eligible to vote, then they are not over 18. |

📝 Note: Make sure to read each statement carefully and identify the hypothesis and conclusion before forming the converse, inverse, and contrapositive statements.
Conclusion

Understanding converse, inverse, and contrapositive statements is an essential skill for logical reasoning and mathematical proofs. With practice, you’ll become more comfortable identifying these statements and using them to solve problems. Remember to always read each statement carefully and identify the hypothesis and conclusion before forming the converse, inverse, and contrapositive statements.
What is the difference between converse, inverse, and contrapositive statements?

+
The converse of a statement is formed by switching the hypothesis and conclusion. The inverse of a statement is formed by negating both the hypothesis and conclusion. The contrapositive of a statement is formed by negating both the hypothesis and conclusion, and then switching them.
Why are converse, inverse, and contrapositive statements important in logical reasoning?
+
Converse, inverse, and contrapositive statements are important in logical reasoning because they help us to analyze and evaluate arguments. By identifying these statements, we can determine the validity of an argument and make logical conclusions.
How can I practice identifying converse, inverse, and contrapositive statements?

+
You can practice identifying converse, inverse, and contrapositive statements by working through worksheets and exercises. Try to identify the hypothesis and conclusion of each statement, and then form the converse, inverse, and contrapositive statements.
Related Terms:
- converse inverse contrapositive formula
- converse vs inverse chart
- converse inverse contrapositive truth table
- inverse converse contrapositive worksheet pdf
- converse vs inverse truth table
- inverse converse and contrapositive examples