7 Ways to Master Constant of Proportionality in 7th Grade

Learning about the constant of proportionality in 7th grade is an essential stepping stone in the realm of mathematics. It not only helps students understand direct relationships between quantities but also lays a foundation for tackling more complex algebraic concepts later on. Here are seven proven strategies to master this critical math concept:
The Concept of Proportionality

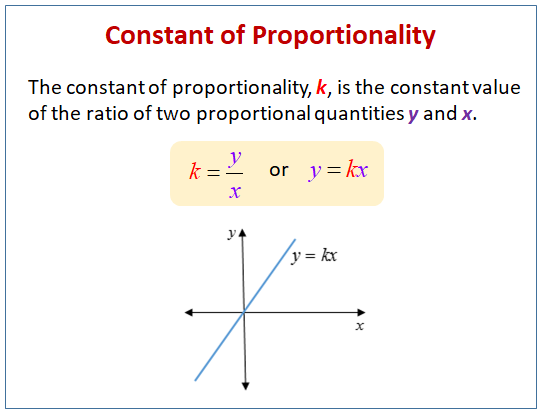
Before delving into the methods, it’s crucial to comprehend what the constant of proportionality is. Simply put, it's the constant value (denoted often as k) that connects two variables in a directly proportional relationship where one variable is always a multiple of the other. If y varies directly with x, we express this relationship mathematically as:
- y = kx
Here, k is the constant of proportionality. Understanding this basic formula is pivotal for mastering related concepts.
1. Use Real-Life Examples

Relating math to real-life scenarios helps students visualize and grasp abstract concepts better:
- Speed and Distance: If a car travels at a constant speed, the distance covered is proportional to the time spent traveling (d = kt).
- Cooking Recipes: Cooking ingredients can be a great way to teach proportionality. For example, doubling or halving a recipe shows that the quantity of each ingredient changes proportionally.
🔍 Note: Encouraging students to find their own examples can foster creativity and a deeper understanding of the concept.
2. Proportional Relationships Through Graphing


Graphing provides a visual interpretation of proportionality:
- Plotting Points: Create a table of values for x and y and plot these points on a graph. If the line is straight and passes through the origin, the relationship is directly proportional.
- Line Equation: The slope of this line is the constant of proportionality. From the graph, students can determine k by looking at the slope or the rise over the run.
3. Manipulate Algebraic Equations

Teaching students how to manipulate equations is crucial:
- Given an equation like 5y = 10x, rearrange to find y = 2x, where k = 2.
- Students should practice solving for k in various equations to solidify their understanding.
4. Interactive Learning Activities

Interactive activities can engage students and make learning fun:
| Activity | Description |
|---|---|
| Proportionality Card Game | Use cards where students match quantities to their proportional relationships. |
| Proportional Walk | Students physically measure distances and times to determine the constant of proportionality. |

5. Mastering Word Problems

Word problems require students to translate everyday scenarios into mathematical models:
- Reading through the problem to identify variables, constants, and the proportional relationship.
- Setting up the equation and solving for k, then using it to solve other parts of the problem.
💡 Note: Encourage students to write down what each variable represents to avoid confusion in word problems.
6. Group Study Sessions

Group work fosters a collaborative learning environment:
- Peer teaching: Students explaining concepts to one another can reinforce their understanding.
- Problem-solving sessions: Groups can work through complex problems together, discussing strategies and solutions.
7. Assessment and Feedback

Regular assessment is key to gauge understanding and provide feedback:
- Short quizzes or homework assignments can help identify areas of weakness.
- Constructive feedback from teachers and peers helps to correct misconceptions and deepen knowledge.
Having covered these methods, students will not only master the constant of proportionality but also develop a strong foundation in mathematical reasoning. With a deeper understanding of how quantities relate, they'll be equipped to handle more abstract mathematical problems in the future, thereby enhancing their mathematical prowess.
How do I know if two quantities are directly proportional?
+
If the ratio of the two quantities is constant, they are directly proportional. Mathematically, y = kx where k is a constant.
Can the constant of proportionality be zero?
+
No, if k = 0, then y would always be zero, which means there is no change in y with respect to x, indicating no relationship.
What if I have non-linear data; can I still use proportionality?

+
For non-linear data, the relationship might be inverse or quadratic, requiring different approaches than direct proportionality.
Related Terms:
- Teacher synergy llc
- Khan Academy
- IXL Learning
- BrainPop
- Udacity
- Duolingo