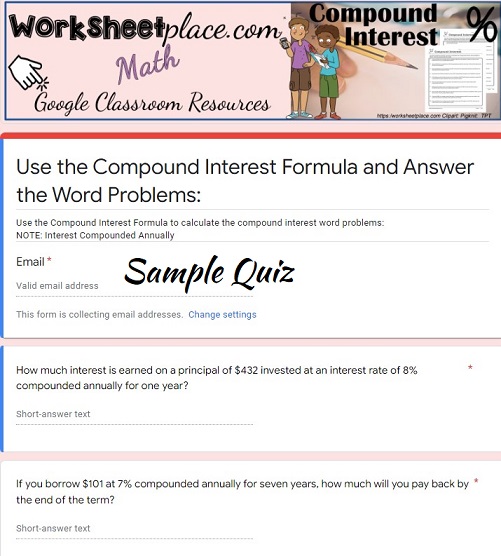
Compound Interest Worksheet Answers Explained: A Key Guide

The concept of compound interest is fundamental in finance and investment, often considered one of the most powerful tools for wealth accumulation. This long-form guide will delve deep into how compound interest works, how to answer common worksheet questions, and the key principles involved. Whether you're a student trying to understand compound interest for your homework or an investor looking to harness its power, this detailed explanation aims to be your definitive resource.
Understanding Compound Interest


Compound interest is interest on interest, which means you earn interest not only on your initial investment but also on the accumulated interest from previous periods. This can significantly increase the growth rate of your investments over time compared to simple interest.
The Compound Interest Formula

The formula for compound interest is:
A = P(1 + r/n)^(nt)
- A - The future value of investment/loan, including interest
- P - Principal investment amount (the initial deposit or loan amount)
- r - Annual interest rate (decimal)
- n - Number of times interest is compounded per year
- t - Number of years the money is invested or borrowed for
💡 Note: Remember, the more frequently interest is compounded, the higher the amount you will earn over time.
Compound Interest Worksheet Examples

Example 1: Basic Compound Interest Calculation

Question: Calculate the future value of a 10,000 investment with a 6% annual interest rate compounded quarterly for 5 years.</p> <ul> <li>Given: <ul> <li>Principal (P) = 10,000
- A = 10000 * (1 + 0.06/4)^(4*5) = $13,468.55
Your investment would grow to approximately $13,468.55 over 5 years.
Example 2: Impact of Compounding Frequency

Question: Compare the final amount of 5,000 invested for 10 years at an 8% interest rate when compounded annually versus semi-annually.</p> <table> <tr> <th>Compounding Frequency</th> <th>Final Amount</th> </tr> <tr> <td>Annually</td> <td>10,794.62 Semi-annually $11,025.00
Example 3: Effective Annual Rate (EAR)

Question: What is the effective annual rate (EAR) for an 8% nominal rate compounded monthly?
- Given: Nominal Rate = 8% or 0.08, Compounding Frequency = 12
- Formula: EAR = (1 + (r/n))^n - 1
- EAR Calculation: EAR = (1 + 0.08/12)^12 - 1 = 0.0830 or 8.30%
Even though the nominal rate is 8%, due to monthly compounding, the effective rate is higher at 8.30%.
Additional Considerations for Compound Interest

Rule of 72

The Rule of 72 is a simple way to estimate how long it will take for your investment to double with a fixed annual rate of interest. By dividing 72 by the annual rate of return, you get an approximate number of years.
The Effect of Withdrawals

If you withdraw from your investment, you reduce the principal, which impacts the future compounding. This principle underscores the importance of regular contributions to keep your compound interest working at its full potential.
💡 Note: Compound interest favors long-term investments, so patience and consistency can greatly enhance your financial outcomes.
Wrapping Up

The magic of compound interest lies in its ability to transform modest investments into significant sums over time. Understanding the principles, formulas, and real-world applications of compound interest through worksheets and practical examples can help demystify finance for everyone. Remember, the key is not just understanding the math but applying the knowledge to make informed financial decisions, choosing investments that maximize compounding benefits, and being aware of how different rates and compounding frequencies impact your returns. Now, let’s tackle some of the common questions you might have about compound interest.
What is the difference between simple and compound interest?

+
Simple interest is calculated only on the principal amount, whereas compound interest is calculated on the initial principal and also on the accumulated interest of previous periods. Over time, this results in exponential growth of money with compound interest.
How does the frequency of compounding affect my investment?

+
More frequent compounding leads to a higher yield because interest gets calculated and added back to the principal more often, allowing for interest on the interest to compound more quickly.
Can compound interest work against me?
+
Yes, if you’re on the borrowing side. Debt like credit card balances or loans with compound interest can grow rapidly if not paid off, leading to potentially overwhelming debts.
How does inflation affect compound interest?

+
Inflation reduces the purchasing power of money. If your investment’s compound interest rate is lower than the inflation rate, your money will effectively lose value over time.
What investment vehicles are best for utilizing compound interest?
+Long-term investments like retirement accounts (e.g., 401(k)s, IRAs), bonds, or dividend reinvestment plans (DRIPs) in stocks can benefit from compound interest, especially those that allow for automatic reinvestment of earnings.