Simplify Math: 8th Grade Combining Like Terms Worksheet

Mastering the concept of combining like terms is a crucial step in a student's mathematical journey. This skill not only simplifies expressions but also prepares students for more complex algebraic operations in future courses. Whether you're a student tackling 8th-grade math, a teacher, or a parent looking to support your child, this comprehensive guide will provide you with worksheets, strategies, and tips to understand and apply combining like terms effectively.
What Are Like Terms?

Before diving into combining like terms, understanding what they are is fundamental. Like terms are terms that have the same variable raised to the same power. Here are key points:
- They share the same variable(s).
- Exponents on the variables must be the same.
- Coefficients (numbers in front of the variables) can be different.
For instance, in the expression 5x^2 + 2x + 3x^2 , 5x^2 and 3x^2 are like terms because they have the same variable x raised to the second power. On the other hand, 2x is not a like term in this context since its exponent differs from x^2 .
How to Combine Like Terms?

Combining like terms involves adding or subtracting their coefficients while keeping the variable and exponent unchanged. Here's how:
- Identify like terms within the expression.
- Add or subtract the coefficients.
- Combine the result with the variable part.
Let's simplify the earlier example:
- 5x^2 + 3x^2 = (5 + 3) x^2 = 8x^2 .
- The term 2x remains as is because it has no like terms.
The simplified expression then becomes 8x^2 + 2x .
📝 Note: Always make sure to check if terms can be combined before simplifying. Don't overlook any like terms!
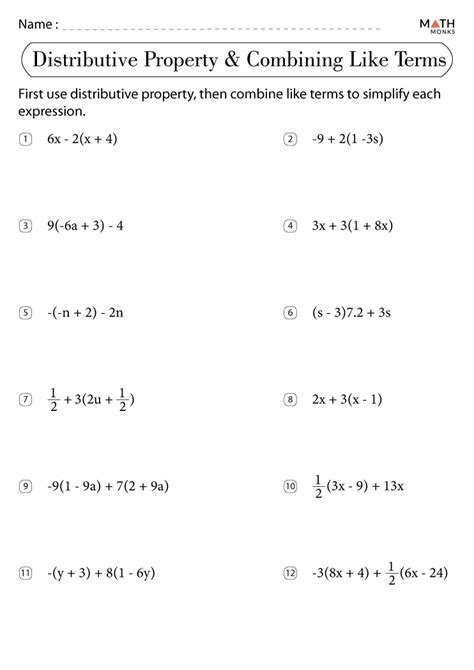
Practical Worksheets for Combining Like Terms

Now let's move to practical exercises to solidify this concept. Here's a table summarizing different types of worksheets you might come across:
| Worksheet Type | Description | Difficulty |
|---|---|---|
| Basic | Includes simple expressions with like terms and no negative numbers. | ★☆☆☆☆ |
| Intermediate | Introduces negative coefficients and constants along with variables. | ★★☆☆☆ |
| Advanced | Combines like terms in multi-step expressions, potentially with distributive property. | ★★★☆☆ |
| Challenging | Complex algebraic expressions requiring careful term identification and multi-step combining. | ★★★★☆ |

Here are some examples of combining like terms from basic to challenging:
Basic Level
Problem: Simplify ( 4x + 3x ).
Solution:
- ( 4x ) + ( 3x ) = (4 + 3)( x ) = ( 7x ).
Intermediate Level

Problem: Simplify ( 2y^2 - y + 5y^2 + 3 ).
Solution:
- Combine like terms ( 2y^2 ) and ( 5y^2 ) to get ( 7y^2 ).
- Combine the constants (-y + 3 ) which stays as (-y + 3 ) since there are no other like terms.
- The result is ( 7y^2 - y + 3 ).
Advanced Level

Problem: Simplify ( 3a - 5b + 6a - 2b + a ).
Solution:
- Combine ( 3a ), ( 6a ), and ( a ) to get ( 10a ).
- Combine (-5b) and (-2b ) to get (-7b ).
- The simplified expression is ( 10a - 7b ).
Challenging Level

Problem: Simplify ( 9 + 3x + 2(4 - 2x) + 7 ).
Solution:
- Distribute the 2 through the parentheses: ( 9 + 3x + 8 - 4x + 7 ).
- Combine like terms: (-x + 24 ).
By progressing through these worksheets, students can build confidence and skill in combining like terms. Make sure to check the work by reversing the process to ensure correctness.
🔍 Note: It’s beneficial to practice combining like terms in both horizontal and vertical formats to improve understanding and flexibility in solving.
Strategies for Teaching and Learning

Here are some effective strategies to teach and learn how to combine like terms:
- Color-Coding: Use different colors to highlight like terms within expressions, making it easier for students to visualize.
- Interactive Learning: Incorporate games and interactive activities where students match like terms or sort terms by variable and exponent.
- Mnemonic Devices: Teach students mnemonic devices like "SSDD" (Same Sign, Distribute and Divide) to remember the process.
- Conceptual Understanding: Explain why like terms can be combined, reinforcing the idea that algebra is essentially about organizing and simplifying mathematical statements.
Summing Up Key Points

Combining like terms is foundational in algebra, allowing us to simplify expressions effectively. It involves:
- Identifying like terms by their variable and exponent.
- Adding or subtracting coefficients.
- Practicing through varied worksheets.
- Using teaching strategies like color-coding and interactive learning to enhance understanding.
Remember, mastery of this concept not only streamlines algebraic work but also builds a deeper comprehension of mathematics, paving the way for more advanced algebraic concepts.
What are examples of like terms?

+
Examples of like terms include: ( 3x ) and ( 5x ), ( 4y^2 ) and ( -2y^2 ), or ( 7 ) and ( 9 ).
Can terms with different variables be combined?

+
No, only terms with identical variables and exponents can be combined.
How can I check if my like term combining is correct?

+
You can reverse the process to verify your work or plug simplified expressions back into equations to ensure they work.