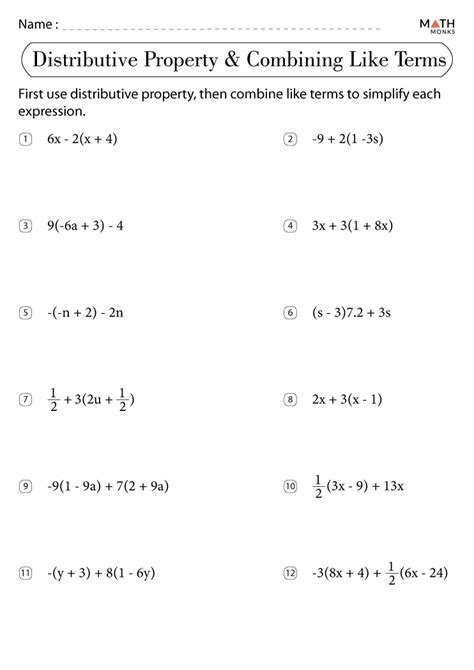
Distributive Property Worksheet
Introduction to the Distributive Property

The distributive property is a fundamental concept in mathematics that helps us simplify expressions and solve equations. It states that for any numbers a, b, and c, the following equation holds: a(b + c) = ab + ac. This property allows us to distribute the multiplication over addition, making it easier to work with complex expressions. In this article, we will explore the distributive property in detail, provide examples, and offer a worksheet to practice applying this concept.
Understanding the Distributive Property

To understand the distributive property, let’s consider a simple example. Suppose we have the expression 2(3 + 4). Using the distributive property, we can rewrite this expression as 2*3 + 2*4, which simplifies to 6 + 8 = 14. This demonstrates how the distributive property helps us simplify expressions by distributing the multiplication over addition.
Applying the Distributive Property

The distributive property can be applied in various mathematical operations, including addition, subtraction, multiplication, and division. Here are some examples: * 3(2 + 5) = 3*2 + 3*5 = 6 + 15 = 21 * 4(10 - 3) = 4*10 - 4*3 = 40 - 12 = 28 * 2(7 + 2) = 2*7 + 2*2 = 14 + 4 = 18
As you can see, the distributive property makes it easier to simplify complex expressions and solve equations.
Distributive Property Worksheet

Now that we have explored the distributive property, it’s time to practice applying this concept. Here is a worksheet with 10 questions to help you reinforce your understanding:
| Question | Expression |
|---|---|
| 1 | 2(5 + 3) |
| 2 | 4(2 + 1) |
| 3 | 3(7 - 2) |
| 4 | 5(9 + 1) |
| 5 | 2(11 - 4) |
| 6 | 6(3 + 2) |
| 7 | 8(5 - 1) |
| 8 | 9(2 + 6) |
| 9 | 7(1 + 3) |
| 10 | 1(9 + 8) |

To solve these questions, simply apply the distributive property to each expression.
📝 Note: Take your time to work through each question, and make sure to simplify each expression using the distributive property.
Real-World Applications of the Distributive Property

The distributive property has numerous real-world applications, including: * Cost calculation: When calculating the total cost of items, the distributive property can be used to simplify expressions and find the total cost. * Measurement conversion: The distributive property can be used to convert between different units of measurement, such as converting between inches and feet. * Science and engineering: The distributive property is used in various scientific and engineering applications, including calculating forces, velocities, and accelerations.
Key Takeaways

In summary, the distributive property is a powerful tool in mathematics that helps us simplify expressions and solve equations. By applying this property, we can make complex expressions more manageable and easier to work with. Remember to practice applying the distributive property using the worksheet provided, and explore its real-world applications in various fields.
Now, let’s summarize the key points and reflect on what we have learned. The distributive property is an essential concept in mathematics, and understanding its application can help us solve complex problems with ease. By practicing and reinforcing our understanding of this concept, we can become more proficient in mathematics and develop a stronger foundation for future learning.
What is the distributive property?

+
The distributive property is a mathematical concept that states that for any numbers a, b, and c, the following equation holds: a(b + c) = ab + ac.
How do I apply the distributive property?

+
To apply the distributive property, simply multiply the number outside the parentheses by each number inside the parentheses, and then add or subtract the results.
What are some real-world applications of the distributive property?

+
The distributive property has numerous real-world applications, including cost calculation, measurement conversion, and science and engineering.



