Circle Geometry Mastery: 5 Essential Answers You Need
Circle geometry, often referred to as the study of geometric figures and their properties, offers a fascinating insight into the fundamental concepts of mathematics. It's not just about learning formulas; it's about understanding the intrinsic nature of shapes, the harmony within their dimensions, and how these principles apply in real-world scenarios. Here, we'll dive into 5 essential questions to unlock the depth of circle geometry, ensuring that every reader can grasp the beauty and utility of this branch of mathematics.
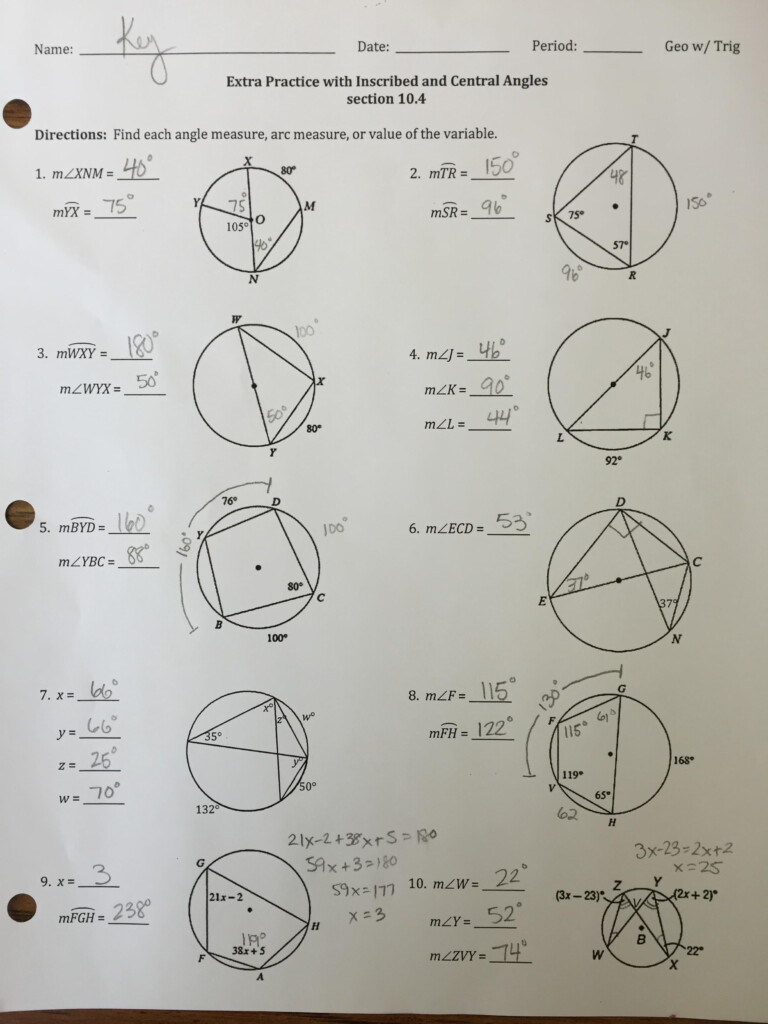
Central Angles, Inscribed Angles, and their Practical Applications

Central and inscribed angles are pivotal in circle geometry, offering practical applications beyond the classroom. Here’s how they play a role:
- Central Angle: A central angle is an angle whose vertex is at the center of the circle, and its sides are radii of the circle. Its measure equals the arc it intercepts.
- Inscribed Angle: An inscribed angle has its vertex on the circumference, and its sides intersect the circle again. The angle measure is half of the central angle intercepting the same arc.
Here's a quick demonstration:
| Concept | Formula | Example |
|---|---|---|
| Central Angle | Angle Measure = Arc Measure | A central angle intercepting a 60-degree arc |
| Inscribed Angle | Angle Measure = ½ Arc Measure | An inscribed angle intercepting the same arc would measure 30 degrees |

🔍 Note: Remember, the arc measure refers to the degree measure of the arc, which can be used to solve for the arc length if you know the circle's circumference.
Thales’ Theorem and its Real-World Implications

Named after the ancient Greek philosopher Thales, this theorem illustrates that any angle inscribed in a semicircle is always a right angle. Here’s how it works:
- The key is recognizing that the diameter acts as the hypotenuse of a right triangle.
- This theorem has real-world applications in architecture, particularly in the design of arches or domes.
Chords, Tangents, and Their Intersection with Circles

The relationship between chords, tangents, and circles is intricate:
- Chords: Two equal chords in a circle are equidistant from the center.
- Tangents: A tangent touches the circle at exactly one point, forming a right angle with the radius at that point of tangency.
Intersection Properties:
- Two tangents drawn from a point outside the circle are equal.
- A tangent and a chord intersect at a point, the power of the point formula applies.
Cyclic Quadrilaterals and their Hidden Properties

A cyclic quadrilateral is one where all four vertices lie on a single circle. Here are some key features:
- Opposite angles sum to 180 degrees.
- Diagonals are perpendicular if the quadrilateral is isosceles.
- This concept finds utility in engineering and art, such as in the design of perspective in paintings.
Arcs, Sectors, and Segments: The Building Blocks of Circle Geometry

Understanding these components is essential:
- Arc: The portion of the circumference between two points.
- Sector: The region bounded by two radii and an arc.
- Segment: The area enclosed by a chord and the arc subtended by the chord.
In summarizing these 5 essential aspects, we’ve unveiled the intricate beauty and practical applicability of circle geometry. From central angles to inscribed figures, these elements are not just abstract concepts but have real-world implications in engineering, architecture, and beyond. By mastering these fundamental questions, we open doors to further explorations in mathematics and its applications, proving that geometry is not just about shapes but about understanding the harmony in the universe.
Why are inscribed angles important in construction?

+
Inscribed angles are crucial because they can create right angles, which are fundamental in constructing perpendicular lines, ensuring stability and accuracy in building structures.
Can I use Thales’ theorem to check if a quadrilateral is cyclic?

+
Absolutely! If a quadrilateral has an angle that can be inscribed in a semicircle (90 degrees), it’s a dead giveaway that the quadrilateral is cyclic.
How do tangents relate to tangents in real-world applications?

+
Tangents provide a model for how light and other entities interact with surfaces. For instance, in optics, tangents to the lens help in designing lenses to bend light to a specific point.