5 Easy Steps to Convert Mixed Numbers to Fractions

In the world of mathematics, converting mixed numbers into improper fractions is a foundational skill that's often used in problem-solving, simplifying equations, and understanding quantities. If you've ever wondered how to convert a mixed number to a fraction, you're in the right place. Here, we'll explore the step-by-step process in a straightforward manner, ensuring you can master this technique with ease.

Understanding Mixed Numbers and Improper Fractions

Before diving into the conversion process, let's understand what we're working with:

• Mixed Numbers: These are numbers that consist of a whole number combined with a fraction, like 3 ¼ or 5 ⅗. They represent quantities greater than one.
• Improper Fractions: Fractions where the numerator (top number) is larger than or equal to the denominator (bottom number), such as 7/2 or 9/4. These can represent the same quantity as mixed numbers but in a different format.

Step 1: Identify the Parts of the Mixed Number

Let's take the mixed number 2 3/4 as an example. Here’s how you can break it down:

• The whole number part: 2
• The numerator of the fractional part: 3
• The denominator of the fractional part: 4

Step 2: Multiply the Whole Number by the Denominator

Multiply the whole number (2 in this case) by the denominator (4):

2 * 4 = 8

Step 3: Add This Product to the Numerator

Add the result from Step 2 (8) to the original numerator (3):

8 + 3 = 11

Step 4: Place the Result over the Denominator

Now, place this new numerator (11) over the original denominator (4):

11/4

This is your improper fraction equivalent of the mixed number 2 3/4.

Step 5: Simplify if Necessary

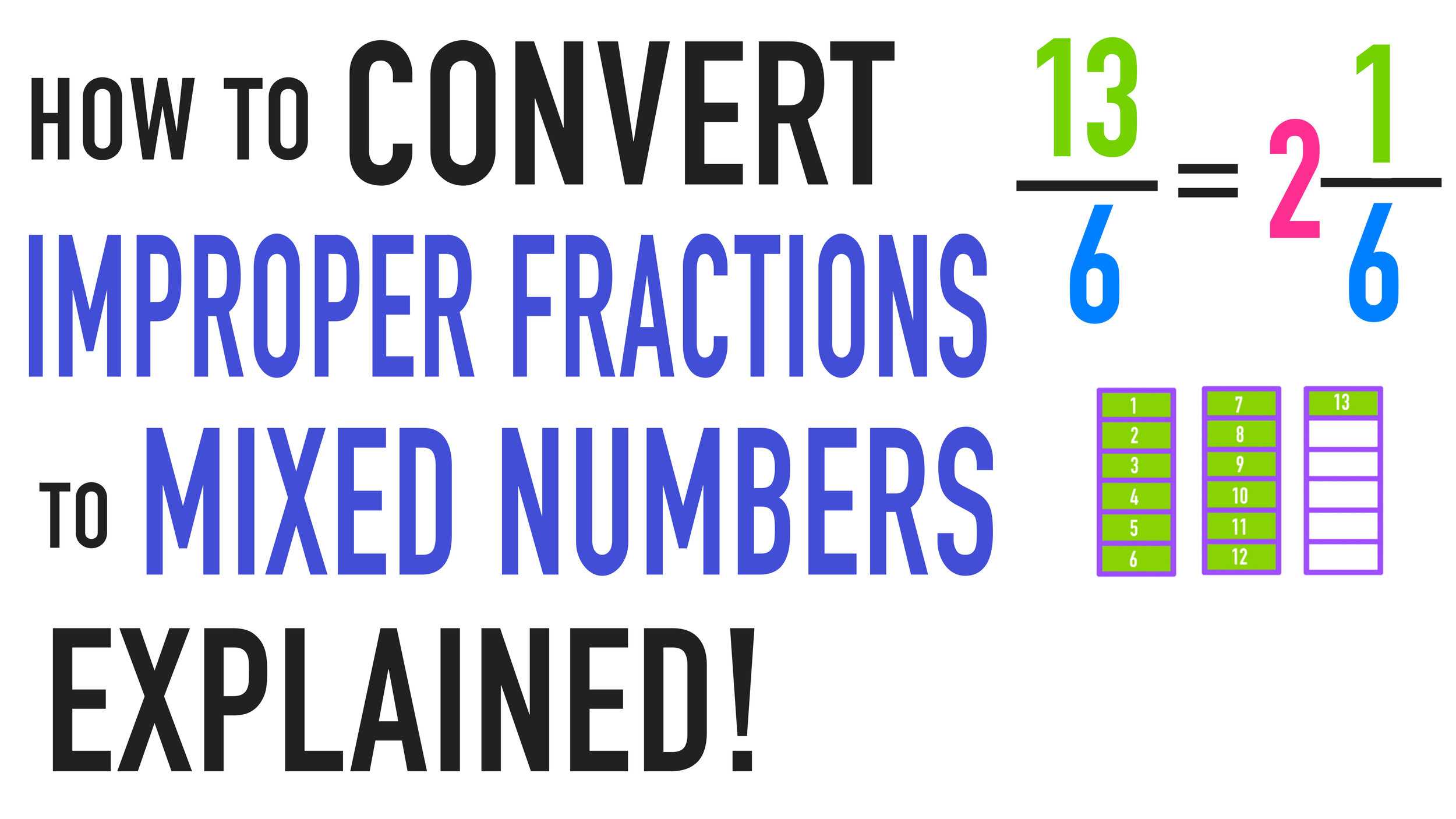
Sometimes, you'll need to reduce or simplify the resulting improper fraction if it's possible:

• If the numerator and denominator have common factors, divide both by their greatest common divisor (GCD). For example, if we had 15/6, we could divide both numerator and denominator by 3, resulting in 5/2.

In our case, 11 and 4 have no common factors other than 1, so 11/4 is already in its simplest form.

🔔 Note: Keep in mind that not all improper fractions can be simplified, especially when the numbers are prime to each other.

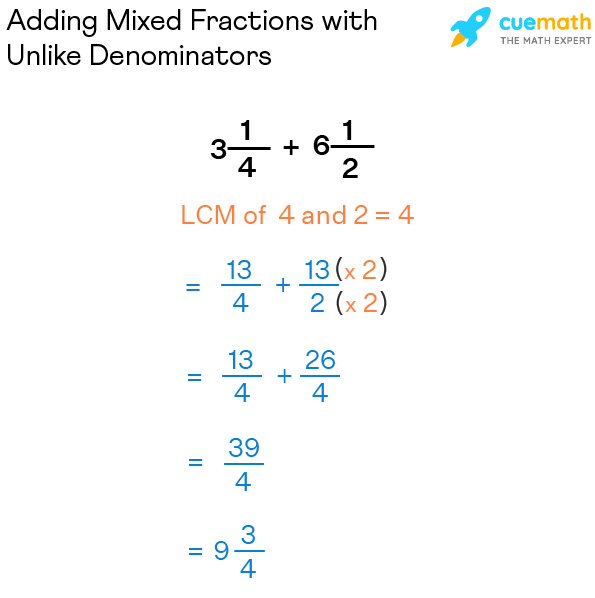
By following these five easy steps, you can convert any mixed number to an improper fraction. This skill is not only useful for basic arithmetic operations but also for higher-level math concepts. Remember, understanding the relationship between mixed numbers and improper fractions can make solving equations and understanding proportions much simpler.

At the core, converting between mixed numbers and improper fractions is about grasping the essence of numbers in different representations. Whether you're dealing with algebra, geometry, or just daily life calculations, having a solid foundation in this skill can streamline your mathematical processes.

Why should I learn to convert mixed numbers to fractions?

+

Converting mixed numbers to fractions simplifies complex operations like addition, subtraction, multiplication, and division. It’s essential in algebra, geometry, and even in practical applications like cooking, where precise measurements are crucial.

Is there an easy way to memorize the conversion steps?

+

Yes, you can use the acronym “Multiply, Add, Denominator” (M.A.D) to remember the steps: Multiply the whole number by the denominator, Add this to the numerator, then write it over the Denominator.

Can I convert an improper fraction back into a mixed number?

+

Absolutely. To convert an improper fraction like ¹¹⁄₄ back to a mixed number, divide the numerator by the denominator (11 ÷ 4 = 2 R3), where the quotient becomes the whole number, and the remainder becomes the numerator over the denominator (2 ³⁄₄).

When is it necessary to convert fractions to mixed numbers?

+

Converting back to mixed numbers can be useful when you need to communicate the fraction as a “quantity” more easily understood by others. For instance, saying “I bought 3 ¹⁄₂ pounds of apples” rather than “I bought ⁷⁄₂ pounds of apples” is more intuitive in everyday scenarios.

What if the improper fraction’s numerator is equal to the denominator?

+

If the numerator equals the denominator, the result is a whole number, not a fraction. For example, ⁴⁄₄ is equal to 1. There’s no need to convert this back to a mixed number as it’s already in its simplest form.