Transform Fractions into Decimals Easily with Our Worksheet

Converting fractions into decimals is a fundamental mathematical skill, crucial for understanding numbers in our daily lives. Whether you're dividing goods for a recipe, calculating sale prices, or analyzing statistics, this skill can be incredibly useful. Here, we will explore how to transform fractions into decimals step-by-step, making this learning process both engaging and practical for learners of all levels.
Understanding the Basics of Fractions

Before we delve into conversion, it's essential to understand what a fraction represents. A fraction is essentially a division of two numbers:
- Numerator: The number above the line, which represents the number of parts you have.
- Denominator: The number below the line, which shows into how many parts the whole is divided.
A fraction like 3/4 means you have 3 parts out of a total of 4 parts, or you're dividing 3 by 4.
The Conversion Process: A Step-by-Step Guide

Step 1: Set Up for Long Division

When converting fractions to decimals:
- Take the numerator and divide it by the denominator. If your fraction is
3/4, set up the division as 3 ÷ 4. - If the result of the division isn't a whole number, you'll need to carry out the division to get a decimal.
Step 2: Perform the Division

Let's take the example of 3/4:
- 3 is less than 4, so we can't divide directly. Add a decimal point to 3, making it 3.0, and place a zero after the decimal. Now we have 3.0 ÷ 4.
- 7 goes into 30
0 times, so we write 0 above the line to start our decimal answer. - 4 goes into 30 seven times, giving us a quotient of 0.75
Therefore, 3/4 expressed as a decimal is 0.75.
Step 3: Handle Non-Terminating Decimals

Not all fractions convert into nice round decimals. Consider the fraction 1/3:
- Set up the division of 1 ÷ 3.
- 1 is less than 3, so we proceed with the decimal: 1.0 ÷ 3.
- We get a repeating pattern, which means
1/3as a decimal is0.333..., commonly written as0.3bar.
Some fractions result in numbers that don't terminate (end) but instead repeat indefinitely. This is an important aspect to understand when dealing with real-life applications of fractions.
⚠️ Note: While some decimals repeat, there are conventions to indicate this repetition, such as writing a bar over the repeating part.
Common Fraction to Decimal Conversions

Here are some common fractions and their decimal equivalents for quick reference:
| Fraction | Decimal |
|---|---|
| 1/2 | 0.5 |
| 1/4 | 0.25 |
| 3/4 | 0.75 |
| 1/5 | 0.2 |
| 1/8 | 0.125 |

🖍️ Note: Familiarizing yourself with these common conversions can save time in calculations.
Using Technology for Conversion

If manual conversion feels overwhelming or if you're looking for accuracy in complex fractions:
- Use a calculator with a division function.
- Apps or online tools that convert fractions to decimals automatically.
Here are a few steps to ensure accuracy when using technology:
- Enter the numerator into the calculator or app first.
- Then input the division symbol or click the division button.
- Enter the denominator and press equals to see the result.
💡 Note: For educational purposes, practicing manual conversion helps in understanding the underlying mathematics.
Wrapping It Up

The journey from fractions to decimals is not just about performing arithmetic; it's about grasping how numbers work together to represent quantities in various forms. We've covered the basic steps, handled repeating decimals, and provided a quick reference for common conversions. Remember, while technology can aid us, understanding the process manually allows for greater appreciation and better problem-solving skills. Whether in school, shopping, or everyday life, this skill will serve you well in understanding and navigating numbers in all their diversity.
What if the decimal doesn’t end?
+
Some fractions result in non-terminating decimals which means they have numbers that repeat indefinitely. For example, 1⁄3 becomes 0.333… (or 0.3bar).
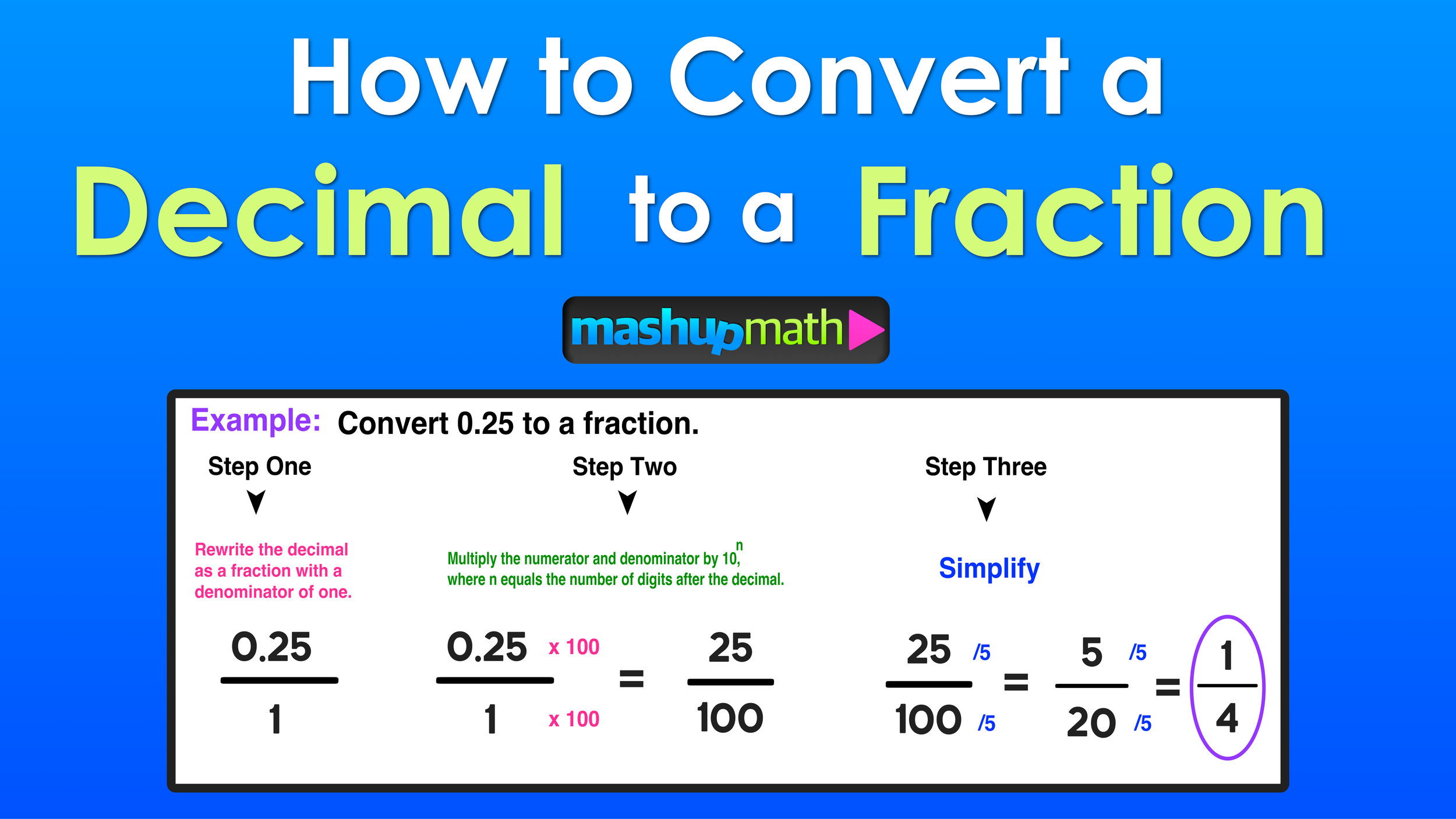
How can I convert a decimal back to a fraction?

+
Take the decimal number and place it over 1, then convert it to its simplest form. For instance, 0.5 is 5⁄10, which simplifies to 1⁄2.
Why is it useful to convert fractions to decimals?

+
Decimals provide a straightforward way to perform calculations, especially when dealing with money, measurements, or statistics. They allow for easier comparison and operation between numbers.