5 Key Points on Triangle Centers for Math Review

The concept of triangle centers is fundamental in mathematics, particularly in the study of geometry. These points, known as centers, serve specific purposes within triangles and provide insights into their properties. Here are five key points on triangle centers you should know for your next math review session.
1. Definition of Triangle Centers

Triangle centers are special points that lie within a triangle, which can be derived from its sides, angles, or vertices. These points are unique in that they exhibit various properties and can be constructed or determined mathematically. Key examples include:
- Circumcenter: The point equidistant from all three vertices of the triangle.
- Centroid: The point where the medians intersect; it is also the average position of all points in the triangle.
- Incenter: The center of the triangle’s incircle, where the angle bisectors meet.
- Orthocenter: The intersection of the triangle’s altitudes.
- Excenters: Points outside the triangle where the external angle bisectors of the three angles meet.
2. Importance of Triangle Centers

Understanding triangle centers is crucial because:
- They help in analyzing and solving geometric problems more efficiently.
- They have applications in various fields including architecture, engineering, and computer graphics.
- They provide a framework for understanding symmetry, equilibrium, and balance within a triangle.
- They are used in the calculation of distances, areas, and other properties of triangles.
3. How to Find Triangle Centers
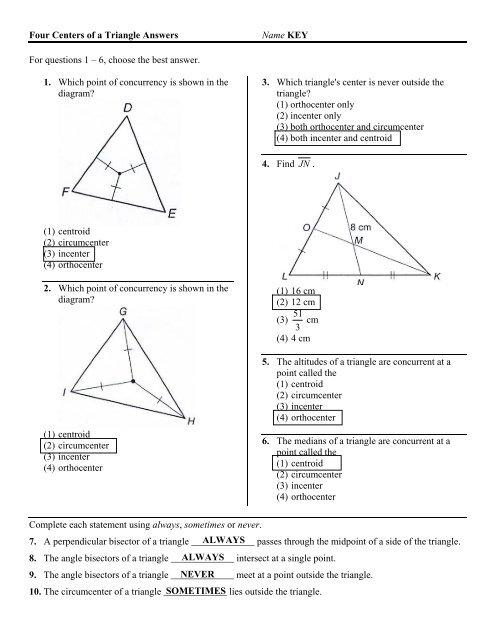
Here are brief explanations on how to find some of the major triangle centers:
- Circumcenter: Find the perpendicular bisectors of any two sides of the triangle. Their intersection is the circumcenter.
- Centroid: Connect the vertices to the midpoints of the opposite sides. These medians will meet at the centroid.
- Incenter: Find the point where the angle bisectors of the triangle intersect.
- Orthocenter: Extend the altitudes of the triangle. The point where they intersect is the orthocenter.
🔍 Note: While the formulas for calculating these centers are useful, visual methods and geometric constructions are often preferred for hands-on learning and problem-solving.
4. Special Properties of Triangle Centers

Each triangle center has unique properties:
- Circumcenter: It is the center of the circle passing through all vertices of the triangle.
- Centroid: It divides each median in a 2:1 ratio, with the centroid being closer to the vertex.
- Incenter: It is equidistant from all sides of the triangle, making it the center of the incircle.
- Orthocenter: The orthocenter has a reflective property where reflected triangles over sides share the same orthocenter.
5. Connections Between Centers

Triangle centers are not isolated points; they are interconnected:
- The Euler line connects the orthocenter, centroid, and circumcenter of any triangle.
- In an equilateral triangle, all these centers coincide.
- The nine-point circle passes through specific points related to the orthocenter, circumcenter, and vertices, connecting them in a unique geometric pattern.
In revisiting these key points, we see that triangle centers not only enhance our understanding of triangles but also connect different aspects of geometry in profound ways. Each center has its own significance and when combined, they reveal a deep, interconnected structure within triangles. Whether you're preparing for a math exam, exploring the beauty of geometry, or applying these principles in practical settings, grasping the concepts of triangle centers will provide a solid foundation for understanding more complex mathematical relationships.
What is the most important triangle center?

+
The most important center depends on the context. However, for most geometric applications, the centroid is often considered central due to its balance point properties.
Can triangle centers lie outside the triangle?

+
Yes, centers like the orthocenter can lie outside the triangle in certain types, such as obtuse triangles.
What is the Euler Line?

+
The Euler Line is a line in any triangle that passes through the orthocenter, centroid, and circumcenter, connecting these key centers in a straight line.
How many triangle centers are there?

+
Theoretically, there are an infinite number of triangle centers, but commonly studied ones are typically in the range of several dozen.
Is the circumcenter always inside the triangle?

+
No, the circumcenter can be outside of an obtuse triangle or at a vertex for a right-angled triangle.