Master Arithmetic and Geometric Sequences with Our Worksheet

Mastering sequences is a fundamental aspect of mathematics, offering a fascinating glimpse into patterns and predictability. In algebra, two types of sequences take center stage: arithmetic and geometric sequences. An understanding of these sequences not only fosters problem-solving abilities but also prepares you for advanced mathematics and real-world applications. Here's a comprehensive guide on how you can master these sequences with the help of our specifically designed worksheet.
Understanding Sequences

Before delving into the specifics of arithmetic and geometric sequences, let’s define what a sequence is. A sequence is an ordered list of numbers that follow a certain pattern. Each number in the sequence is called a term, and the position of the term is called its index.
Arithmetic Sequences

In an arithmetic sequence, each term is calculated by adding or subtracting a fixed number from the previous term. This fixed number is called the common difference.
Defining Arithmetic Sequences

- The first term of the sequence is often denoted as a.
- The common difference is denoted as d.
- The nth term of an arithmetic sequence can be found using the formula:
[ a_n = a + (n-1)d ]
Finding Terms in Arithmetic Sequences

To find specific terms or to generate terms in an arithmetic sequence, follow these steps:
- Identify the first term (a) and the common difference (d).
- Use the formula to find the desired term by substituting n for the position you want.
Sum of an Arithmetic Sequence

The sum of the first n terms of an arithmetic sequence can be calculated by:
[ S_n = \frac{n}{2} (2a + (n-1)d) ]Geometric Sequences

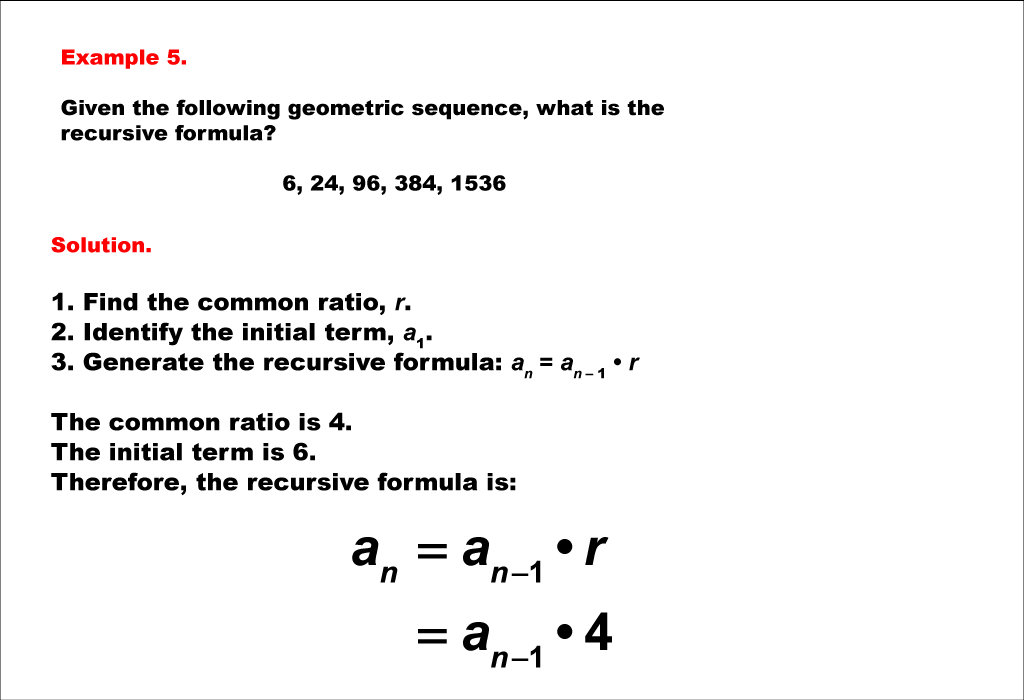
Geometric sequences are defined differently. Each term is found by multiplying the previous term by a fixed number, known as the common ratio.
Defining Geometric Sequences
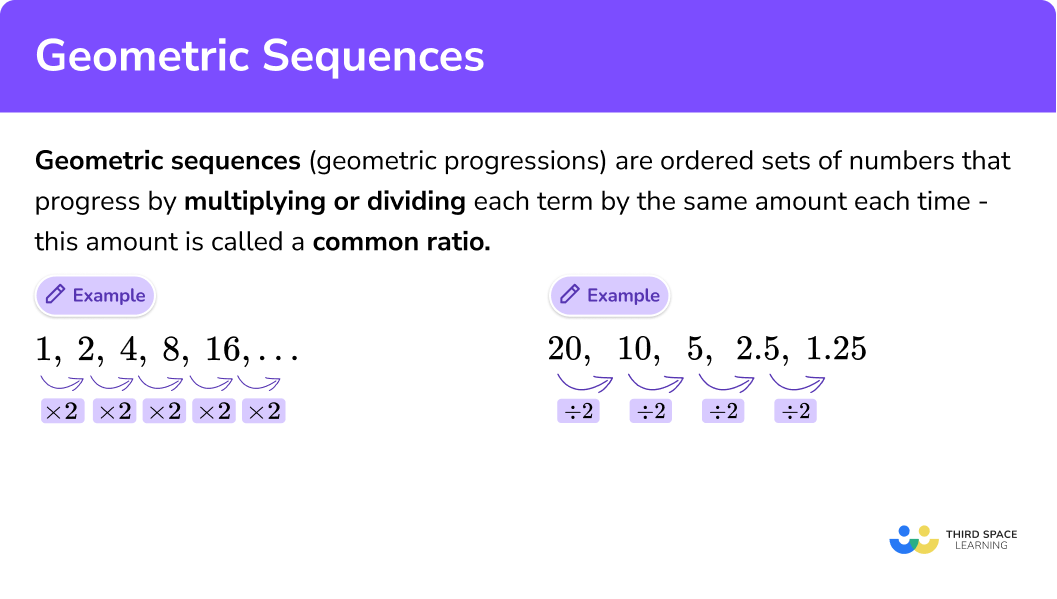
- The first term is denoted as a.
- The common ratio is denoted as r.
- The nth term of a geometric sequence can be found with:
[ a_n = ar^{n-1} ]
Finding Terms in Geometric Sequences

Similar to arithmetic sequences, the process involves:
- Identifying the first term (a) and the common ratio (r).
- Using the formula to find the nth term.
Sum of a Geometric Sequence

The sum of the first n terms of a geometric sequence can be calculated with:
[ S_n = a \frac{1-r^n}{1-r}, \quad \text{for} \quad r \neq 1 ]Using Our Worksheet

Our worksheet is a practical tool to apply these concepts, allowing you to:
- Practice identifying sequences as arithmetic or geometric.
- Calculate the nth term and sum of sequences.
- Work through word problems to understand real-world applications.
💡 Note: Always check the common difference or ratio for consistency in the sequence. Errors in calculation often stem from incorrect identification of these values.
🔍 Note: While our worksheet focuses on the basics, sequences have many advanced applications in calculus, finance, and data analysis.
Throughout your journey of learning arithmetic and geometric sequences with our worksheet, you'll not only master calculating terms and sums but also gain insights into how these sequences operate in the real world. From understanding financial growth models to predicting future events based on historical data, the applications are vast. This knowledge serves as a stepping stone to further mathematical exploration, fostering a deeper appreciation for the patterns that govern our world.
What is the difference between an arithmetic and a geometric sequence?

+
In an arithmetic sequence, each term increases or decreases by a fixed common difference, whereas in a geometric sequence, each term is a product of the previous term and a fixed common ratio. This difference results in linear growth for arithmetic sequences and exponential growth for geometric sequences.
How can I recognize an arithmetic sequence?
+
Identify a consistent difference between consecutive terms. If the difference between any two consecutive terms is constant, you have an arithmetic sequence.
What practical applications do geometric sequences have?

+
Geometric sequences are widely used in finance for compound interest calculations, in population growth modeling, and in digital signals processing where data points form a geometric progression.
Can sequences be used in real life?
+
Yes, sequences appear in numerous real-life scenarios such as predicting stock market trends, calculating loan payments, understanding decay in radioactive materials, and even in the sequencing of genetic information.