Fun Triangle Area Worksheet for Grade 6 Students

Geometry is a fascinating subject that helps students understand the shapes and spaces around them. For grade 6 students, learning about triangles can be both fun and educational. This worksheet focuses on calculating the area of triangles, which is a crucial concept in basic geometry. Whether you're a teacher, parent, or student looking for an engaging way to practice, this guide will walk you through various methods to find the area of different types of triangles.
Understanding Triangle Basics

Before diving into area calculations, let’s refresh our knowledge about triangles:
- Vertices: A triangle has three vertices.
- Sides: A triangle has three sides that connect these vertices.
- Angles: The sum of the angles inside a triangle always equals 180 degrees.
- There are several types of triangles based on sides and angles:
- Equilateral Triangle: All sides and angles are equal.
- Isosceles Triangle: Two sides and two angles are equal.
- Scalene Triangle: All sides and angles are different.
Calculating the Area of a Triangle

The standard formula for finding the area of any triangle is:
Area = (\frac{1}{2} \times \text{base} \times \text{height})
Here are steps to calculate it:
- Identify the base of the triangle. This is usually the side on which the triangle is resting.
- Locate the height (also called the altitude). It’s the perpendicular distance from the base to the opposite vertex.
- Multiply the base by the height.
- Then, multiply the result by (\frac{1}{2}).
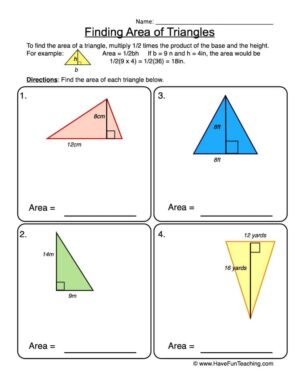
Worksheet Activities

Let’s engage in some practice with these activities:
Activity 1: Using Base and Height

Calculate the area of the following triangles:
| Triangle | Base (in cm) | Height (in cm) |
|---|---|---|
| A | 8 | 5 |
| B | 12 | 7 |
| C | 5 | 10 |

⚡ Note: Remember, the base and height must be measured in the same units for the calculation to work!
Activity 2: Visual Estimation

Look at the triangle below, estimate its area using the given grid:

Activity 3: Real-World Application

If a triangle-shaped playground has a base of 10 meters and a height of 8 meters, how much space does it occupy?
🚩 Note: Triangles are not only found in geometry books; they’re everywhere in real life!
Deepening Understanding

Now that we’ve calculated areas using the basic formula, let’s explore other ways:
Using Heron’s Formula

When you don’t have the height, but you know the lengths of all three sides, use Heron’s Formula:
Area = (\sqrt{s(s-a)(s-b)(s-c)}), where (s) is the semi-perimeter calculated by (\frac{a+b+c}{2}).
Key Points to Remember

- Triangles can be classified based on sides (equilateral, isosceles, scalene) or angles (acute, right, obtuse).
- The formula for area remains constant, but methods of calculation can vary.
- Practical applications include land surveys, architecture, and even in creating video game landscapes.
In wrapping up our exploration of triangle areas, we see how these shapes play a pivotal role in various fields. Understanding the area of triangles provides a foundation not only for geometry but for many real-world applications. Practicing with fun and engaging activities like the ones in this worksheet can help solidify this knowledge, making math both enjoyable and useful.
What if I don’t know the height of a triangle?

+
You can use Heron’s Formula, which requires the lengths of all three sides to calculate the area without knowing the height directly.
Can I calculate the area of an equilateral triangle without knowing the height?

+
Yes, since all sides are equal, you can derive the height using the Pythagorean theorem and then apply the standard area formula, or use Heron’s Formula directly with all sides being equal.
How do real-life triangles differ from geometric triangles?
+
Real-life triangles might not have perfectly straight sides or exact measurements, but the principles of geometry still apply. They help in areas like civil engineering for calculating land area or in design for creating balanced structures.