5 Essential Tips for Polygon Area Worksheet Answers
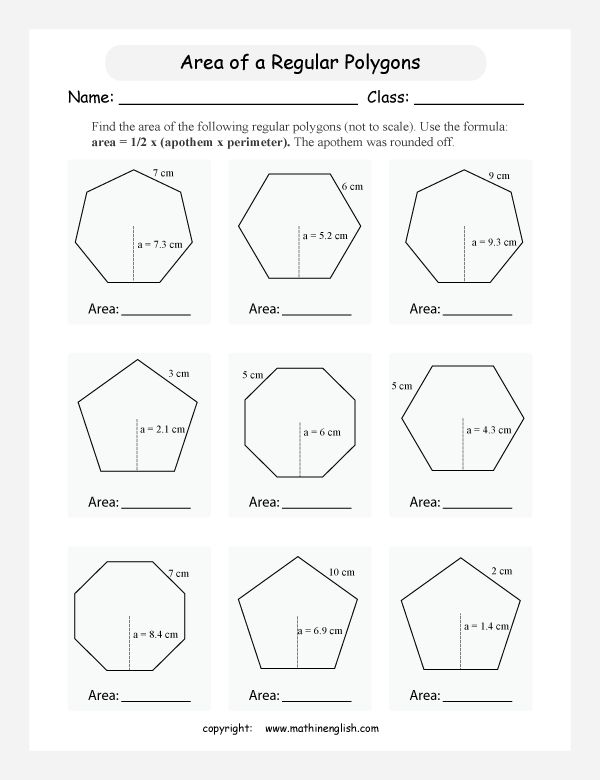
When it comes to understanding geometry and measurements in schools and colleges, polygon area worksheet answers play a crucial role. Here's your go-to guide with essential tips to ace your calculations and understand polygon areas better:
Tip 1: Master the Basic Formulas

To find the area of polygons, having a firm grasp on the basic formulas is essential. Here are the formulas for common polygons:
- Triangle: Area = 1⁄2 * base * height
- Rectangle: Area = Length * Width
- Parallelogram: Area = Base * Height
- Trapezoid: Area = 1⁄2 * (Base1 + Base2) * Height
- Circle: Although not a polygon, understanding its formula helps: Area = π * r^2
💡 Note: While these formulas are straightforward, they form the bedrock for understanding more complex polygons.
Tip 2: Visualize and Identify Shapes

Before diving into calculations, take a moment to visualize the polygon in question. Break down complex shapes into simpler ones if necessary. For instance:
- Divide irregular shapes into triangles, rectangles, or other known polygons.
- If a polygon is not given on a grid, sketch it roughly to identify potential simpler shapes inside.
- Use color or shading to distinguish between different parts of your polygon if you’re drawing it.
Tip 3: Use Coordinate Geometry When Possible

If you’re given the vertices of a polygon on a coordinate plane, you can leverage the power of coordinate geometry:
- Direct Calculation: For a polygon with vertices (x1, y1), (x2, y2), …, (xn, yn), you can use the Shoelace Formula for area calculation.
- Decompose: Split the polygon into triangles or rectangles whose area can be easily calculated using coordinate differences.
| Polygon | Formula |
|---|---|
| Triangle | Area = |((x1*(y2-y3) + x2*(y3-y1)+ x3*(y1-y2))/2)| |
| Quadrilateral | Area = 1/2 |(x1y2 + x2y3 + x3y4 + x4y1) - (y1x2 + y2x3 + y3x4 + y4x1)| |

Tip 4: Pay Attention to Units

The area of a polygon is typically measured in square units, which can change based on the context of the problem:
- If the polygon is on a grid where each side is one unit, area units will be 1x1 = 1 square unit.
- For real-world applications, like calculating areas of fields or rooms, units might be in square feet, meters, or other measurements.
Make sure your units are consistent throughout the problem. If they’re not provided, take a moment to derive what they should be.
📏 Note: Always check the scale of your problem before calculating areas, as units can greatly impact the result.
Tip 5: Check Your Work

After obtaining your answer, a quick recheck can save you from simple mistakes:
- Redo the calculation to ensure the result is consistent.
- Use estimation or another method to verify your answer seems reasonable.
- If possible, physically measure or model the polygon to confirm your calculations.
Double-checking your work is particularly important in math where small errors can lead to significant miscalculations.
After exploring these tips, you’re now equipped to handle polygon area worksheet answers with confidence. Understanding these basic principles allows you to approach any polygon problem methodically and accurately. Whether it’s for homework, exams, or real-world applications, mastering polygon areas is not just about solving equations but also about understanding geometric patterns and spatial relationships.
What if the polygon’s shape isn’t easily divisible?

+
If a polygon’s shape is complex, try using the ‘Pick’s Theorem’ if it’s grid-based, or integrate using calculus for more complex, non-grid shapes.
How do I handle negative coordinates?

+
Negative coordinates are handled similarly to positive ones in calculations. The sign matters only for calculating the y-difference in some formulas, ensuring you get the absolute value for the area.
Can I use software for these calculations?
+
Yes, many geometry software tools like GeoGebra, Desmos, or even online calculators can solve polygon area problems. However, manual calculations ensure you understand the underlying concepts.